Ionization potential of hydrogen atom is 13.6V. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1eV. The spectral lines emitted by hydrogen atoms according to Bohr's theory will be
To solve the problem, we need to determine the spectral lines emitted by hydrogen atoms when they are excited by a photon of energy 12.1 eV. Here’s a step-by-step solution:
Step 1: Understand the Given Information
- Ionization potential of hydrogen atom = 13.6 eV
- Energy of the incoming photon = 12.1 eV
Step 2: Calculate the Energy of the Electron in the Excited State
The energy of the electron in the excited state can be calculated by adding the energy of the incoming photon to the ionization potential of the hydrogen atom.
Eexcited=Eionization+Ephoton=−13.6eV+12.1eV
Calculating this gives:
Eexcited=−13.6+12.1=−1.5eV
Step 3: Relate the Excited State Energy to Quantum Number
According to Bohr's model, the energy of an electron in the nth orbit is given by:
En=−13.6n2eV
We set this equal to the energy we calculated for the excited state:
−1.5=−13.6n2
Step 4: Solve for n
Rearranging the equation gives:
n2=13.61.5
Calculating this:
n2=13.61.5≈9.0667
Taking the square root gives:
n≈3
Step 5: Determine Possible Transitions
The electron is now in the n=3 state. The possible transitions (and thus spectral lines) are:
1. From n=3 to n=2
2. From n=3 to n=1
3. From n=2 to n=1
Step 6: Count the Spectral Lines
The total number of spectral lines emitted corresponds to the possible transitions:
- 3 to 2
- 3 to 1
- 2 to 1
Thus, there are 3 spectral lines emitted.
Final Answer
The spectral lines emitted by hydrogen atoms according to Bohr's theory will be 3.
---
- Ionization potential of hydrogen atom = 13.6 eV
- Energy of the incoming photon = 12.1 eV
The energy of the electron in the excited state can be calculated by adding the energy of the incoming photon to the ionization potential of the hydrogen atom.
According to Bohr's model, the energy of an electron in the nth orbit is given by:
Rearranging the equation gives:
The electron is now in the n=3 state. The possible transitions (and thus spectral lines) are:
1. From n=3 to n=2
2. From n=3 to n=1
3. From n=2 to n=1
The total number of spectral lines emitted corresponds to the possible transitions:
- 3 to 2
- 3 to 1
- 2 to 1
The spectral lines emitted by hydrogen atoms according to Bohr's theory will be 3.
Topper's Solved these Questions
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - Check point 12.1
14 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - Check point 12.2
16 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - JEE MAIN
62 Videos
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
Similar Questions
Ionization potential of hydrogen atom is 13.6 eV. Hydrogen atoms in the ground state are excited by monochromatic radiation of photon energy 12.1 eV. According to Bohr's theory, the spectral lines emitted by hydrogen will be
View SolutionIonisation potential of hydrogen atom is 13.6eV. Hydrogen atom in ground state is excited by monochromatic light of energy 12.1eV. The spectral lines emitted by hydrogen according to Bohr's theory will be
View SolutionAn H- atom in the ground state is excited by monochromatic radiation of photon energy 13.056 eV. The number of emission lines will be (given its ionisation energy is 13.6 eV)
View SolutionHydrogen atom in ground state is excited by a monochromatic radiation of λ=975Å. Number of spectral lines in the resulting spectrum emitted will be
View SolutionA sample consisting of Hydrogen atoms in the ground state is excited by monochromatic radiation of energy 12.75 eV. If we were to observe the emission spectrum of this sample, then the number of spectral lines observed, will be
View SolutionExcitations energy of hydrogen atom is 13.6eV match the following
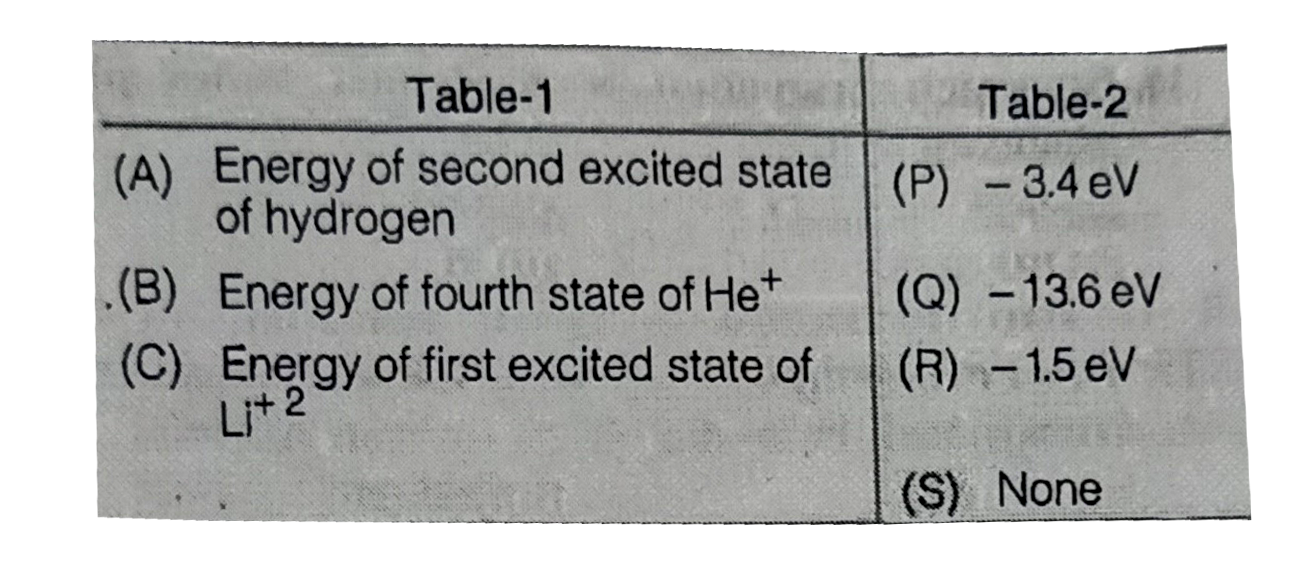
View SolutionThe ionisation potential of hydrogen atom is -13.6 eV. An electron in the ground state of a hydrogen atom absorbs a photon of energy 12.75 eV. How many diggerent spectral lines can one expect when the electron make a downward transition
View SolutionThe energy of electron in an excited hydrogen atom is −3.4eV. Its angular momentum according to bohr's theory will be
View SolutionThe hydrogen atom in ground state is excited by a monochromatic radiation of wavelength λ=1025A. The possible number of emmision spectral lines in the spectrum will be (hc = 12400 eV A)
View SolutionA hydrogen atom in the ground state is excited by an electron beam of 12.5 eV energy. Find out the maximum number of lines emitted by the atom from its excited state.
View Solution
