Is it possible for a moving object to have instantaneous velocity equal to the average velocity ?
Yes , it is possible only in motion of an object in one dimension . It is not possible in case when object is moving in two or three dimensions.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - TOUGH & TRICKY ( PROBLEMS)10videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - NCERT (TEXTBOOK EXERCISES )22videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - PRACTICE PROBLEMS28videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test15videos
MOTION IN A PLANE
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)12videos
OSCILLATIONS
Similar Questions
Knowledge Check
- Question 1 - Select One
The trajectory of a particle moving in vast maidan is as shown in the figure. The coordinates of a position A are (0,2). The coordinates of another point at which the instantaneous velocity is same as the average velocity between the points are
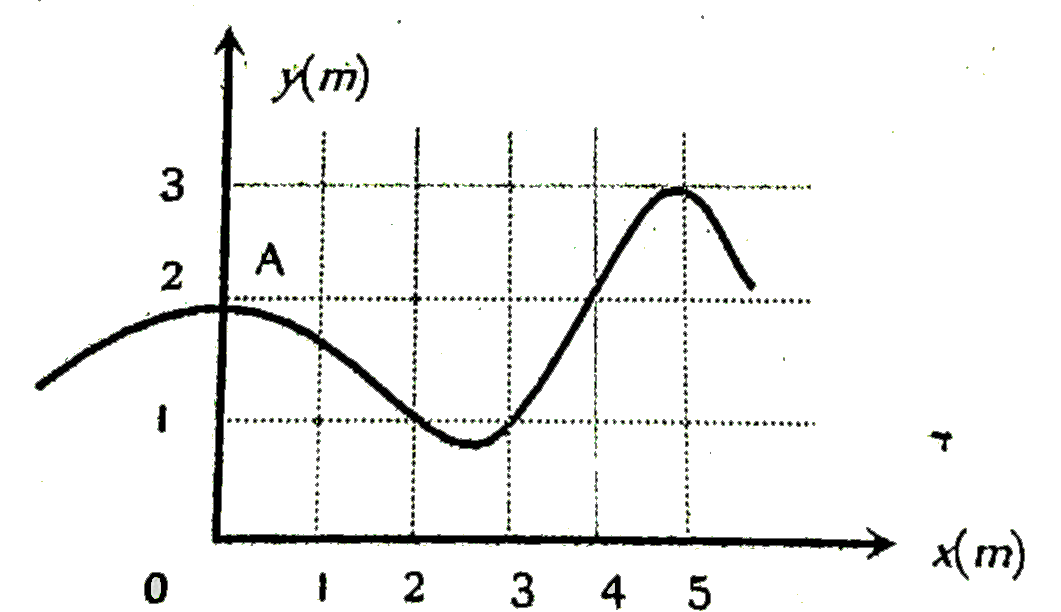A(1,4)B(5,3)C(3, 4)D(4, 1) - Question 2 - Select One
Position time graph is shown which is a semicircle form t=2 to t=8sec. Find time t at which the instantaneous velocity, is equal to average velocity over first t second.
A4.8secB3.2secC2.4secD5sec - Question 3 - Select One or More
The instantaneous velocity of a body can be measured
AGraphicallyBVectoriallyCBy speedometerDNone of these
A cockroach moves rectilinearly such that after sometime t0 let its (instantaneous) velocity be equal to its average velocity over that time. Referring to the SΔt graph as shown in , for the motion of the cockroach, find the time t0 and the average velocity of the cockroach over the time t0
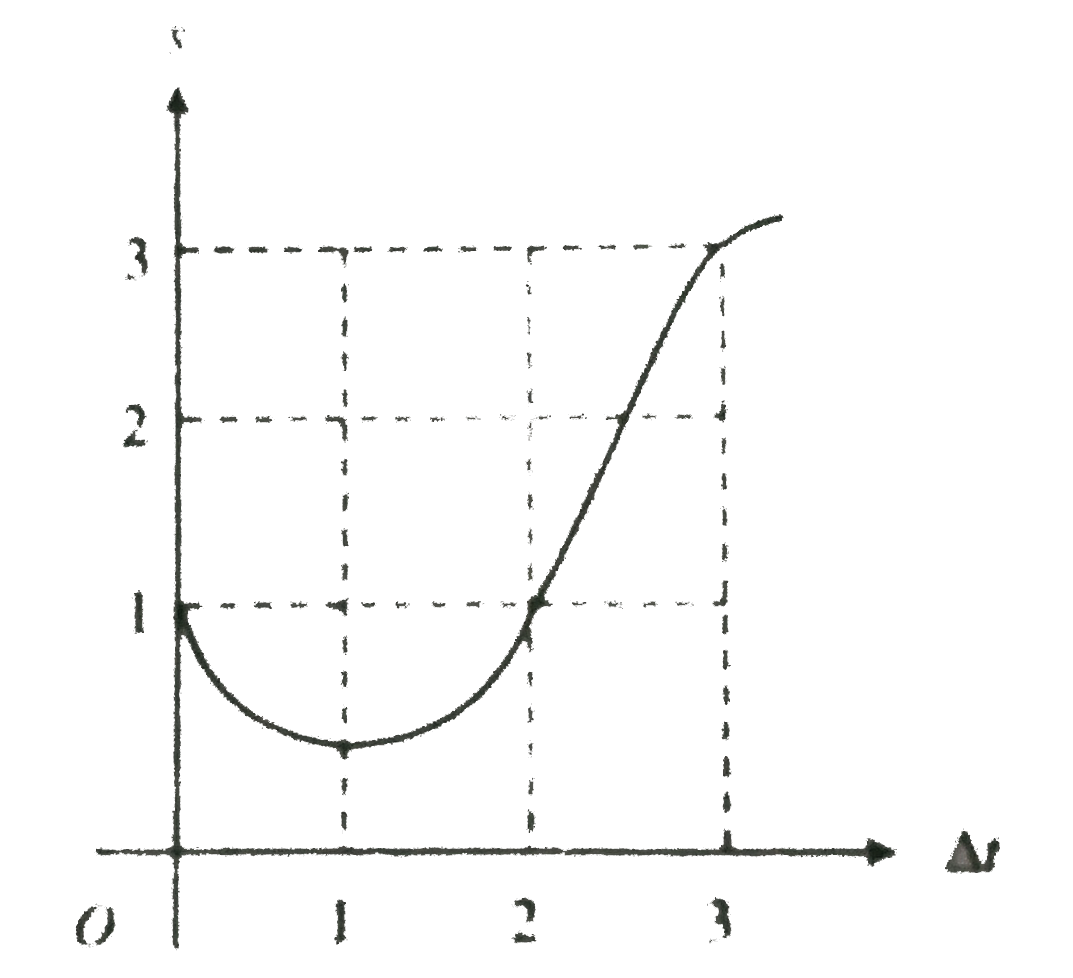. View SolutionIs it possible for a uniformly accelerating object to change its direction of velocity ?
View SolutionIs it possible for a uniformly accelerating object to change its direction of velocity?
View SolutionAssertion: The instantaneous velocity does not depend on instantaneous position vector.
Reason: The instantaneous velocity and average velocity of a particle are always same.View SolutionThe instantaneous velocity of a particle is equal to time derivative of its position vector and the instantaneous acceleration is equal to time derivatives of its velocity vector. Therefore :
View Solution