Kα radiation of Mo (Z = 42) has a wavelength of 0.71Å. Calculate wavelength of the corresponding radiation of Cu (Z=29) Solution in Kannada
1.45Å
1.52Å
0.52Å
1.02Å
The correct Answer is:B
From the Mosley.s law, we have
v=a(Z−σ)2
for Kα radiation screening constant σ=1andv=cλ
∴1λ∝(Z−1)2
λCuλMo=(ZMo−1)2(ZCu−1)1=412282
∴λMo=0⋅71Å
Then λCu=(0.71)Å×412282=1⋅52Å
Topper's Solved these Questions
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-III)
9 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS
4 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
Similar Questions
Energy required for the electron excitation in Li++ from the first to the third Bohr orbit is :
View SolutionAccording to Einstein's photoelectric equation the graph of K.E. of the photoelectron emitted from the metal versus the frequency of the incident radiation gives a straight line graph whose slope
View SolutionAccording to Einstein's photoelectric equation the graph of K.E. of the photoelectron emitted from the metal versus the frequency of the incident radiation gives a straight line graph whose slope
View SolutionThe speed of electromagnetic wave in vacuum depends upon the source of radiation
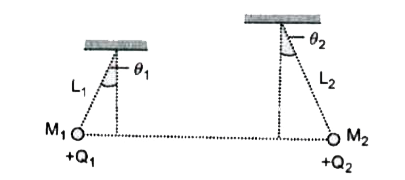
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionIf the radiation of Mo (Z = 42) has a wevelength of 0.71Å , calculate wevelength of the corresponding radiation of Cu,i.e. , k_(alpha)for Cu (Z = 29) assuming b = 1 .
View SolutionIf λCu is the wavelength of Kα X-ray line of copper (atomic number 29) and λMo is the wavelength of the KαX-ray line of molybdenum (atomic number 42),then the ratio λCu/λMo is close to
View SolutionThe K_(alpha) line obtained for molybdenum (Z = 42) is 0.71 Å. Then, the wavelength of the K_(alpha) line of copper (Z = 29) is
View SolutionIf wavelength of Kα from Z = 46 is λ, then find out wavelength of Kα from Z = 31.
View SolutionIf the wavelength of K_(alpha) radiation emitted an atom of atomic number Z = 41 is lambda , then the atomic number for an atom that emits K_(alpha) radiation with the wavelength 4lambda , is
View Solutionयदि Mo(Z = 42) के Kα विकिरण का तरंगदैर्घ्य 0.71Å है, तो Cu(Z = 29) के संगत विकिरण का तरंगदैर्घ्य है
View Solutionमॉलिब्डेनम (Z=42) के लिए Kα तरंगदैर्ध्य निकालें।
View Solutionतांबे (परमाणु क्रमांक 29) की Kα X- किरण रेखा की तरंगदैर्ध्य λcu है तथा मॉलिब्डेनम (परमाणु क्रमांक 42) की Kα X- किरण रेखा की तरंगदैर्ध्य λmo है, तब अनुपात λcu/λmo लगभग है
View SolutionIf the Kα radiation of Mo(Z=42) has a wevelength of 0.71Å, calculate wevelength of the corresponding radiation of Cu,i.e.,kαforCu(Z=29) assuming b = 1.
View Solutionएक परमाणु के लिए परमाणु क्रमांक ज्ञात कीजिए, जो 4λ तरंग दैर्ध्य का Kα विकिरण उत्सर्जित करता है, यदि परमाणु क्रमांक Z=11 के परमाणु द्वारा उत्सर्जित विकिरण Kα की तरंगदैर्ध्य λ है।
View Solution
