Two small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
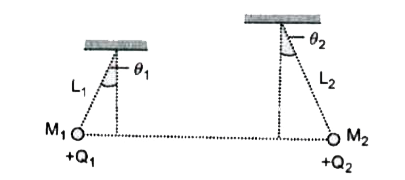
M1≠M2,θ1=θ2
M1=M2
θ1=θ2
L1=L2
The correct Answer is:B
For sphere 1 , in equilibrium
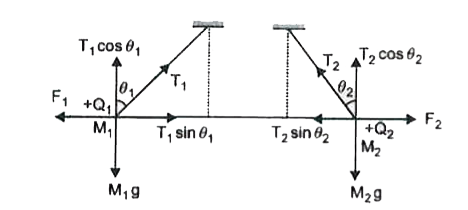
T1cosθ1=M1g
and T1cosθ1=F1
∴ taθ1=F1M1g
Similarly for sphere 2 , tanθ2=F2M2g
F is same on both the charges , θ will be same only if their masses M are equal .
Topper's Solved these Questions
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - LEVEL-III
23 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- GRAVITATIONBOOK - MODERN PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - Recent Competitive Questions
8 Videos
Similar Questions
Two small spheres of masses M1and M2 are suspended by weightless insulating threads of lengths L1 and L2. The spheres carry charges Q1 and Q2 respectively. The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles θ1 and θ2respectively . Which one of the following conditions is essential for θ1=θ2 ?
View SolutionTwo positively charged sphere of masses m1 and m2 are suspended from a common point at the ceiling by identical insulating massless strings of length l. Charges on the two spheres are q1 and q2 respectively. Equilibrium both strings make the same angle θ with the vertical. then
View Solution
Knowledge Check
Two small spheres of masses M1and M2 are suspended by weightless insulating threads of lengths L1 and L2. The speres carry charges Q1 and Q2 respectively. The spheres are suspended such that they are in level with one another adn the threads are inclined to the verticle at angles θ1 and θ2respectively . Which one of the following conditions is essential for θ1=θ2 ?
AM1≠M2 , but Q1=Q2BQ1=Q2CL1=L2DM1=M2Two small spheres with mass m1 and m2 hang from massless insulating threads of length l1 and l2. The two spheres carry charges q1 and q2 respectively. The spheres are hung such that they are on the same horizontal level and the threads are inclined to the vertical at angles θ1 and θ1. Which of the condition is required if θ1=θ2?
 AIf m1=m2B|q1|=|q2|Cl1=l2Dq1m1=q2m2
AIf m1=m2B|q1|=|q2|Cl1=l2Dq1m1=q2m2Two positively charged spheres of masses m1, and m2 are suspended from a common point at the ceiling by identical insulating massless strings of length l. Charged on the two spheres are q1 and q2, respectively. At equilibrium both strings make the same angle θ with the vertical. Then
Aq1m1=q2m2Bm1=m2Cm1=m2sinθDq2m1=q1m2
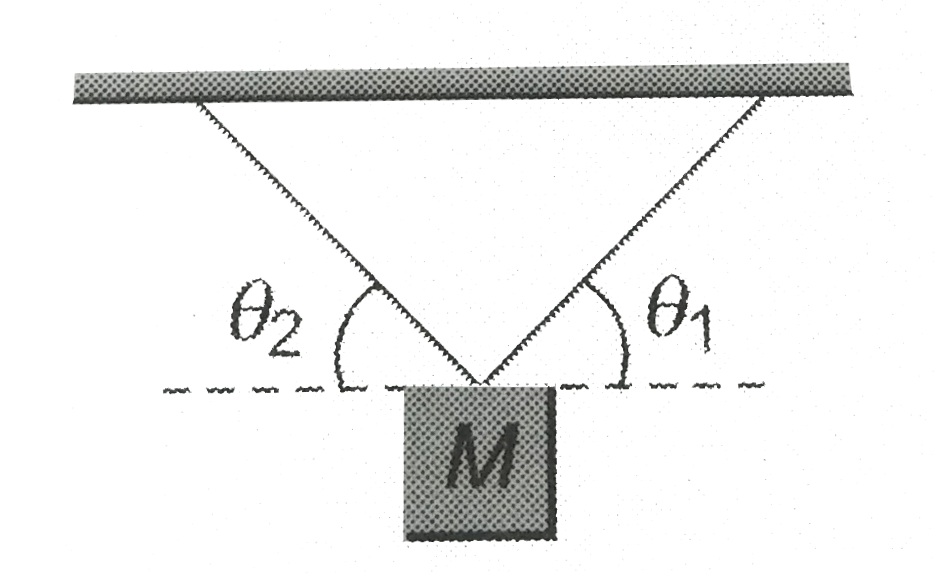
A block of mass M si suspended by two strings angles θ1 and θ2 with the horizontal. Find the tensions in the strings.
View SolutionTwo identical spheres of mass m and charge q are suspended from a common point by two threads of the same length l and it is found that threads make an angle θ with the vertical when the spheres are in equilibrium . Calculate the value of q.
View SolutionTwo positively charged sphere of masses m1 and m2 are suspended from a common point at the ceiling by identical insulating massless strings of length l. Charges on the two spheres are q1 and q2 respectively. Equilibrium both strings make the same angle θ with the vertical. then
View SolutionA small sphere of mass m suspended by a thread is first taken a side so that the thread forms the right angle with the vertical and then released, then
View SolutionTwo identical small spheres carry charge of Q1 and Q2 with Q1>>Q2. The charges are d distance apart. The force they exert on one another and then spheres are made to touch one another and then separated to distance d apart. The force they exert on one another now is F2. Then F1/F2 is :-
View Solution
