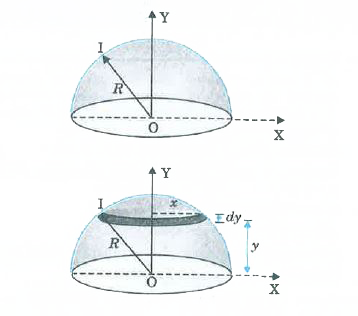
Locate the position of the centre of mass of a hemisphere of radius R as shown in the following figure:

Video Solution

Text Solution

Verified by Experts

Let the density of hemisphere be $ρ$ . The hemisphere is constructed with a large number of co-axial discs. One such elementary disc of radius and thickness dy is at a distance y from the origin as shown above. Here the radius of the elemental disc is, $x = \sqrt{R^{2} - y^{2}}$
Mass of the elementary disc=Volume $\times$ density
$= (π x^{2} d y) = π (R^{2} - y^{2}) d y ρ$
The Y-coordinate of centre of mass are given by
$Y_{c m} = \frac{1}{m} \int d m y$
Here, $m = \frac{2}{3} π R^{3} ρ$
$Y_{c m} = \frac{1}{m} \int y d m = \frac{1}{m} \int_{0}^{R} y π (R^{2} - y^{2}) ρ d y$
$= \frac{π ρ}{m} \int_{0}^{R} (R^{2} y - y^{3}) d y$
$= \frac{π ρ}{m} {[\frac{R^{2} y^{2}}{2} - \frac{y^{4}}{4}]}_{0}^{R}$
$= \frac{π ρ}{m} [\frac{R^{4}}{2} - \frac{R^{4}}{4}] = \frac{π ρ}{m} (\frac{R^{4}}{4})$
$= \frac{π ρ}{\frac{2}{3} π R^{3} ρ} (\frac{R^{4}}{4}) = \frac{3}{8} R$
Also, $Z_{c m} = \int z d m = 0 and X_{c m} = \int x d m = 0$
$∴$ The coordinates of centre of mass are $(0, \frac{3}{8} R, 0)$

Updated on:21/07/2023