Why the handles of the doors are at maximum distance from the hinges?
To understand why the handles of doors are placed at maximum distance from the hinges, we need to consider the concept of torque and how it affects the motion of the door.
1. Understanding Torque:
Torque (τ) is a measure of the rotational force applied to an object. It is calculated using the formula:
τ=F×R×sin(θ)
where F is the force applied, R is the distance from the pivot point (hinge) to the point where the force is applied (handle), and θ is the angle between the force vector and the lever arm.
2. Position of the Hinge:
The hinge of the door acts as the pivot point. When the door is pushed or pulled at the handle, it rotates around this hinge.
3. Distance from the Hinge:
The handle is positioned far from the hinge to maximize the distance R. The further the handle is from the hinge, the greater the torque produced for the same amount of force applied.
4. Maximizing Torque:
Since torque is directly proportional to the distance R, having the handle at the maximum distance from the hinge allows for a larger torque to be generated. This means that less force is required to open or close the door.
5. Ease of Operation:
By maximizing the torque, it becomes easier to operate the door. A person can apply a smaller force at the handle, and due to the increased torque, the door will still open or close effectively.
6. Conclusion:
Therefore, the handles of doors are placed at maximum distance from the hinges to ensure that the torque generated is maximized, making it easier to open and close the door with minimal effort.
Final Answer:
The handles of the doors are at maximum distance from the hinges so that the torque generated is maximum, making the door easier to open.
---
Torque (τ) is a measure of the rotational force applied to an object. It is calculated using the formula:
τ=F×R×sin(θ)
where F is the force applied, R is the distance from the pivot point (hinge) to the point where the force is applied (handle), and θ is the angle between the force vector and the lever arm.
The hinge of the door acts as the pivot point. When the door is pushed or pulled at the handle, it rotates around this hinge.
The handle is positioned far from the hinge to maximize the distance R. The further the handle is from the hinge, the greater the torque produced for the same amount of force applied.
Since torque is directly proportional to the distance R, having the handle at the maximum distance from the hinge allows for a larger torque to be generated. This means that less force is required to open or close the door.
By maximizing the torque, it becomes easier to operate the door. A person can apply a smaller force at the handle, and due to the increased torque, the door will still open or close effectively.
Therefore, the handles of doors are placed at maximum distance from the hinges to ensure that the torque generated is maximized, making it easier to open and close the door with minimal effort.
The handles of the doors are at maximum distance from the hinges so that the torque generated is maximum, making the door easier to open.
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (INTEGER TYPE QUESTIONS) )
15 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Knowledge Check
The earth is at its maximum distance from the sun on:
Ajuly 4Bjanuary 30CSeptember 22DDecember 22A door can just be opened with 10N force on the handle of the door. The handle is at a distance of 50cm from the hinges. Then, the torque applied on the door (in Nm) is
A5B10C15D20The earth is at its maximum distance from the Sun on :
AJanuary 30 thBDecember 22ndCSeptember 22 ndDJuly 4 th
A nut cracker is used to crack a nut. In it nut is held in two arms at a distance 2 cm from the hinge and the handle of it is at a distance 25 cm from hinge. The force required to crack a walnut is 30 N. What is the minimum force required to crack it with the nut cracker.
[2.4 N]
View SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
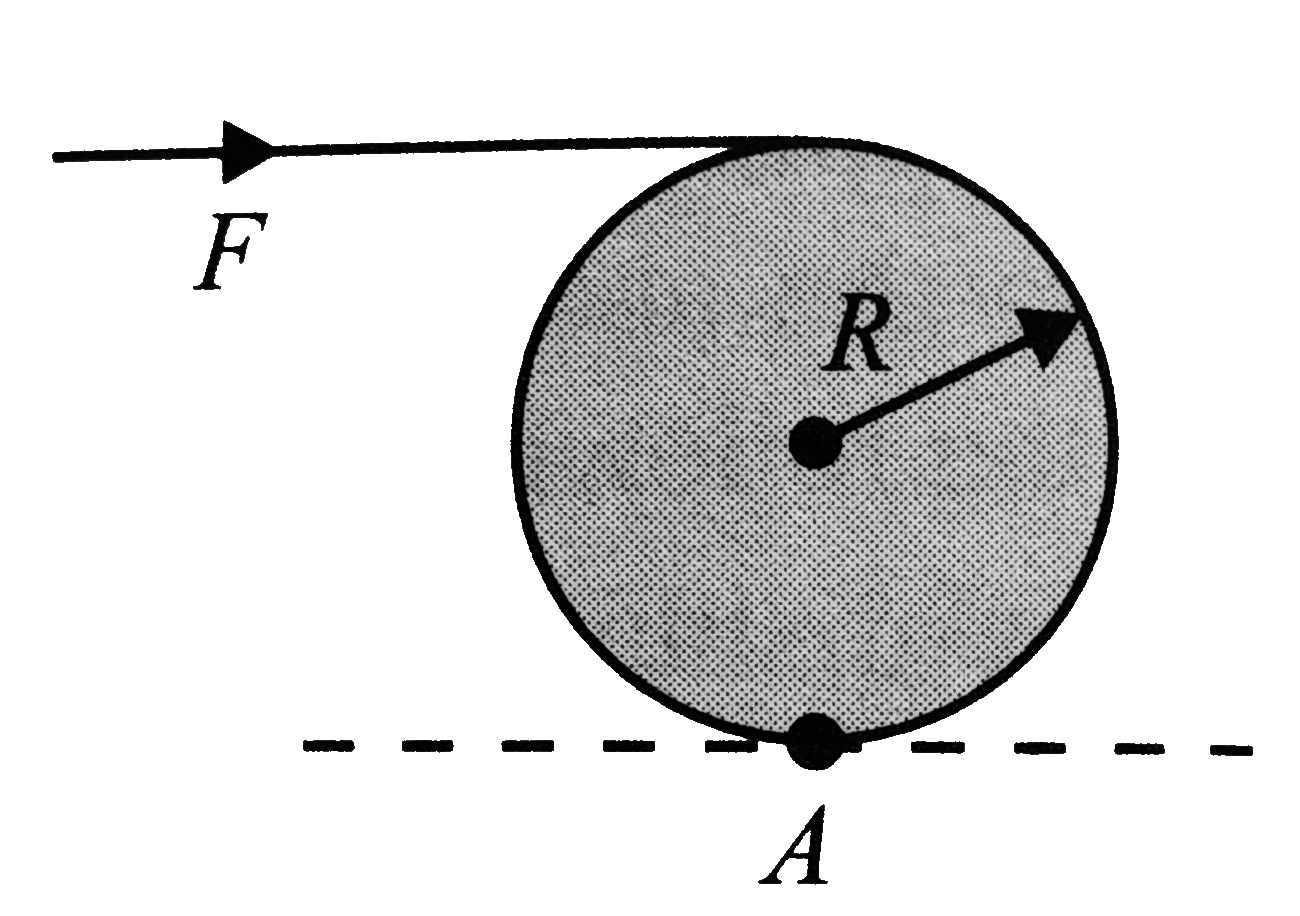
Component of reaction at the hinge in the horizontal direction is
View SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
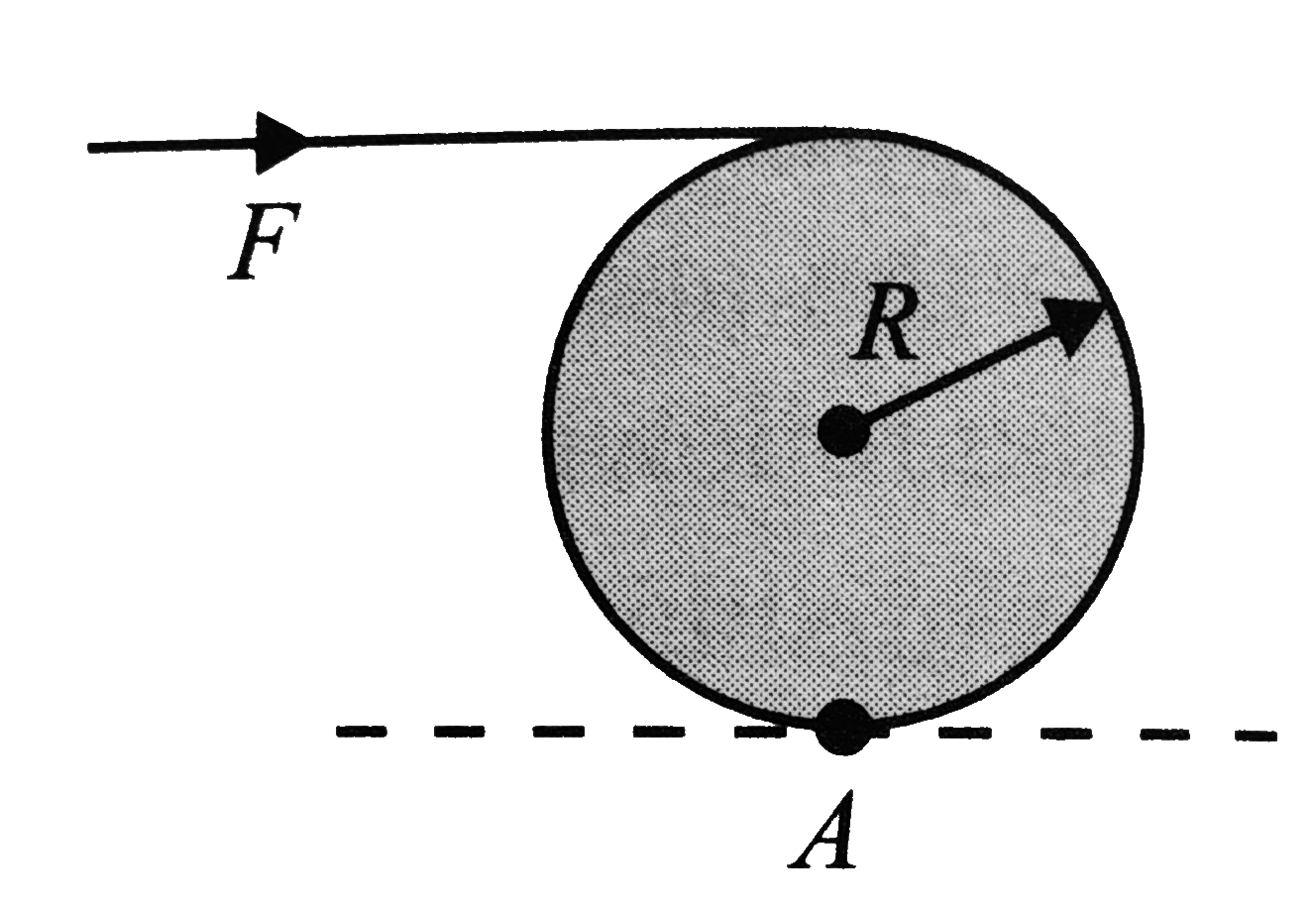
Component of reaction at the hinge in the vertical direction is
View SolutionWhen a body is hinged at a point and a force is acting on the body in such a way that the line of action of force is at some distance from the hinged point, the body will start rotating about the hinged point. The angular acceleration of the body can be calculated by finding the torque of that force about the hinged point. A disc of mass m and radius R is hinged at point A at its bottom and is free to rotate in the vertical plane. A force of magnitude F is acting on the ring at top most point.
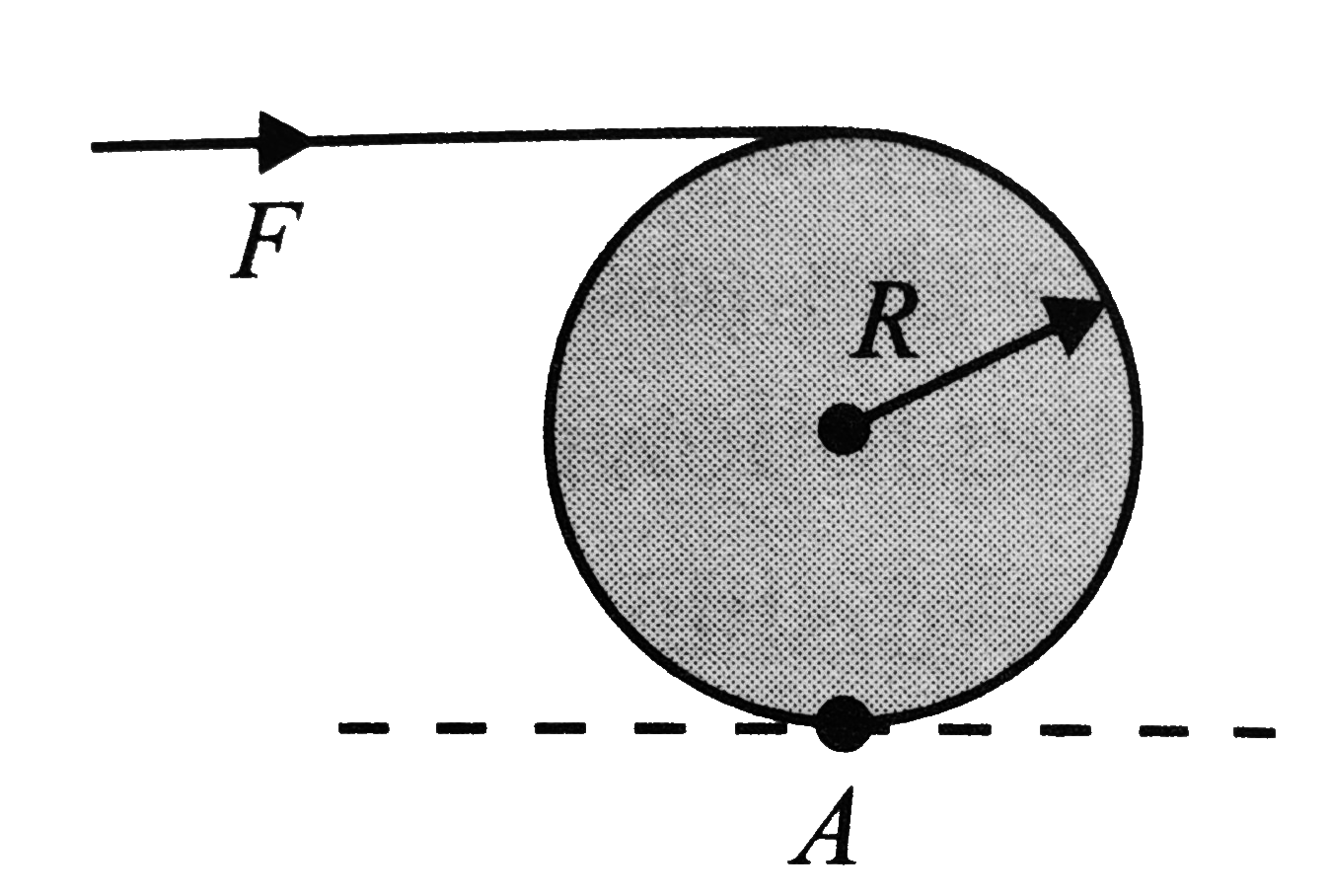
Tangential acceleration of the centre of mass is
View SolutionA door 1.6 m wide requires a force of 1N to be applied at the free and to open or close it. The force that is required at a point 0.4 m distance from the hinges for o[ening or closing the door is
View Solution
