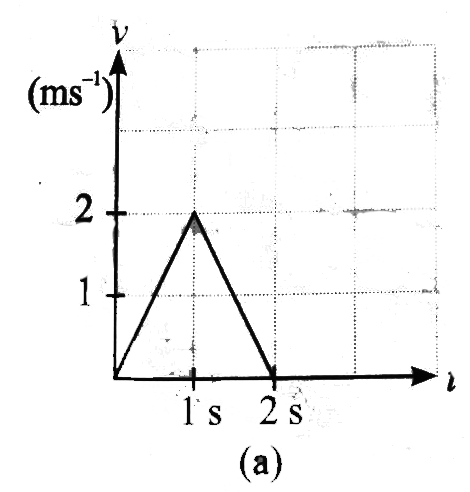

Figure shows (vx,t) and (vy,t) diagram for a body of unit mass. Find the force as a function of time.
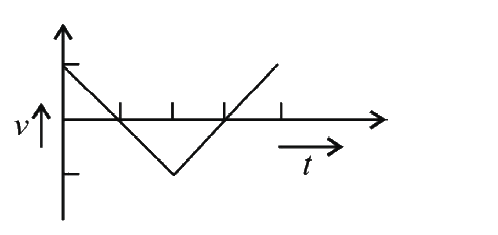
Consider figure(a) vx=2t for 0<t<1s
= 2(2−t) for 1<t<1s
= 0 for t > 2s.
From figure (b) vy=t for 0<t<1s
= 1 for t > 1s
Fx=max=mdvxdt
=1×2 for0<t<1s
=1(−2) for1<t<2s
for2<t

Fy=may=mdvydt
=1×1 for 0<t<1s=0 for 1<t
Hence, F=Fxˆi+Fyˆj
=2ˆi+ˆj for0<t<1s
=−2ˆi for 1<t<2s
=0 for t>2s
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - NCERT EXEMPLARCHAPTER - LAWS OF MOTIONEXERCISE - Short answer type questions
6 Videos
- KINETIC THEORYBOOK - NCERT EXEMPLARCHAPTER - KINETIC THEORYEXERCISE - Multiple Choice Questions (MCQs)
31 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT EXEMPLARCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions
3 Videos
Similar Questions


Figure shows (vx,t) and (vy,t) diagram for a body of unit mass. Find the force as a function of time.
View SolutionFigure shows (x,t) (y,t) diagram of a particle moving in 2-dimensions.
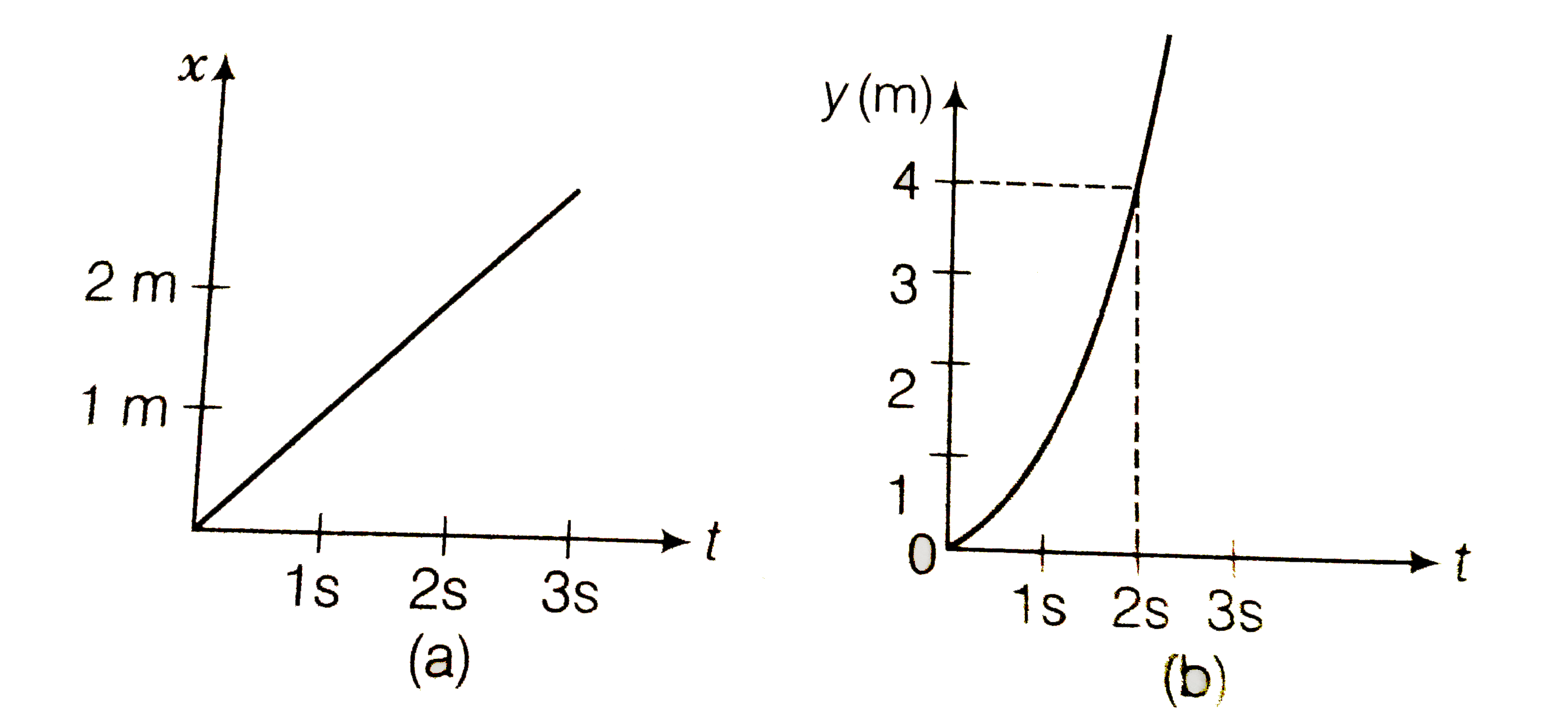
If the particle has a mass of 500 g , find the force (direction and magnitude) acting on the particle .
View Solution
Knowledge Check
The work done in time t on a body of mass m which is accelerated from rest to a speed v in time t1 as a function of time t is given by
A12mvt1t2Bmvt1t2C12(mvt1)2t2D12mv2t21t2Work done in time t on a body of mass m which is accelerated from rest to a speed v in time t1 as a function of time t is given b
A12mvt1t2Bmvt1t2C12(mvt1)2t2D12mv2t21t2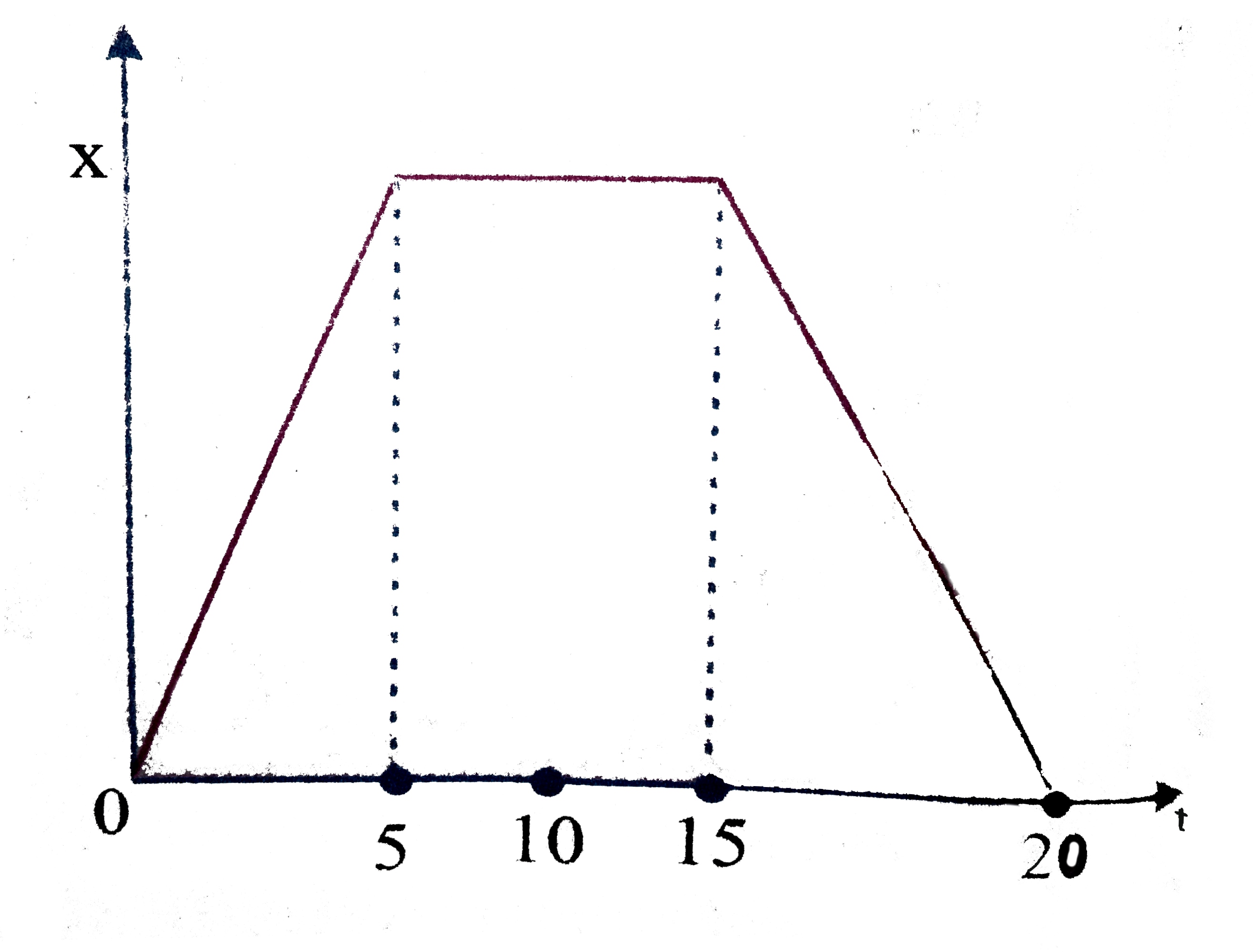
Figure shows the displacement tiime (x-t) graph of a body moving in a straight line which one of the graph shown in figure represents the velocity- time (v-t) graph of the motion of the body.A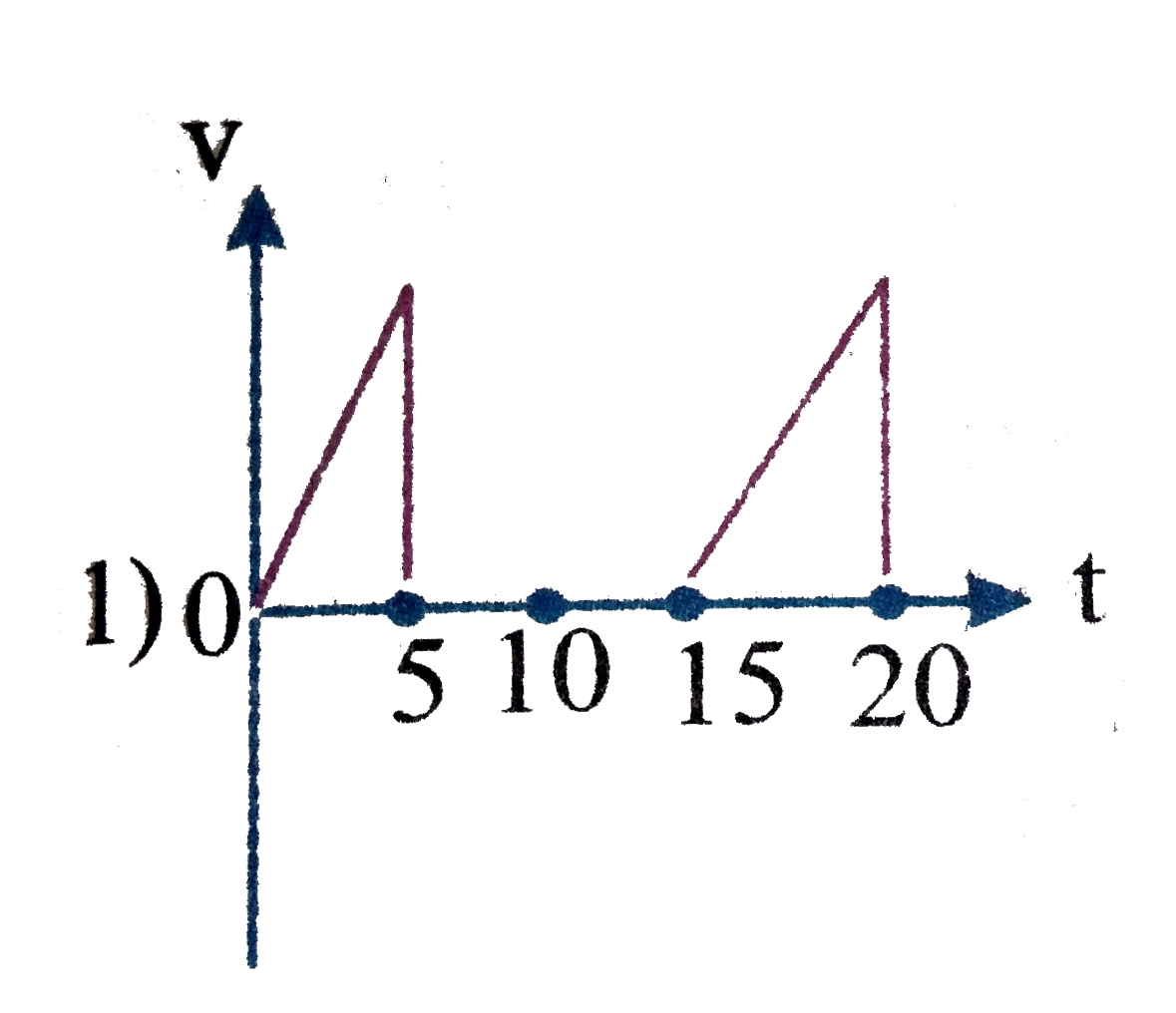 B
B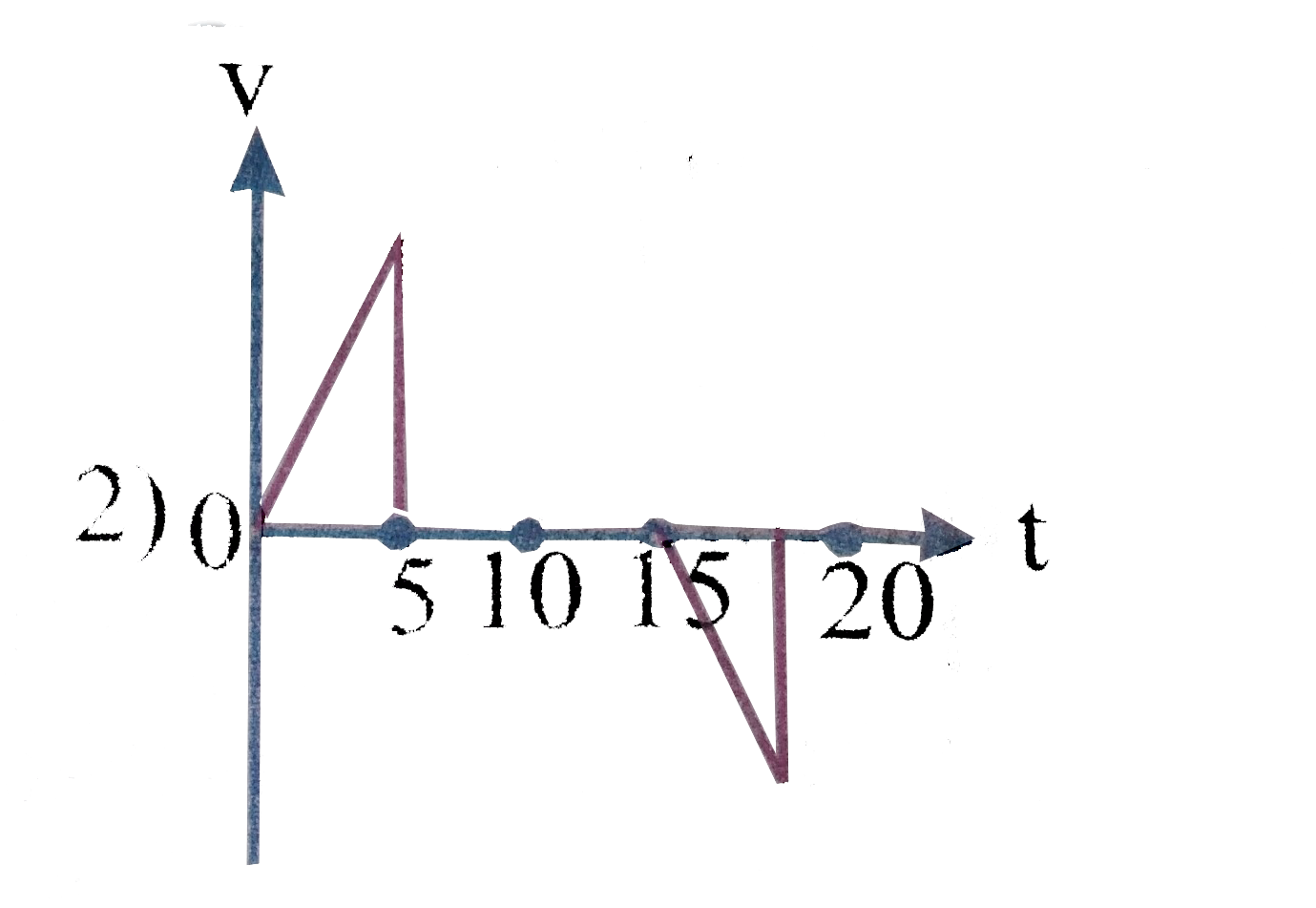 C
C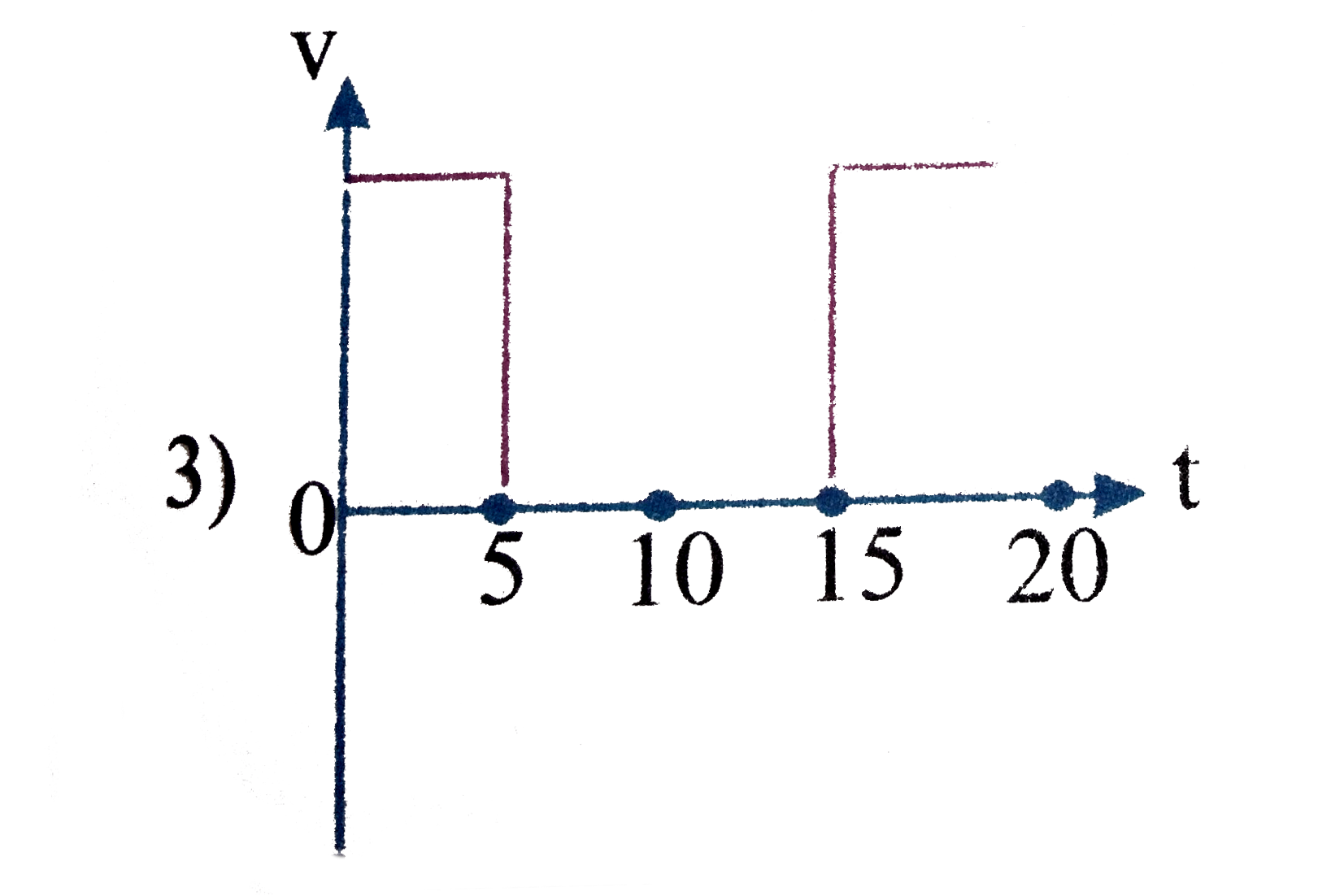 D
D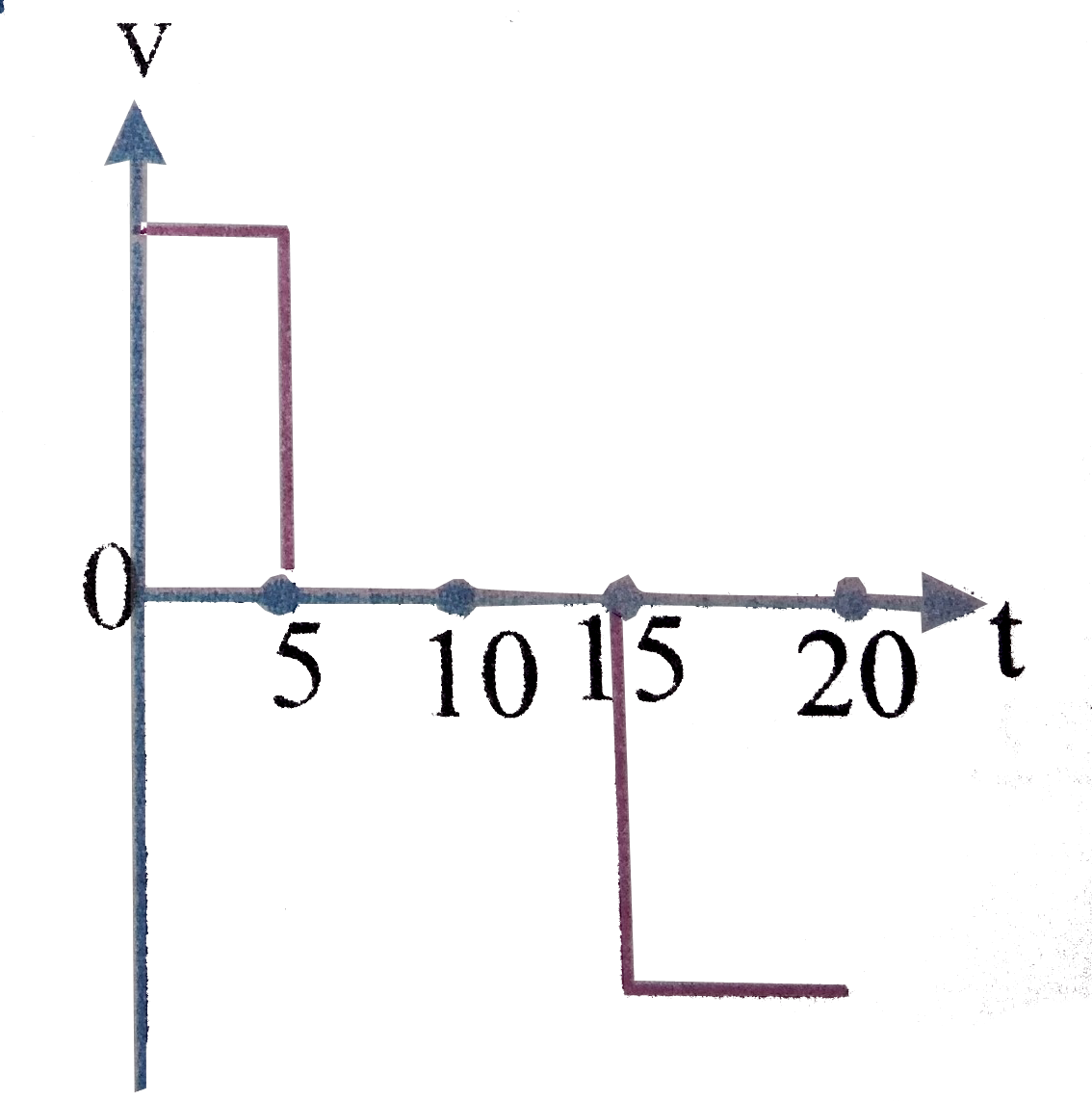
In the figure show,
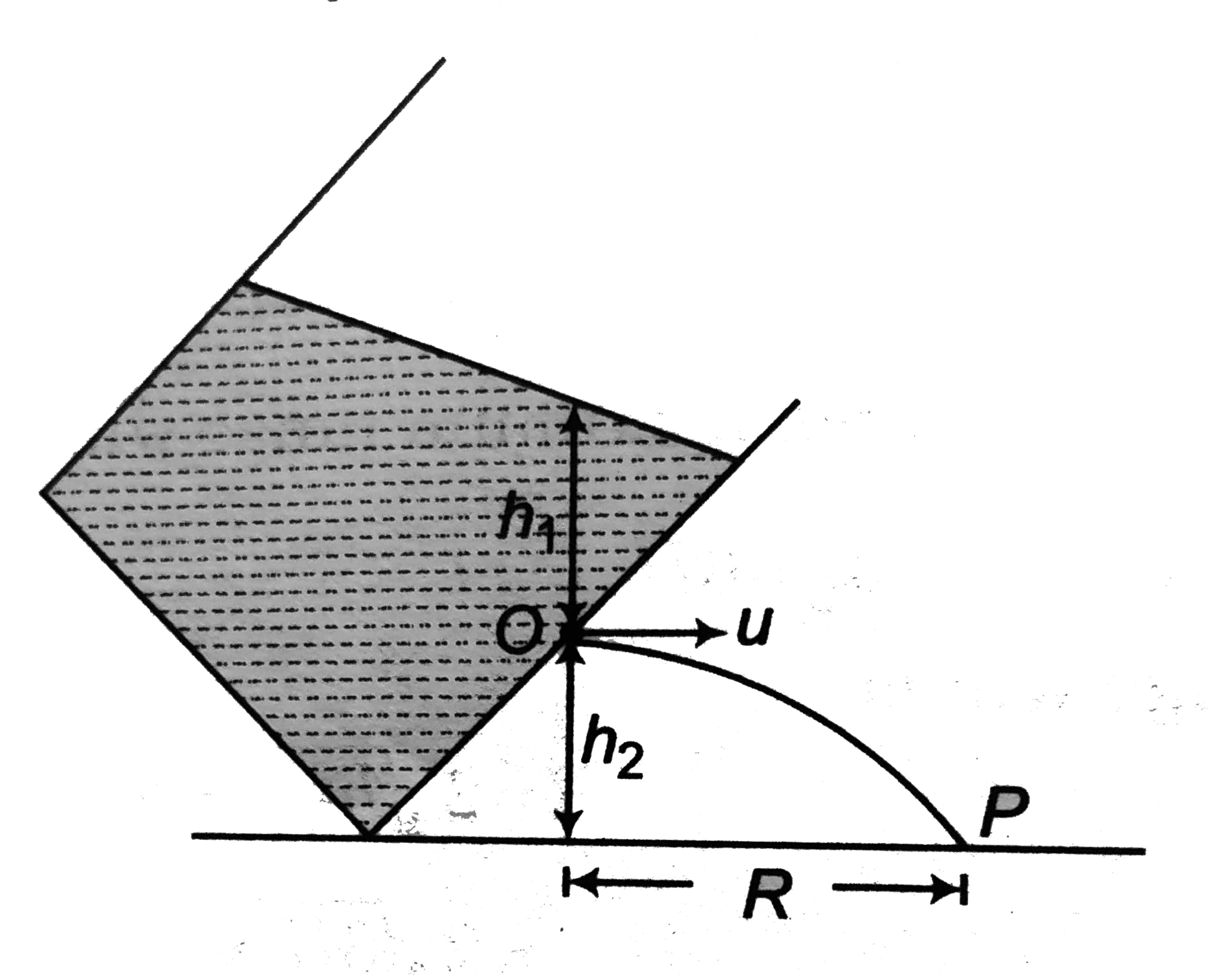
find v,top and R.
View SolutionA body of mass m, accelerates uniformly from rest to v1 in time t1. Find the instantaneous power delivered to the body as a function of time t.
View SolutionThe graph in figure shows the velocity v versus time t for a body. Which of the graphs shown in figure represents the corresponding acceleration versus time graphs ?
View SolutionA body of mass m is acceleratad uniformaly from rest to a speed v in a time T . The instanseous power delivered to the body as a function of time is given by
View SolutionWork done in time t on a body of mass m which is accelerated from rest to a speed v in time t1 as a function of time t is given b
View Solution
