Net charge on a capacitor is always zero, then what does a capacitor store? Explain.
To answer the question, "Net charge on a capacitor is always zero, then what does a capacitor store?" we can break down the explanation into several steps:
1. Understanding Capacitor Structure:
- A capacitor consists of two conductive plates separated by an insulating material (dielectric). When connected to a battery, one plate accumulates positive charge (+Q) while the other plate accumulates an equal amount of negative charge (-Q).
2. Net Charge on the Capacitor:
- The total charge on the capacitor is the sum of the charges on both plates. Since one plate has +Q and the other has -Q, the net charge on the capacitor is:
Qnet=+Q+(−Q)=0
- Therefore, the net charge on the capacitor is indeed zero.
3. Charge Distribution:
- Although the net charge is zero, the capacitor has charges of equal magnitude but opposite sign on its plates. This separation of charge creates an electric field between the plates.
4. Electric Field:
- The electric field (E) between the plates is created due to the potential difference (voltage) applied across the capacitor. The electric field is directed from the positively charged plate to the negatively charged plate.
5. Energy Storage:
- The capacitor stores energy in the form of an electric field generated between the plates. The energy (E) stored in a capacitor can be expressed mathematically as:
E=12CV2
or
E=Q22C
- Where C is the capacitance of the capacitor, V is the potential difference across the capacitor, and Q is the charge on one of the plates.
6. Conclusion:
- In summary, a capacitor stores energy in the form of an electric field created by the separation of charges on its plates. Even though the net charge is zero, the individual charges on the plates are non-zero, allowing the capacitor to store energy effectively.
- A capacitor consists of two conductive plates separated by an insulating material (dielectric). When connected to a battery, one plate accumulates positive charge (+Q) while the other plate accumulates an equal amount of negative charge (-Q).
- The total charge on the capacitor is the sum of the charges on both plates. Since one plate has +Q and the other has -Q, the net charge on the capacitor is:
Qnet=+Q+(−Q)=0
- Therefore, the net charge on the capacitor is indeed zero.
- Although the net charge is zero, the capacitor has charges of equal magnitude but opposite sign on its plates. This separation of charge creates an electric field between the plates.
- The electric field (E) between the plates is created due to the potential difference (voltage) applied across the capacitor. The electric field is directed from the positively charged plate to the negatively charged plate.
- The capacitor stores energy in the form of an electric field generated between the plates. The energy (E) stored in a capacitor can be expressed mathematically as:
E=12CV2
or
E=Q22C
- Where C is the capacitance of the capacitor, V is the potential difference across the capacitor, and Q is the charge on one of the plates.
- In summary, a capacitor stores energy in the form of an electric field created by the separation of charges on its plates. Even though the net charge is zero, the individual charges on the plates are non-zero, allowing the capacitor to store energy effectively.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Long Answer Questions)
13 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Numerical Problems)
25 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Fill in the Blanks)
11 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
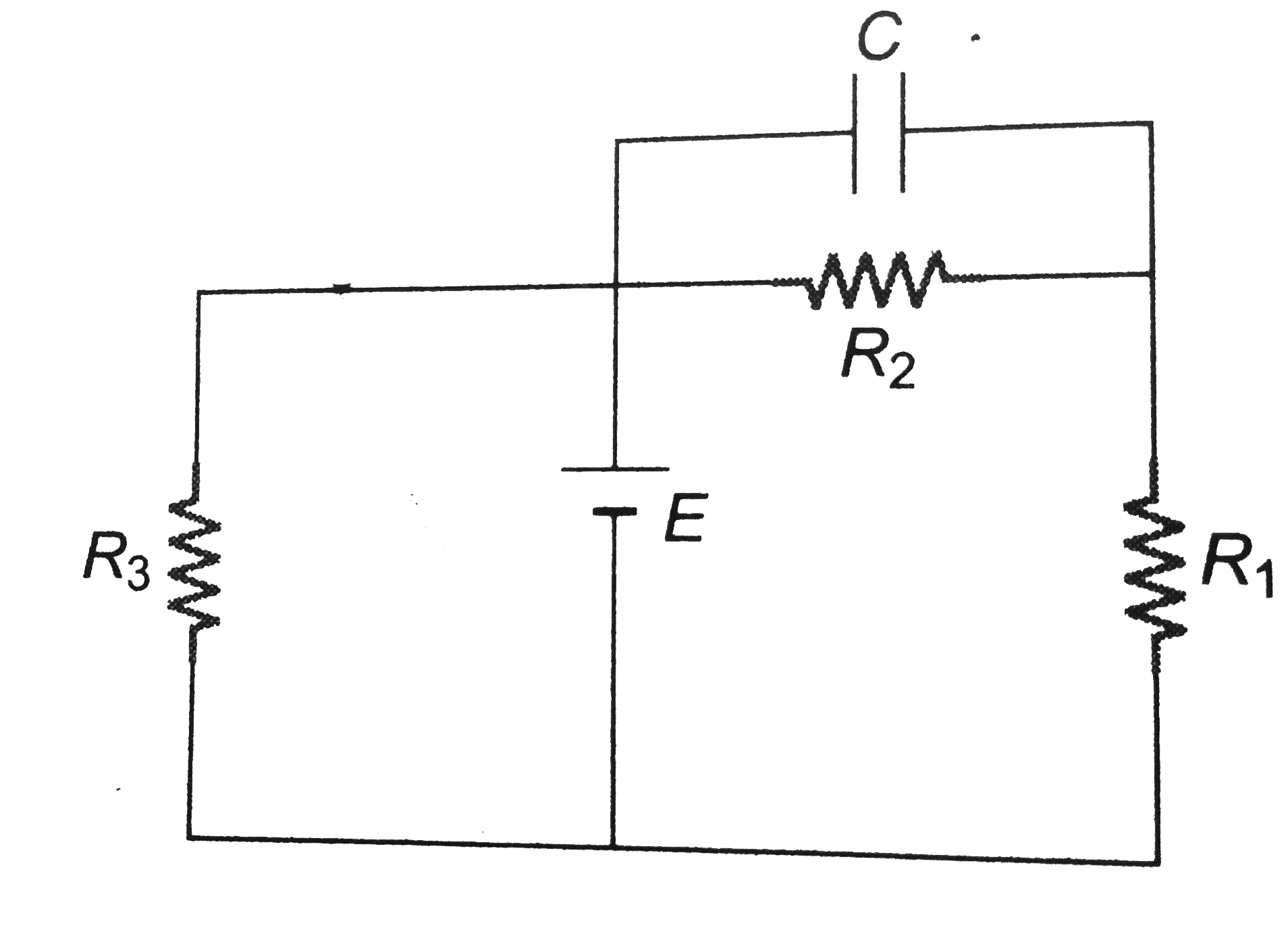
The charge on the capacitor is initially zero. Find the charge on the capacitor as a function of time t. All resistors are of equal value R.
View SolutionIf the potentail difference across a capacitor is doubled, what happens to : (a) the charge on the capacitore and (b) the energy stored in the capacitor
View Solution
Knowledge Check
The net charge on a capacitor is
AinfiniteBzeroCfiniteDdepends on size of capacitorIn an oscillating LC circuit the maximum charge on the capacitor is Q. The charges on the capacitor when the energy is stored equally between the electric and magnetic field is
AQ/2BQ/√3CQ/√2DQIf the charge on a capacitor is increased by 2C, then the energy stored in it increases by 21 %. The original charge on the capacitor is
A10 CB20 CC30 CD40 C
(a) Find charge on capacitors
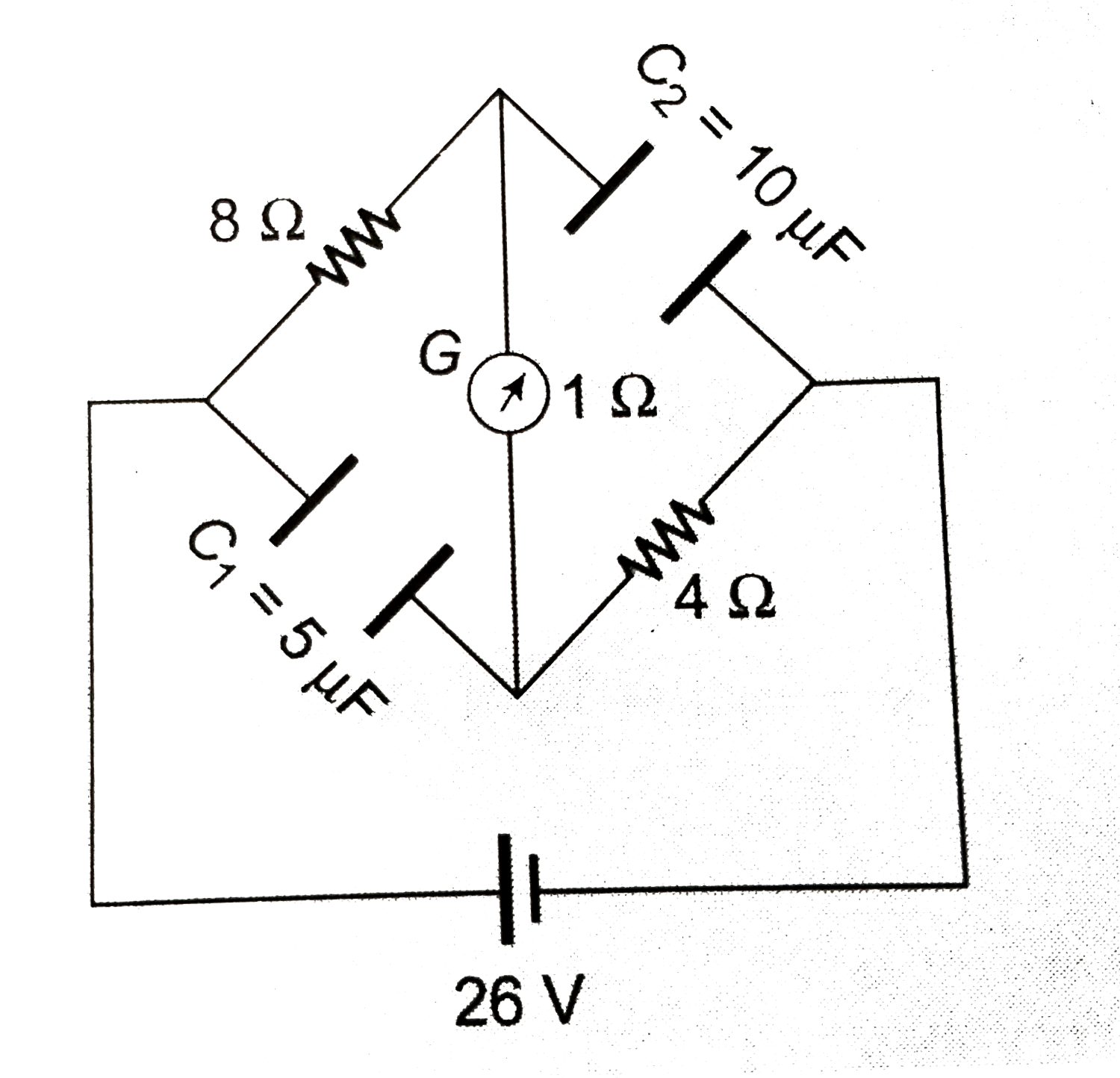
(b) Find charge on capacitor
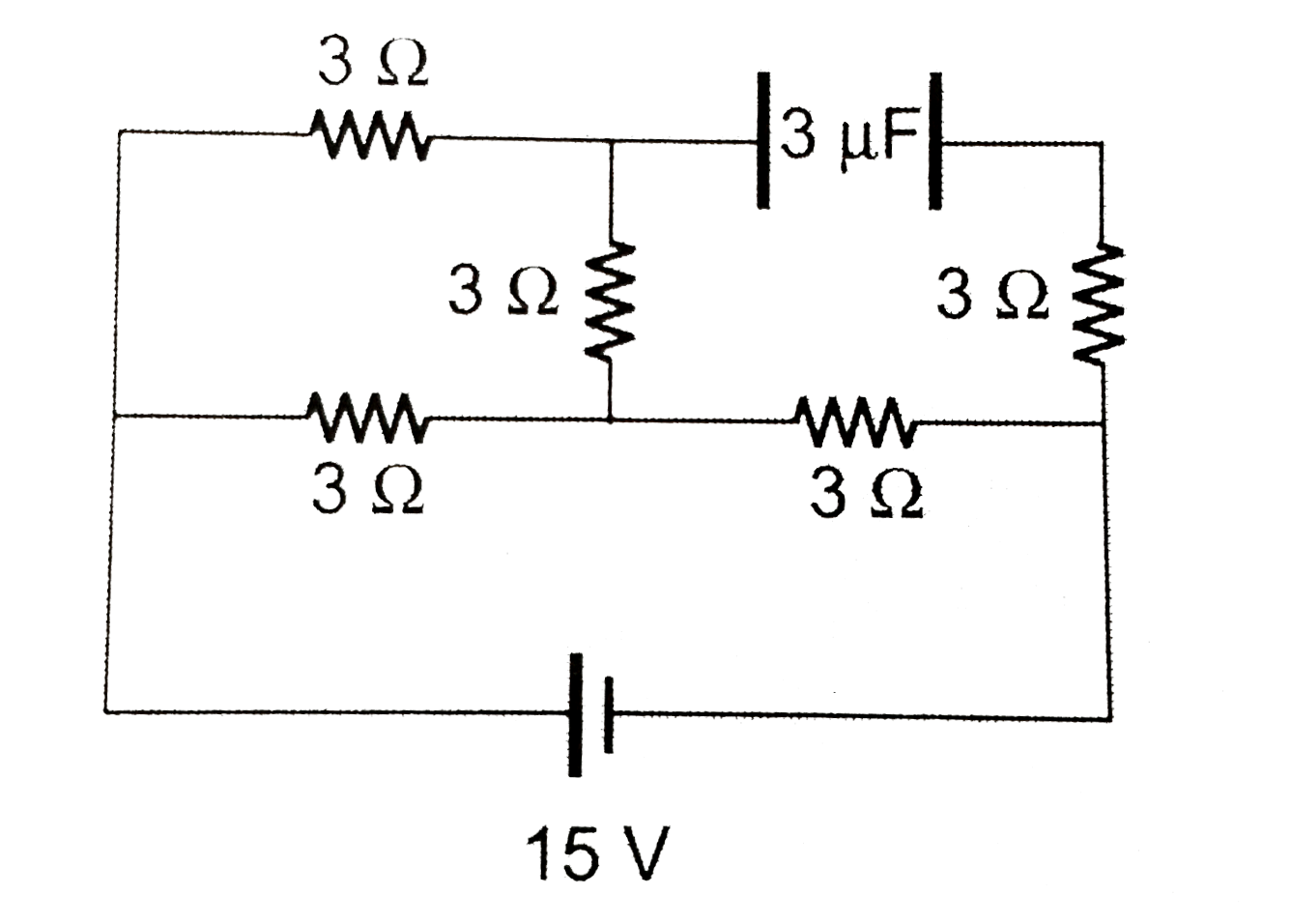
(c ) Find energy stored in capacitor.
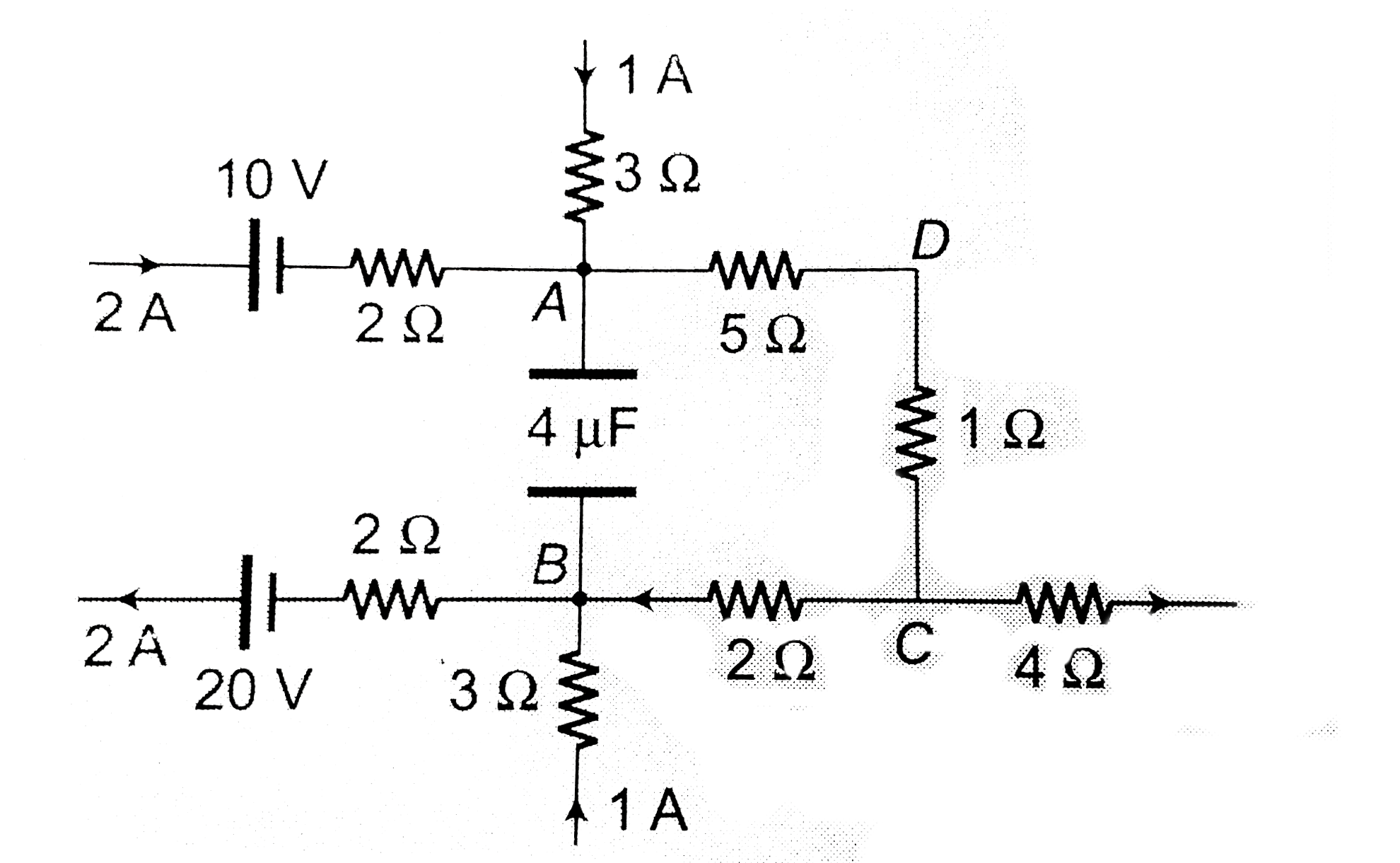
View Solution
At t=0 switch S is closed .
(a) After how much time , charge on capacitor is 50% of maximum charge stored on capacitor.
(b) After how much time, energy stored in capacitor is halt of maximum energy stored in capacitor.
View SolutionWhat happens to the energy stored in a capacitor if after disconnecting the battery, the plates of a charged capacitor are moved farther
View SolutionWhat happens to the energy stored in a capacitor if after disconnecting the battery, the plates of a charged capacitor are moved farther
View SolutionThe energy stored in an electric device known as capacitor is given by U=q22C
where U= energy stored in capacitor
C= capacity of capacitor
q= charge on capacitor
Find the dimensions of capacity of the capacitor
View Solution
