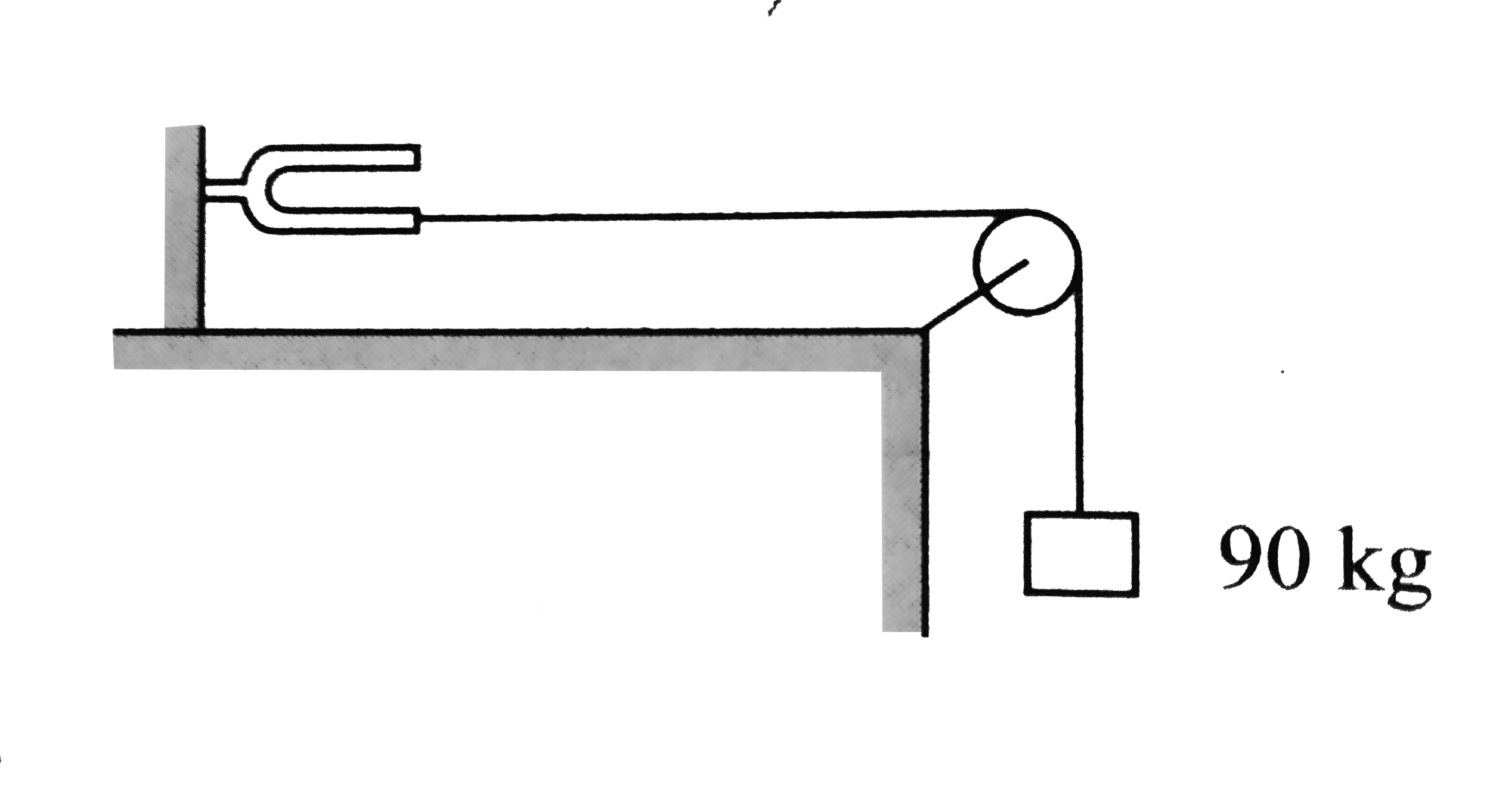
One end of a horizontal rope is attached to a prong of an electrically driven fork that vibrates at . The other end passes over a pulley and supports a mass. The linear mass density of the rope is .
(a) What is the speed of a transverse wave on the rope?
(b) Whatis the wavelength?
How would your answer to parts (a) and (b) change if the mass were increased to ?
The correct Answer is:A, B, C
(a) …(i)
(b)
( c ) From Eq. (i), we can see that . If is double, then will become times. Hence, from the relation will also become times. As remains unchanged.
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Exercise 17.6
6 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Level 1 Assertion And Reason
10 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Exercise 17.4
2 Videos
- VECTORSBOOK - DC PANDEYCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
A body of mass 50 kg is pulled by a rope of length 8 m on a surface by a force of 108N applied at the other end. The force that is acting on 50 kg mass, if the linear density of rope is 0.5 kg/m will be -
View SolutionOne end of 12.0 m long rubber tube with a total mass of 0.9 kg is fastened to a fixed support. A cord attached to the other and passes over a pulley and supports an object with a mass of 5.0 kg. The tube is struck a transverse blow at one end. Find the time required for the pulse to reach the other end
(g=9.8m/s2)
View Solution
Knowledge Check
A uniform rope of mass 0.1 kg and length 2.5 m hangs from ceiling. The speed of transverse wave in the rope at upper end at a point 0.5 m distance from lower end will be
A5 ms−1and2.24ms−1B10 ms−1and3.23ms−1C7.5 ms−1and1.2ms−1DNone of these
A rope of length of 2m is tied between two ends . If the speed of transverse waves propagating in the rope is 4ms−1 and the tension in the rope is 2 N , find the mass of the rope is C.G.S units .
View SolutionA pull of 15 N is applied to a rope attached to a block of mass 7 kg lying on a smooth horizontal surface. The mass of the rope is 0.5 kg. What is the force exerted by the block on the rope?
View SolutionIn an experiment of standing waves, a string 90cm long is attached to the prong of an electrically driven tuning fork that scillates perpendicular to the length of the string at a frequency of 60Hz. The mass of the string is 0.044kg. What tension (in newton) must the string be under (weights are attached to the other end) if it is to oscillate in four loops?
View SolutionOne end of a long string of linear mass density 10−2kgm−1 is connected to an electrically driven tuning fork of frequency 150 Hz. The other end passes over a pulley and is tied to a pan containig a mass of 90 kg. the pulley end absorbs all the incoming energy so that reflected waves from this end have neglidible amplitude , At t =0, the left end (fork end ) of the string is at x=0 has a transverse displacement of 2.5 cm and is moving along positive y-direction. the amplitude of the wave is 5 cm. write down the transverse displacement y (in cetimetres) as function of x(in metres) and t (in seconds ) that describes the wave on the string.
View SolutionOne end of a long string of linear mass dnesity 8.0×10−3kgm−1 is connected to an electrically driven tuning fork of frequency 256 Hz. The other end passes over a pulley and is tied to a pan containing a mass of 90 kg. The pulley end absorbs all the incoming energy so that reflected waves at this end have negligible amplitude. At t=0 the left end (fork end) of the string x=0 has zero transverse displacement (y=0) and is moving along positive y-direction. The amplitude of the wave is 5.0 cm. Write down the transverse displacement y as function of x and t that describest the wave on the string.
View SolutionA uniform rope of length 12m and mass 6kg hangs vertically from a rigid support . A block of mass 2kg is attached to the free end of the rope . A transverse pulse of wavelengths 0.06m is produced at the lower end of the rope . What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionFrom a rigid support, a rope of mass M is hung vertically. To the free end of this rope, a wooden block of mass m is attached. At the lower end of rope, a transverse pulse of wavelength λ is produced. What will be wavelength of pulse when it reaches the top of the rope ?
View Solution
