A uniform rope of length 12m and mass 6kg hangs vertically from a rigid support . A block of mass 2kg is attached to the free end of the rope . A transverse pulse of wavelengths 0.06m is produced at the lower end of the rope . What is the wavelength of the pulse when it reaches the top of the rope?
Tension at the lower end of the rope ,
T1=2g=2×9.8=19.6N
Tension at the upper end of rope ,
Let v1andv2 be the speeds of pulse at the lower and upper end , respectively . So
v=√(T1m),v2=√(T2m)
On dividing , we get
v2v1=√(T2T1)=√(78.419.6)=√4=2
As frequency is independent of medium , therefore if λ1andλ2 are wavelengths at lower and upper ends respectively . Then
v1=nλ1andv2=nλ2
So, λ2λ1=v2v1=2
Therefore , the wavelength of pulse at upper end =2λ.
=2×0.06=0.12m
Similar Questions
A uniform rope of length 12m and mass 6kg hangs vertically from a rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse whwn it reaches the top of the rope?
View SolutionA uniform rope of length 20m and mass 8kg hangs vertically froma rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top os the rope?
View Solution
Knowledge Check
A uniform rope of length 10 m and mass 3 kg hangs vertically from a rigid support. A block of mass 1 kg is attached to the free end of the rople. A transverse pulse of wavelength 0.05 m is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is
A0.10 mB0.12 mC0.16 mD0.18 mA uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1+m2m1B√m1m2C√m1+m2m2D√m2m1
A uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
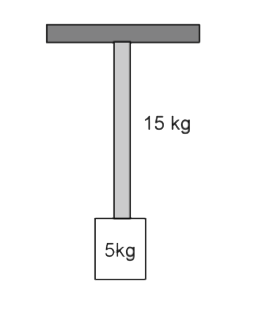
View SolutionA uniform rope of length 10 m and mass 15 kg hangs vertically from a rigid support. A block of mass 5 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.08 m is produced at the lower end of the 3 rope. The wavelength of the pulse when it reaches the top of the rope will be-
View SolutionA uniform rope of elngth l and mass m hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ is produced at the lower end of the rope. The wavelength of the pulse, when it reaches the top of the rope. is aλ. Find a2

View SolutionA uniform rope of elngth l and mass m hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ is produced at the lower end of the rope. The wavelength of the pulse, when it reaches the top of the rope. is aλ. Find a2

View SolutionA uniform rope of mass 6 kg and length 6 m hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.075 m is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is K cm where K is
View Solution
