One metre long tube, closed at both ends, is lying horizontally. A mercurry column of length 0.1 m is filled in its middle and in rest two parts air is filled at atmospheric pressure. What will be the displancement of the mercurry column when the tube is turned to vertical position ? Atmospheric pressure = 0.76 m of Hg.
To solve the problem step by step, we will analyze the situation and apply the principles of physics, particularly the behavior of gases and the concept of pressure.
Step 1: Understand the Initial Conditions
The tube is 1 meter long and is closed at both ends. In the middle, there is a mercury column of length 0.1 m, and the remaining two parts are filled with air at atmospheric pressure (0.76 m of Hg).
Step 2: Determine the Lengths of Air Columns
When the tube is horizontal, the lengths of the air columns on either side of the mercury column can be calculated as follows:
- Total length of the tube = 1 m
- Length of the mercury column = 0.1 m
- Length of air on each side = (1 m - 0.1 m) / 2 = 0.45 m
Step 3: Transition to Vertical Position
When the tube is turned to a vertical position, the mercury column will move due to the change in pressure distribution. The air columns will compress and expand, respectively.
Step 4: Apply Boyle's Law
Since the temperature remains constant, we can apply Boyle's Law, which states that P1V1=P2V2.
- For the upper air column (initially at atmospheric pressure):
- Initial pressure P1=Patm=0.76m of Hg
- Initial volume V1=A⋅(0.45+x)
- For the lower air column (after the mercury column is displaced):
- Final pressure P2 (unknown)
- Final volume V2=A⋅(0.45−x)
Step 5: Set Up the Equations
Using Boyle's Law for both sides:
1. For the upper part:
Patm⋅A⋅(0.45+x)=P1⋅A⋅0.45
Simplifying gives:
0.76⋅(0.45+x)=P1⋅0.45
2. For the lower part:
P2⋅A⋅(0.45−x)=Patm⋅A⋅0.45
Simplifying gives:
P2⋅(0.45−x)=0.76⋅0.45
Step 6: Relate Pressures
The pressure in the lower part can be expressed as:
P2=Patm+hHg
Where hHg is the height of the mercury column displaced.
Step 7: Solve for Displacement
By substituting P1 and P2 into the equations derived from Boyle's Law, we can set up a quadratic equation in terms of x (the displacement of the mercury column).
Step 8: Calculate the Displacement
After solving the quadratic equation, we find:
x≈0.029m or 2.9cm
Conclusion
The displacement of the mercury column when the tube is turned to a vertical position is approximately 2.9 cm.
---
The tube is 1 meter long and is closed at both ends. In the middle, there is a mercury column of length 0.1 m, and the remaining two parts are filled with air at atmospheric pressure (0.76 m of Hg).
When the tube is horizontal, the lengths of the air columns on either side of the mercury column can be calculated as follows:
- Total length of the tube = 1 m
- Length of the mercury column = 0.1 m
- Length of air on each side = (1 m - 0.1 m) / 2 = 0.45 m
When the tube is turned to a vertical position, the mercury column will move due to the change in pressure distribution. The air columns will compress and expand, respectively.
Since the temperature remains constant, we can apply Boyle's Law, which states that P1V1=P2V2.
- Initial pressure P1=Patm=0.76m of Hg
- Initial volume V1=A⋅(0.45+x)
- For the lower air column (after the mercury column is displaced):
- Final pressure P2 (unknown)
- Final volume V2=A⋅(0.45−x)
Using Boyle's Law for both sides:
1. For the upper part:
Patm⋅A⋅(0.45+x)=P1⋅A⋅0.45
Simplifying gives:
0.76⋅(0.45+x)=P1⋅0.45
P2⋅A⋅(0.45−x)=Patm⋅A⋅0.45
Simplifying gives:
P2⋅(0.45−x)=0.76⋅0.45
The pressure in the lower part can be expressed as:
P2=Patm+hHg
Where hHg is the height of the mercury column displaced.
By substituting P1 and P2 into the equations derived from Boyle's Law, we can set up a quadratic equation in terms of x (the displacement of the mercury column).
After solving the quadratic equation, we find:
x≈0.029m or 2.9cm
The displacement of the mercury column when the tube is turned to a vertical position is approximately 2.9 cm.
Topper's Solved these Questions
- BEHAVIOUR OF PERFECT GAS & KINETIC THEORYBOOK - PRADEEPCHAPTER - BEHAVIOUR OF PERFECT GAS & KINETIC THEORYEXERCISE - Curiosity Question
2 Videos
- BEHAVIOUR OF PERFECT GAS & KINETIC THEORYBOOK - PRADEEPCHAPTER - BEHAVIOUR OF PERFECT GAS & KINETIC THEORYEXERCISE - Solved Examples
43 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions
19 Videos
Similar Questions
A horizontal tube of 1m is closed at both ends. A mercury column of 20 cm is contained in the middle of the tube and two equal lengths of this tube contain air at an atmospheric pressure of 76cm. The tube is now placed vertically. Calculate the distance through which the mercury column will be displaced at a constant temperature.
View SolutionA uniform tube closed at aone end, contains a pellet of mercury 10cm long. When the tube is lept vertically with the closed-end upward, the length of the air column trapped is 20cm. Find the length of the air column trapped when the tube is inverted so that the closed-end goes down. Atmospheric pressure=75cm of mercury.
View Solution
Knowledge Check
A small capillry tube of length 100cm closed at one end is kept horizontally and a mercury column of length 10cm is exactly in the middle. If the atmospheric pressure is 1 atm then identify the correct option(s).
AThe pressure of "air trapped" in the orgina: horizontal position is 750mm of Hg.BThe length of the air trapped when the tube is held vertically with open end upwards is 45×7686cm.CThe length of the air trapped when the tube is held vertically with open end downwards is 45×7666cm.DNo air will be trapped when it is held vertically with open end downward.What will be the length of mercury column in a barometer tube when the atmospheric pressure is x cm of mercury and the tube is inclined at an angle ϕ with the vertical direction ?
AxsinϕBxcosϕCxtanϕDxAn open glass tube is immersed in mercury in such a way that a lenth of 8cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46cm. What will be length of the air column above mercury in the tube now?
(Atmosphere pressure =76cm of Hg)A38 cmB6 cmC16 cmD22 cm
Both limbs of a 'U' tube are of equal length. One of the limbs is sealed and contains a column of 28 cm of air at atmospheric pressure. The air is separated from the atmosphere by mercury. What will be the height of air in the sealed limb, if the other limb is now filled to the top with mercury ? Atmospheric pressure is 76 cm of mercury.
View SolutionA column of mercury of 10cm length is contained in the middle of a narrow horizontal 1m long tube which is closed at both the ends. Both the halves of the tube contain air at a pressure of 76 cm of mercury. By what distance will the column of mercury be displaced if the tube is held vertically?
View SolutionA thin tube, sealed at both ends, is 100cm long. If lies horizontally, the middle 10cm containing mercury and the two equal containing air at stan-dard atmospheric pressure. If the tube is now turned to a verical position, by what amount will the mercury be displaced ?
View SolutionIn the given figure, atmospheric pressure p0=1 atm and mercury column length is 9 cm. Pressure p of the gas enclosed in the tube is
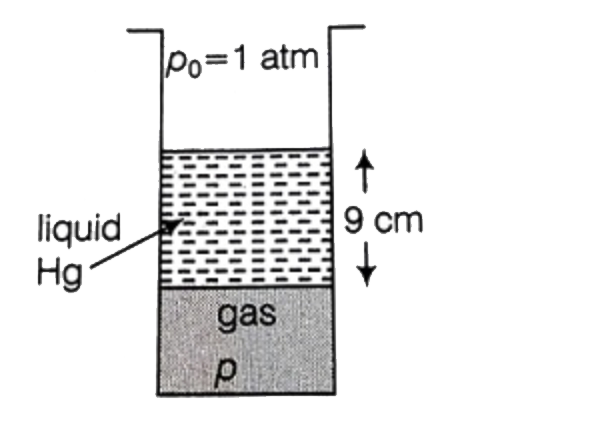
View SolutionAn open glass tube is immersed in mercury in such a way that a length of 8 cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46 cm. what will be length of the air column above mercury in the above now ?
(Atmospheric pressure = 76 cm of Hg)
View Solution
