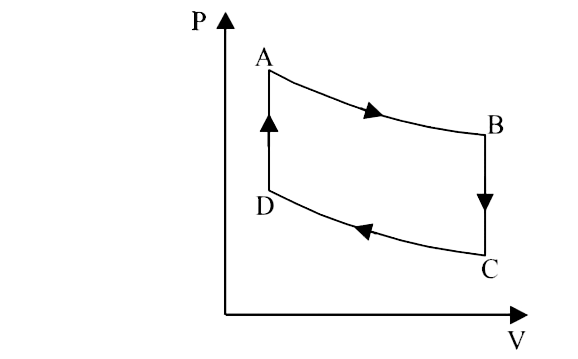
One mole of a monoatomic ideal gas is taken through the cycle shown in Fig:
A→B: adiabatic expansion
B→C: cooling at constant volume
C→D: adiabatic compression
D→A: heating at constant volume
![One mole of a monoatomic ideal gas is taken through the cycle shown in Fig: AtoB: adiabatic expansion BtoC: cooling at constant volume CtoD: adiabatic compression DtoA: heating at constant volume The pressure and temperature at A,B,etc. are denoted by PA, TA,PB,TBetc. respectively. Given that TA=1000K, PB=(2//3)PA and PC=(1//3)PA, calculate the following quantities: (i) The work done by the gas in the process AtoB (ii) The heat lost by the gas in the process BtoC. (iii) The temperature TD. [Given :(2//3)^(2//5)=0.85]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/JMA_HTG_C09_118_Q01.png)
The pressure and temperature at A,B,etc. are denoted by PA,TA,PB,TBetc. respectively. Given that TA=1000K, PB=(2/3)PAandPC=(1/3)PA, calculate the following quantities:
(i) The work done by the gas in the process A→B
(ii) The heat lost by the gas in the process B→C.
(iii) The temperature TD. [Given :(2/3)2/5=0.85]
The correct Answer is:A, B
Given TA=1000K
PB=23PA
PC=13PA
(i) WAB(adiabaticexpansion)
WAB=nR[TA−TB]γ−1
Here, n=1,R=8.32Jmol−1k−1,TA=1000K
γ=53(Formonoa→micgas)
To find TB, we use
TγAPγ−1A=TγBPγ−1B⇒(PAPB)γ−1=(TATB)γ....(i)
⇒TB=TA⎡⎢⎣(PAPB)γ−1γ=1000[32]1−5/35/3=850K
∴WAB=1×8.31[1000−850]5/3−1=1870J
(ii) Heat Lost B→C
Q=nCvΔT=nCv(TB−TC)
Here, n=1,Cv=32R(Formonoa→micgas),
TB=850K
To find TC, we use PBTB=PCTC (volume constant)
PCPB=TCTB......(ii)
⇒TC=PCPB×(TB)=12×850=425K⎡⎣∵PCPA=13PA23PA=12⎤⎦
∴Q=1×32×8.31[425−850]=−5298J
(ii) Temperature TD : C to D is adiabatic compression
(PCPD)γ−1=(TCTD)γ......(iii)
D to A is isochoric process PDTD=PATA
⇒PAPD=TATD......(iv)
Multiplying (i) and (iii)
(PCPA(PDPB)γ−1=(TCTD×TATB)γ......(v)
Multiplying (ii) and (iv)
((P_AP_C)/(P_BP_D)=((T_CT_A)/(T_BT_D)).....(vi)<br>From(v)and(vi)<br>((T_CT_A)/(T_BT_D))^(gamma-1)=((T_CT_A)/(T_BT_D))^(gamma) rArr (T_AT_C)/(T_BT_D)=1<br>rArrT_D=(T_AT_C)/(T_B)=(1000xx425)/850=500K
Topper's Solved these Questions
Similar Questions
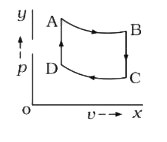
One mole of a monatomic ideal gas is taken through the cycle shown in the figure: A→B adiabatic expansion

B→C: cooling at constant volume
C→D adiabatic compression D→C: heating at constant volume.
The pressure and temperature at PA,PB etc. TA,TB etc. are denoted etc. respectively. Given that
TA=1000K,PB=(2/3)PA and PC=(1/3)PA
Calculate the following quantities.
The work done by the gas in process A→B (in J)
View SolutionOne mole of a monatomic ideal gas is taken through the cycle shown in the figure: A→B adiabatic expansion
B→C: cooling at constant volume
C→D adiabatic compression D→C: heating at constant volume.
The pressure and temperature at PA,PB etc. TA,TB etc. are denoted etc. respectively. Given that
TA=1000K,PB=(2/3)PA and PC=(t/3)PA
Calculate the following quantities.
The heat lost by the gas in process B→C (in J)
View Solution
Knowledge Check
One mole of a monatomic ideal gas is taken through the cycle shown in figure
 The pressures and temperatures at A,B etc, are denoted by PA,TA,PB,TBetc respectively.Given TA=1000K,PB=(2/3)PAandPC=(1/3)PA Then choose the incorrect option. AThe work done by the gas in the process A→B is 1869.75JBThe heat lost by the gas sin the process B→C is -5297.25JCTemperature TD is 500KDWork done from B→C is 40J
The pressures and temperatures at A,B etc, are denoted by PA,TA,PB,TBetc respectively.Given TA=1000K,PB=(2/3)PAandPC=(1/3)PA Then choose the incorrect option. AThe work done by the gas in the process A→B is 1869.75JBThe heat lost by the gas sin the process B→C is -5297.25JCTemperature TD is 500KDWork done from B→C is 40JOne mole of a monoatomic ideal gas is taken through the cycle ABCDA as shown in the figure.
TA=1000K and 2pA=3pB=6pC

[Assume(23)0.4=0.85 and R=253JK−1mol−1]
The temperature at B isA350KB1175KC850KD577KOne mole of an ideal monoatomic gas is taken through a polytropic process P2T = constant. The heat required to increase the temperature of the gas by ΔT is
ARΔTB3RΔTC5RΔTD6RΔT
On mole of a monoatomic ideal gas is taken through the cycle shown in figure .
A→B : Adiabatic expansion B→C : Cooling at constant volume
C→D: Adiabatic compression D→A : Heating at constant volume
The pressure and temperature at A,B, etc, are denoted by PA,TA,PB,TB etc., respectively . Given that TA=1000K,PB=(23)PAandPC=(13)PA. Calculate the following quantities: (i) The work done by the gas in the processs A→B (ii) The heat lost by the gas in the process B→C (iii) The temperature TD.(Given:(23)2/5=0.85)
_E01_271_Q01.png)
View SolutionOne mole of a monoatomic ideal gas is taken through the cycle shown in figure.
A→B Adiabatic expansion
B→C Cooling at constant volume
C→D Adiabatic compression.
D→A Heating at constant volume
The pressure and temperature at A,B etc., are denoted by pA,TA,pB,TB etc. respectively.
Given, TA=1000K, pB=(23)pA and pC=(13)pA. Calculate
(a) the work done by the gas in the process A→B
(b) the heat lost by the gas in the process B→C
Given, (23)0.4=0.85 and R=8.31J/mol−K
View SolutionOne mole of a monatomic ideal gas is taken through the cycle shown in the figure: A→B adiabatic expansion
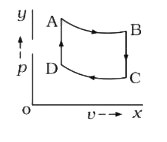
B→C: cooling at constant volume
C→D adiabatic compression D→A: heating at constant volume.
The pressure and temperature at PA,PB etc. TA,TB etc. are denoted etc. respectively. Given that
TA=1000K,PB=(2/3)PA and PC=(1/3)PA
Calculate the following quantities.
The temperature TD (in K) [Given : (25)2/5=0.85 ]
View SolutionOne mole of a monoatomic ideal gas is taken through the cycle ABCDA as shown in the figure.
TA=1000K and 2pA=3pB=6pC

[Assume(23)0.4=0.85 and R=253JK−1mol−1]
Heat lost by the gas in the process B→C is
View SolutionOne mole of a monoatomic ideal gas is taken through the cycle ABCDA as shown in the figure.
TA=1000K and 2pA=3pB=6pC

[Assume(23)0.4=0.85 and R=253JK−1mol−1]
Work done by the gas in the process A→B is
View Solution
