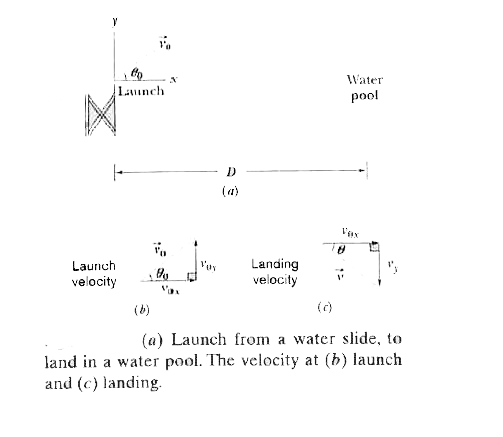
One of the most dramatic videos on the web (but entirely fictitious) supposedly shows a man sliding along a long water slide and then being launched into the air to land in a water pool. Let's attach some reasonable numbers to such a flight to calculate the velocity with which the man would have hit the water. Figure a indicates the launch and landing sites and includes a superimposed coordinate system with its origin conveniently located at the launch site. From the video we take the horizontal flight distance as D = 20.0 m, the flight time as t = 2.50 s, and the launch angle as $θ_{0} = {40.0}^{\circ}$ . Find the magnitude of the velocity at launch and at landing.

Text Solution

Verified by Experts

(1) For projectile motion, we can apply the equations for constant acceleration along the horizontal and vertical axes separately. (2) Throughout the flight, the Vertical acceleration is $a_{y} = - g = - 9.8 m / s^{2}$ and the horizontal acceleration is $a_{x}$ = 0.
In most projectile problems, the initial challenge is to figure out where to start. There is nothing wrong with trying out various equations, to see if we can somehow get to the velocities. But here is a clue. Because we are going to apply the constant acceleration equations separately to the x and y motions, we should find the horizontal and vertical components of the velocities at launch and at landing. For each site, we can then combine the velocity components to get the velocity. Because we know the horizontal displacement D=20.0 m, let.s start with the horizontal motion. Since $a_{x}$ = 0, we know that the horizontal velocity component $v_{x}$ is constant during the flight and thus is always equal to the horizontal component $v_{0}$ , at launch . we can relate that component , the displacement $x - x_{0}$ and the flight time t = 2.50 s with eq.
$x - x_{0} = v_{0} t + \frac{1}{2} a t^{2}$
substituting $a_{x} = 0$ , this becomes eq. with $x - x_{0} = D$ we the write
$20 m = v_{0} (2.0 s) + \frac{1}{2} (0) {(2.50 s)}^{2}$
$v_{0 x} = 8.00 m \frac{/}{s}$
That is a component of the launch velocity , but we need the magnitude of the full vector ,
where the component form the legs of a right triangle and the vector forms the hypotenuse . we can them apply a trig definition to find the magnitude of the full velocity at launch :
${cos θ}_{0} = \frac{v_{0 x}}{v_{0}}$
$v_{0} = \frac{v_{0 x}}{{cos θ}_{0}} = \frac{8.00 m / s}{{cos 40}^{\circ}}$
= 10.44 m//s ~~ 10.4 m//s

Updated on:21/07/2023