Passage VI Two particles collide when they are at same position at same time.
Displacement time equation of two particles moving along x-axis are x1=(8+3sinωt)m
and x2=(4cosωt)m
Here, ω=πrads
The two particles will collide after time t=...................s.
1
2
4
None of the above.
The correct Answer is:D
Step by step video, text & image solution for Passage VI Two particles collide when they are at same position at same time. Displacement time equation of two particles moving along x-axis are x_(1)=(8+3sinomegat)m and x_(2)=(4cosomegat)m Here, omega=pirad/s The two particles will collide after time t=...................s. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Matrix matching type questions
13 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Integer type questions
14 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEYCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - More than one option is correct
50 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEYCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEYCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
The displacement -time equation of a particle moving along x -axis is x=t33−t22+20The acceleration -velocity equation of the particle is a2=KV+1 Then the value of K is
View SolutionThe position of a particle moving along x-axis varies eith time t as x=4t−t2+1. Find the time interval(s) during which the particle is moving along positive x-direction.
View Solution
Knowledge Check
Passage VI Two particles collide when they are at same position at same time.
The two particles are at minimum distance after time t=…………..s.A1B2C3DAll of theseFigure (i) and (i) show the displacement-time graphs of two particles moving along the x-axis. We can say that
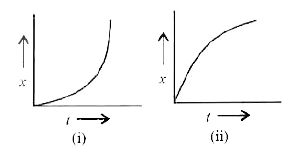 Aboth the particles are having a uniformly retarded motionBboth the particles are having a uniformly acceleraled motionCParticle (1) is having a uniformly accelerated motion while particle (i) is having a uniformly retarded motionDParticle (i) is having a uniformly retarded motion while particle (ii) is having a uniformly accelerated motion
Aboth the particles are having a uniformly retarded motionBboth the particles are having a uniformly acceleraled motionCParticle (1) is having a uniformly accelerated motion while particle (i) is having a uniformly retarded motionDParticle (i) is having a uniformly retarded motion while particle (ii) is having a uniformly accelerated motionDisplacement (s)-time (t) graphs of two particles moving in a straight line along the X-axis are shown below: It may be stated that the
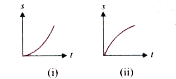 Aparticle (i) has uniform accelerationBparticle (i) has non-uniform accelerationCparticle (ii) has uniform motionDparticle (ii) has a retarded motion
Aparticle (i) has uniform accelerationBparticle (i) has non-uniform accelerationCparticle (ii) has uniform motionDparticle (ii) has a retarded motion
The position of a particle moving along x-axis varies with time t according to equation x=√3sinωt−cosωt where ω is constants. Find the refion in which the particle is confined.
View SolutionThe position of particle moving along the x-axis veries with time t as x=6t−t2+4. Find the time-interval during which the particle is moving along the positive x-direction.
View SolutionThe displacement of a particle moving along x-axis with respect to time t is x=at+bt2−ct3 The dimensions of care
View SolutionThe position x of particle moving along x-axis varies with time t as x=Asin(ωt) where A and ω are positive constants. The acceleration a of particle varies with its position (x) as
View SolutionThe position (x) of a particle moving along x - axis veries with time (t) as shown in figure. The average acceleration of particle in time interval t = 0 to t = 8 s is

View Solution
