P,Q,R and S are the points in a vertical line. It is given that PQ=QR=RS. A particle is released from rest from the point P. particle takes time $t_{P Q}, t_{Q R}$ and $t_{R S}$ to cover three equal distance, respectively. Find ratio $t_{P Q} : t_{Q R} : t_{R S}$

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem, we need to find the ratio of the times taken by a particle to cover three equal distances (PQ, QR, and RS) when released from rest. Let's denote the distance between each pair of points (PQ, QR, RS) as $x$ .
1. Understanding the Motion:
The particle is released from rest, which means its initial velocity $u = 0$ . The distance covered in each segment is equal, $P Q = Q R = R S = x$ .
2. Using the Equation of Motion:
The equation of motion for an object under free fall (with acceleration due to gravity $g$ ) is given by:
$s = u t + \frac{1}{2} g t^{2}$
Since $u = 0$ , this simplifies to:
$s = \frac{1}{2} g t^{2}$
3. Finding Time for Each Segment:
- For the first segment $P Q$ :
$x = \frac{1}{2} g t_{P Q}^{2}$
Rearranging gives:
$t_{P Q}^{2} = \frac{2 x}{g} \Rightarrow t_{P Q} = \sqrt{\frac{2 x}{g}}$
- For the second segment $Q R$ :
The total distance covered from $P$ to $R$ is $2 x$ . The time taken is $t_{P Q} + t_{Q R}$ :
$2 x = \frac{1}{2} g (t_{P Q} + t_{Q R})^{2}$
Expanding this gives:
$2 x = \frac{1}{2} g (t_{P Q}^{2} + 2 t_{P Q} t_{Q R} + t_{Q R}^{2})$
Substituting $t_{P Q}^{2} = \frac{2 x}{g}$ :
$2 x = \frac{1}{2} g (\frac{2 x}{g} + 2 t_{P Q} t_{Q R} + t_{Q R}^{2})$
Simplifying gives:
$4 x = 2 x + 2 g t_{P Q} t_{Q R} + g t_{Q R}^{2}$
Rearranging leads to:
$2 x = 2 g t_{P Q} t_{Q R} + g t_{Q R}^{2}$
Dividing through by $g$ :
$\frac{2 x}{g} = 2 t_{P Q} t_{Q R} + t_{Q R}^{2}$
This is a quadratic equation in $t_{Q R}$ .
- For the third segment $R S$ :
The total distance covered from $P$ to $S$ is $3 x$ :
$3 x = \frac{1}{2} g (t_{P Q} + t_{Q R} + t_{R S})^{2}$
Following similar steps as above will yield $t_{R S}$ .
4. Finding the Ratios:
After calculating $t_{P Q}, t_{Q R},$ and $t_{R S}$ , we can express them in terms of $\sqrt{\frac{2 x}{g}}$ and find the ratio:
$t_{P Q} : t_{Q R} : t_{R S} = 1 : (\sqrt{2} - 1) : (\sqrt{3} - \sqrt{2})$
Final Ratio:
Thus, the final ratio of the times taken is:
$t_{P Q} : t_{Q R} : t_{R S} = 1 : (\sqrt{2} - 1) : (\sqrt{3} - \sqrt{2})$

A particle is released from rest from a tower of height 3h. The ratio of time intervals for fall of equal height h i.e. $t_{1} : t_{2} : t_{3}$ is :
A $\sqrt{3} : \sqrt{2} : 1$
B $3 : 2 : 1$
C $9 : 4 : 1$
D $1 : (\sqrt{2} - 1) : (\sqrt{3} - \sqrt{2})$
A point particle of mass m, moves long the uniformly rough track PQR as shown in figure. The coefficient of friction, between the particle and the rough track equals $μ$ . The particle is released, from rest from the point P and it comes to rest at a point R. The energies, lost by the ball, over the parts, PQ and QR, of the track, are equal to each other, and no energy is lost when particle changes direction from PQ to QR.
The value of the coefficient of friction $μ$ and the distance x $(= Q R)$ , are, respectively close to:

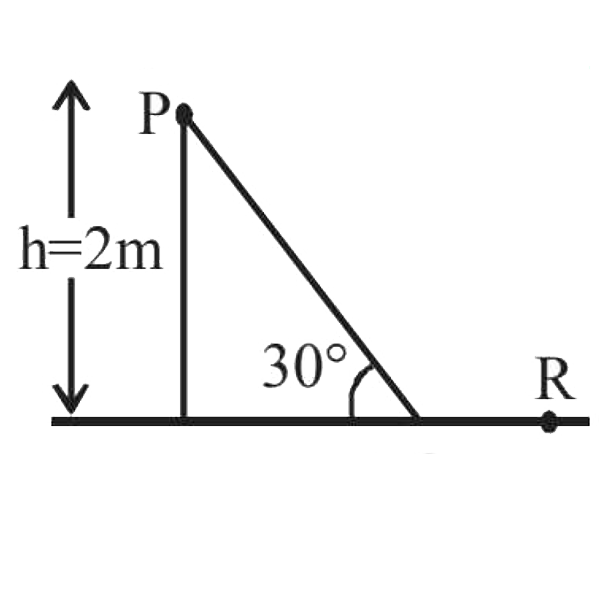A(a) 0.29 and 3.5m
B(b) 0.29 and 6.5m
C(c) 0.2 and 6.5m
D(d) 0.2 and 3.5m
A particle starts from rest from point P and follow a path PQ on the three smooth surfaces as shown in figures. If time taken by the particle in three cases are $t_{1}, t_{2}$ and $t_{3}$ then

A $t_{1} > t_{2} > t_{3}$
B $t_{3} > t_{2} > t_{1}$
C $t_{3} > t_{1} > t_{3}$
D $t_{1} = t_{2} = t_{3}$