Question

Prove that a bar magnet and a solenoid produce similar fields.

Explanation

Bar magnet produce similar field of Solenoid :
(1) We know that the current loop acts as a magnetic dipole. According to Ampere's all magnetic phenomena can be explained in terms of circulating currents.
(2) Cutting a bar magnet is like a solenoid. We get two similar solenoids with weaker magnetic proeperties.
(3) The magnetic field lines remain continuous, emerging from one face of solenoid and entering into other face of solenoid.
(4) If we were to move a small compass needle in the neighbourhood of a bar magnet and a current carrying solenoid, we would find that the deflections of the needle are similar in both cases as shown in diagrams.


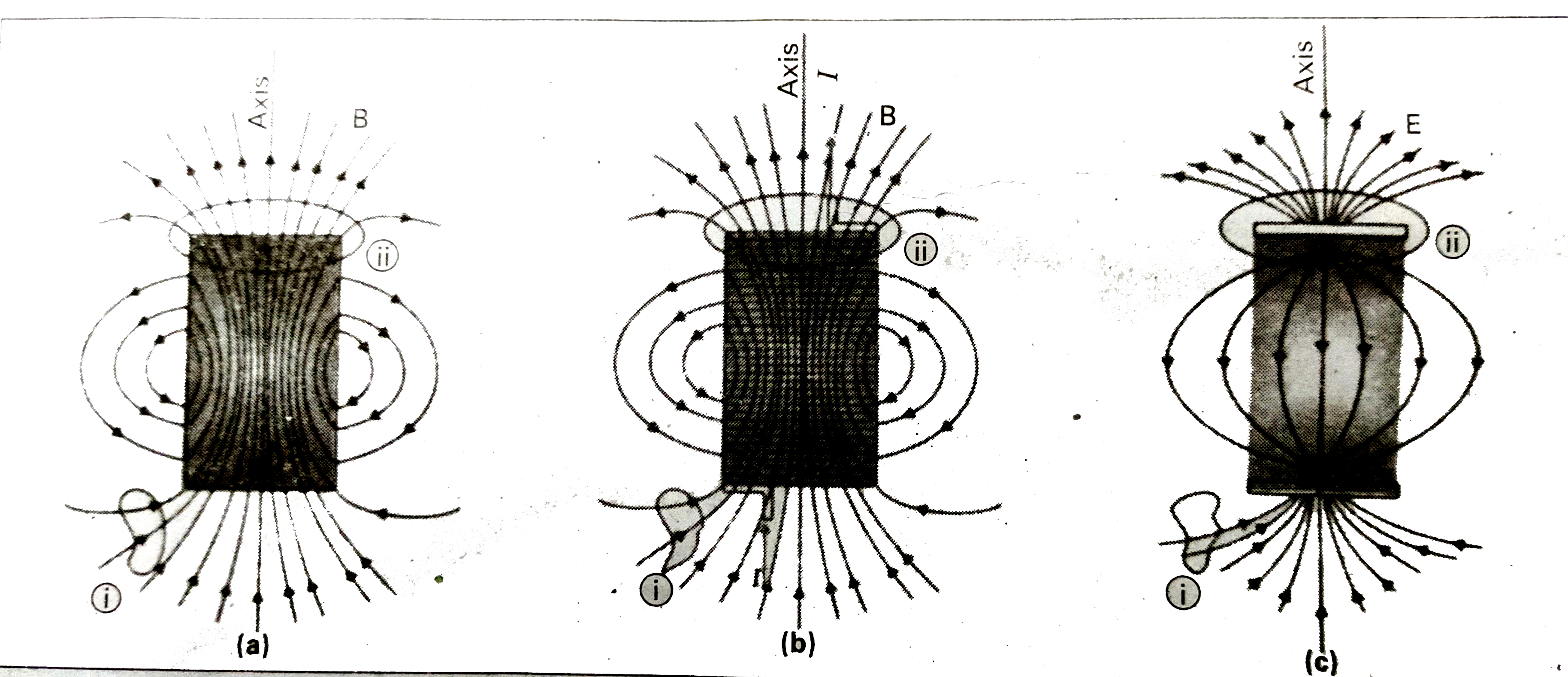
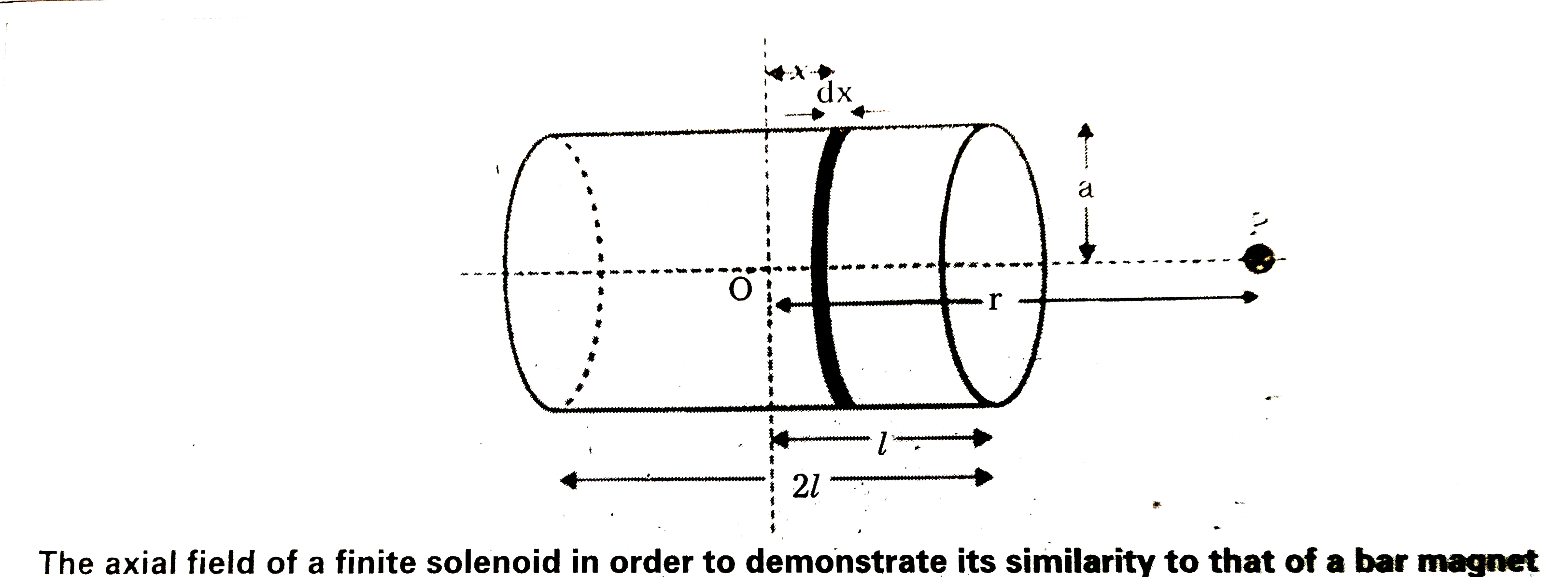
The magnetic field at point P due to bar magnet in the form of solenoid is `B = (mu_(0))/(4pi) (2m)/(r^(3))`
(6) The total magnetic field, at a point P due to solenoid is given by
`B = (mu_(0)n I)/(2)(a^(2))/(r^(3))(2l) = (mu_(0))/(4pi)(2n(2l)I pi a^(2))/(r^(3))`
(7) The magnitude of the magnetic moment of the solenoid is, `m = n(2l) I (pi a^(2))`.
`:. B = (mu_(0))/(4pi) (2m)/(r^(3))`
Therefore, magnetic moment of a bar magnet is equal to magnetic moment of an equivalent solenoid that produces the same magnetic field.