Prove that in any triangle ABC, λ−−→AB+μ−−→AC=(λ+μ)−−→AD where D is a point on BC such that BD:DC=μ:λ.Hence prove that if resultant of −−→AB, 2−−→AC and 3−−→BC cuts BC and CA at E and F respectively, 3EC=BC and 4CF=AC and the resultant is 12 −−→FE
Text Solution
The correct Answer is:NA
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
OAB is a given triangle such that −−→OA=→a,−−→OB=→b. Also C is a point on −−→AB such that −−→AB=2−−→BC. What is −−→AC equal to ?
A12(→b−→a)B12(→b+→a)C32(→a−→b)D32(→b−→a)BC is divided into four equal parts by P,Q and R.The resultant of −−→AB and 3−−→AC is
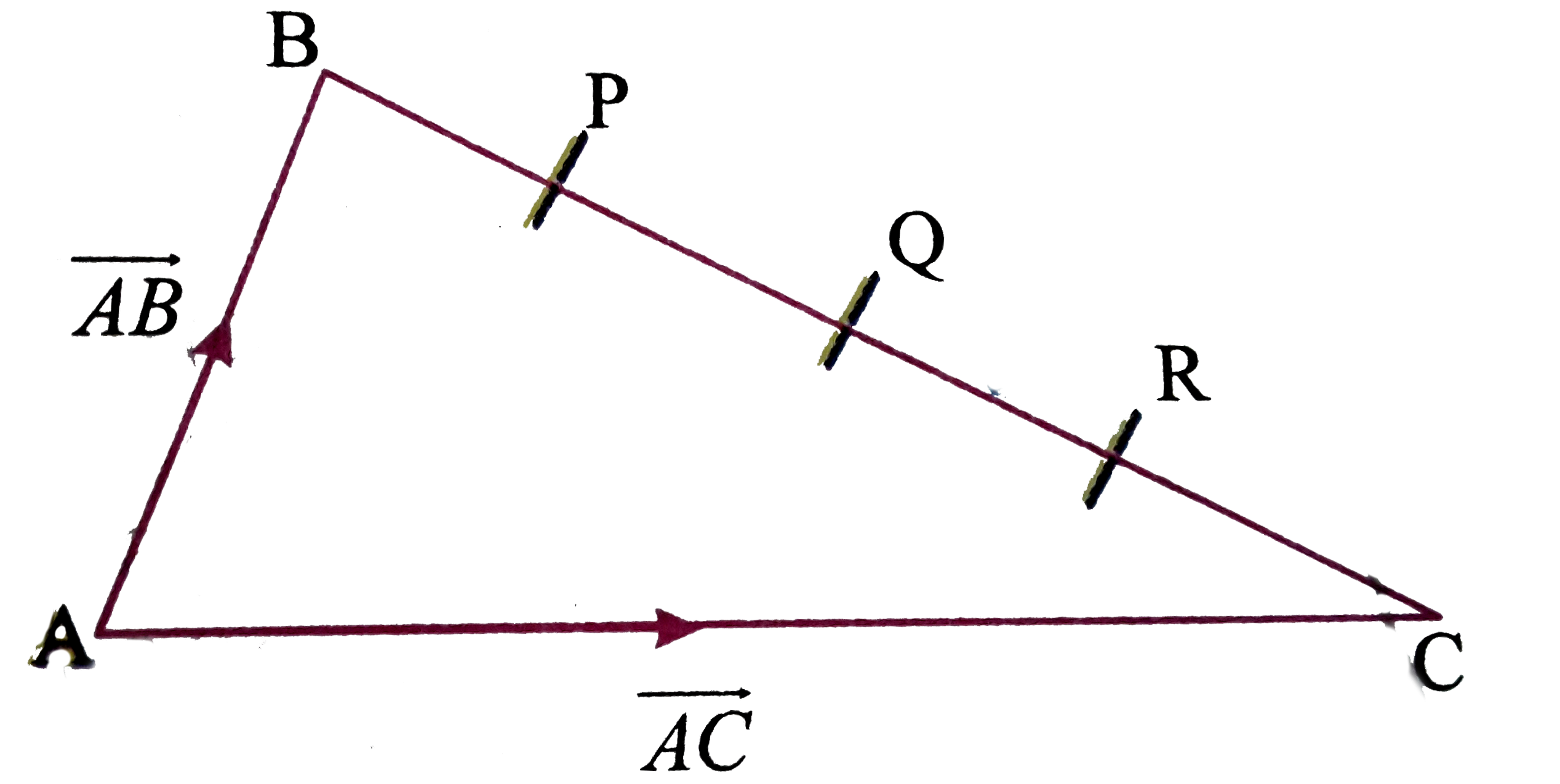 A−−→ARB4−−→ARC4−−→APD−−→PQ
A−−→ARB4−−→ARC4−−→APD−−→PQLet →a,→b,→c represent respectively −−→BC,−−→CAand−−→AB where ABC is a triangle , Then ,
A→a+→b=→cB→b+→c=→aC→a×→b=→b×→c=→c×→aD[→a→b→c]=[→b→c→a]=[→c→a→b]≠0
In a parallelogram ABCD. Prove that −−→AC+−−→BD=2−−→BC
View SolutionLet →a,→b,→c form sides BC,CA and AB respectively of a triangle ABC then
View SolutionIn a triangle ABC, if ∣∣∣−−→BC∣∣∣=8,∣∣∣−−→CA∣∣∣=7,∣∣∣−−→AB∣∣∣=10, then the projection of the −−→AB on −−→AC is equal to :
View SolutionIf D is the mid point of side BC of a triangle ABC such that →AB+→AC=λ →AD, write the value of λ.
View SolutionLet →a,→b,→c represent respectively −−→BC,−−→CAand−−→AB where ABC is a triangle , Then ,
View Solution
