Prove the following formula when refraction takes place at a convex spherical refracting surface and source of light lies in the rarer medium and image formed is real $\frac{μ_{2}}{v} - \frac{μ_{1}}{u} = \frac{μ_{2} - μ_{1}}{R}$ Where the terms have their usual meanings.Solution in Punjabi

Video Solution

Text Solution

Verified by Experts

Assumptions: (i) The object is a point object and lying on the principal axis.
(ii) The aperature of refracting spherical surface is small.
(iii) The incident ray, refracted ray and normal make smaller angles with principal axis.
Cartesian sign conventions,
(i) All the distances are measured from the pole of the spherical surface.
(ii) The distances measured along the incident ray are taken as positive and distances measured opposites to direction of incident ray are taken as negative.
(iii) The distances measured in vertically upward direction from principal axis are taken as positive and in vertically downward direction from principal axis are taken as negative.
Consider a convex spherical refracting surface of refractive index $μ_{2}$ . Let it be placed in a rarer medium of refractive index $μ_{1} (μ_{2} > μ_{1})$ .
A point object O lies on the principal axis at a distance u from the pole of the convex refracting surface in rarer medium. A ray of light from O incident the convex surface at A. AC is the normal to the convex surface. After refraction at A, the ray enters the dense medium and bends towards the normal. The refracted ray meets the principal axis at I which is the real image of the object O. The distance of the image I from the pole of the convex surface is V.

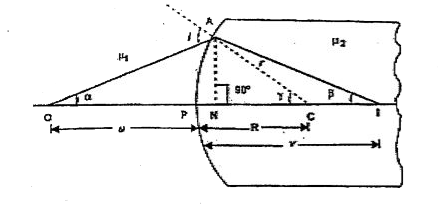
d Let $α, β$ and $γ$ be the angles made by the incident ray, refracted ray and the normal respectively with the principal axis. Draw AN perpendicular on the principal axis.
From $Δ A O E i = α + γ$ .............i
Since $α, β$ and $γ$ are small angles (assumption) so they can be replaced by their tangents,
Hence eqn (i) becomes
$i = tan α + tan γ$ ................ii
From $Δ A I C γ = r + β or r = γ - β$ ...........iii
Again $γ$ and $β s$ are small (assumption) so they can be replaced by their tangents. HEnce eq (iii) becomes
$r = tan γ - tan β$ ............iv
Determination of $tan α, tan β$ and $tan γ$
From rt. $∠ d Δ A N O tan α = \frac{A N}{N O}$ ............v
From rt. $∠ d Δ A N C, tan γ = \frac{A N}{N C}$ ..........vi
From rt. $∠ d Δ A N I tan β = \frac{A N}{N I}$ .............vii
Using eqns v to vi we can writes eqn (iii) and (iv) as
$i = \frac{A N}{N O} + \frac{A N}{N C}$ ......................viii and $r = \frac{A N}{N C} - \frac{A N}{N I}$ ..........ix
Since aperture of the spherical surface is small (assumption) so point N lies very close to point P.
$∴ N O = P O, N C ≅ P C$ and $N I ≅ P I$
Hnece eqn viii and ix can be written as
$i = \frac{A N}{P O} + \frac{A N}{P C}$ .........x
and $r = \frac{A N}{P C} - \frac{A N}{P I}$ ...........xi
Now according to Snell.s law
$\frac{sin i}{sin r} = \frac{μ_{2}}{μ_{1}}$ or $μ_{1} sin i = μ_{2} sin r$
$∴ i$ and r are small angles
so sin i=i and sin r=r
$\Rightarrow μ_{1} i = μ_{2} r$
Using eqns (x) and (xi)
$μ_{1} [\frac{A N}{P O} + \frac{A N}{P C}] = μ_{2} [\frac{A N}{P I} - \frac{A N}{P C}]$
$\Rightarrow \frac{μ_{1}}{P O} + \frac{μ_{1}}{P C} = \frac{μ_{2}}{P I} - \frac{μ_{2}}{P C}$ .....xii
Applying sign conventions
$P O = - u, P C = R, P I = - v$
From eqn xii
$\frac{μ_{1}}{- u} + \frac{μ_{1}}{R} = \frac{μ_{2}}{- v} + \frac{μ_{2}}{R}$
$\Rightarrow - \frac{μ_{1}}{u} + \frac{μ_{2}}{v} = \frac{μ_{2}}{R} - \frac{μ_{1}}{R}$
$- \frac{μ_{1}}{u} + \frac{μ_{2}}{v} = \frac{μ_{2} - μ_{1}}{R}$
Divide all the terms on both sides by $μ_{1}$ we have $- \frac{1}{u} + \frac{μ_{2} / μ_{1}}{v} = \frac{μ_{2} / μ_{1} - 1}{R}$
But $\frac{μ_{2}}{μ_{1}} =^{1} μ_{2} = μ =$ Refractive index of medium 2 w.r.t medium 1.
$\Rightarrow - \frac{1}{u} - \frac{μ}{v} = \frac{μ - 1}{R}$

|

Updated on:21/07/2023

Class 12 PHYSICS GEOMETRICAL OPTICS

Topper's Solved these Questions

Similar Questions

BETTER CHOICE PUBLICATION-REFRACTION OF LIGHT-LONG ANSWER TYPE QUESTIONS

Home
Ask Doubt
Profile