Prove that −μ1u+μ2v=μ2−μ1R when refraction occurs from rarer to denser medium at a concave spherical refracting surface.
To prove the equation −μ1u+μ2v=μ2−μ1R when refraction occurs from a rarer to a denser medium at a concave spherical refracting surface, we can follow these steps:
Step 1: Understand the Setup
We have a concave spherical surface separating two media: the rarer medium (with refractive index μ1) on one side and the denser medium (with refractive index μ2) on the other side. Let the radius of curvature of the spherical surface be R.
Step 2: Draw the Diagram
Draw a diagram representing the concave spherical surface. Mark the object position O at a distance u from the surface, the image position I at a distance v from the surface, and the center of curvature C. The normal at the point of incidence P should be drawn, which is perpendicular to the surface.
Step 3: Apply Snell's Law
According to Snell's Law, we have:
μ1sini=μ2sinr
For small angles, we can approximate sini≈i and sinr≈r, leading to:
μ1i=μ2r
Step 4: Relate Angles to Distances
Using geometry, we can relate the angles to the distances:
- Let α be the angle of incidence, β be the angle of refraction, and γ be the angle at the center of curvature.
- From the geometry of the situation, we can write:
- i=α−γ
- r=β−γ
Step 5: Use the Small Angle Approximation
For small angles, we can replace the angles with their tangents:
tanα≈α,tanβ≈β,tanγ≈γ
Thus, we can express:
α=QMu,β=QMv,γ=QMR
where QM is the height of the triangle formed by the rays.
Step 6: Substitute into Snell's Law
Substituting these into Snell's Law gives:
μ1(QMu−QMR)=μ2(QMv−QMR)
Step 7: Simplify the Equation
Cancelling QM (assuming QM≠0) from both sides results in:
μ1(1u−1R)=μ2(1v−1R)
Step 8: Rearranging Terms
Rearranging the equation gives:
μ11u−μ21v=μ1−μ2R
Rearranging further leads to:
−μ11u+μ21v=μ2−μ1R
Conclusion
Thus, we have proved that:
−μ1u+μ2v=μ2−μ1R
We have a concave spherical surface separating two media: the rarer medium (with refractive index μ1) on one side and the denser medium (with refractive index μ2) on the other side. Let the radius of curvature of the spherical surface be R.
Draw a diagram representing the concave spherical surface. Mark the object position O at a distance u from the surface, the image position I at a distance v from the surface, and the center of curvature C. The normal at the point of incidence P should be drawn, which is perpendicular to the surface.
According to Snell's Law, we have:
μ1sini=μ2sinr
For small angles, we can approximate sini≈i and sinr≈r, leading to:
μ1i=μ2r
Using geometry, we can relate the angles to the distances:
- Let α be the angle of incidence, β be the angle of refraction, and γ be the angle at the center of curvature.
- From the geometry of the situation, we can write:
- i=α−γ
- r=β−γ
For small angles, we can replace the angles with their tangents:
tanα≈α,tanβ≈β,tanγ≈γ
Thus, we can express:
α=QMu,β=QMv,γ=QMR
where QM is the height of the triangle formed by the rays.
Substituting these into Snell's Law gives:
μ1(QMu−QMR)=μ2(QMv−QMR)
Cancelling QM (assuming QM≠0) from both sides results in:
μ1(1u−1R)=μ2(1v−1R)
Rearranging the equation gives:
μ11u−μ21v=μ1−μ2R
Rearranging further leads to:
−μ11u+μ21v=μ2−μ1R
Thus, we have proved that:
−μ1u+μ2v=μ2−μ1R
Topper's Solved these Questions
- RAY OPTICSBook:PRADEEPChapter:RAY OPTICSExercise:Long Answer (c)
Explore 8 Videos
- RAY OPTICSBook:PRADEEPChapter:RAY OPTICSExercise:Long Answer (d)
Explore 6 Videos
- RAY OPTICSBook:PRADEEPChapter:RAY OPTICSExercise:(NCERT)Short Answer
Explore 6 Videos
- PROPERTIES OF BULK MATTERBook:PRADEEPChapter:PROPERTIES OF BULK MATTERExercise:Multiple choice questions
Explore 7 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBook:PRADEEPChapter:SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONExercise:Assertion- Reason Type questions
Explore 20 Videos
Similar Questions
Knowledge Check
The relation governing refraction of light from rarer to denser medium at a spherical refracting surface is
A−μ1u+μ2v=μ2−μ1RBμ1u+μ2v=μ2−μ1RCμ1u−μ2v=μ2−μ1RDnone of theseWhen a light ray from a rarer medium is refracted into a denser medium, its
ASpeed increases, wavelength increasesBSpeed decreases, wavelength increasesCSpeed increases, wavelength decreasesDSpeed decreases, wavelength decreasesIf μv,μr,μb are refractive indices of violet, red and blue, respectively, in a given medium, then
Aμv=μb=μrBμv>μb<μrCμv>μb>μrDμv>μb<μr
When refraction occurs from denser to……….., then μ2μ1 = …………. .
View SolutionWhen reflection occurs from denser to………….., then μ2μ1=……… .
View SolutionObtain lens maker's formula using the expression n2v−n1u=n2−n1R, propagating from a rarer medium of refractive index (n1) to a denser medium of refractive index (n2) is incident on the convex side of spherical refracting surface of radius of curvature R.
View SolutionFigure shows figure three transparent medi of refractive indices μ1,μ2andμ3. A point object O is placed in the medium μ2. If the entire medium on the right of the spherical surface has refractive index μ1, the image forms at O. If this entire medium has refractive index μ2 the image form at O''. In the situation shown

View SolutionIn the figure shown μ1,μ2,μ3,μ4andμ5 are the refractive indices
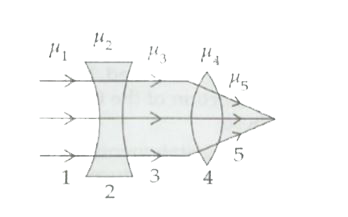
of the mediums 1,2,3,4 and 5 respectively . Consider the following
(1) μ1=μ2 (2) μ3=μ4=μ5
μ2<μ3 (4) μ4>μ5
View Solution
