Repeat the above problem, but with the current in both wires shown in fig. directed into the plane of the figure.
Derive the expression for the magnitude of $\to B$ at any point on the x-axis in terms of the x-coordinate of the point. What is the direciton of $\to B$ ?

A

$\frac{\sqrt{2} μ_{0} I a}{π (x^{2} + a^{2})}$

B

$\frac{3 μ_{0} I a}{π (x^{2} + a^{2})}$

C

$\frac{μ_{0} I a}{2 π (x^{2} + a^{2})}$

D

$\frac{μ_{0} I x}{π (x^{2} + a^{2})}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

(d)
At a position on the x-axis:
$B_{n e t} = 2 \frac{μ_{0} I}{2 π r} cos θ = \frac{μ_{0} I}{π (\sqrt{x^{2} + a^{2}})} \frac{a}{\sqrt{x^{2} + a^{2}}}$
$B_{n e t} = \frac{μ_{0} I x}{π (x^{2} + a^{2})}$
It's graphs is shown below.

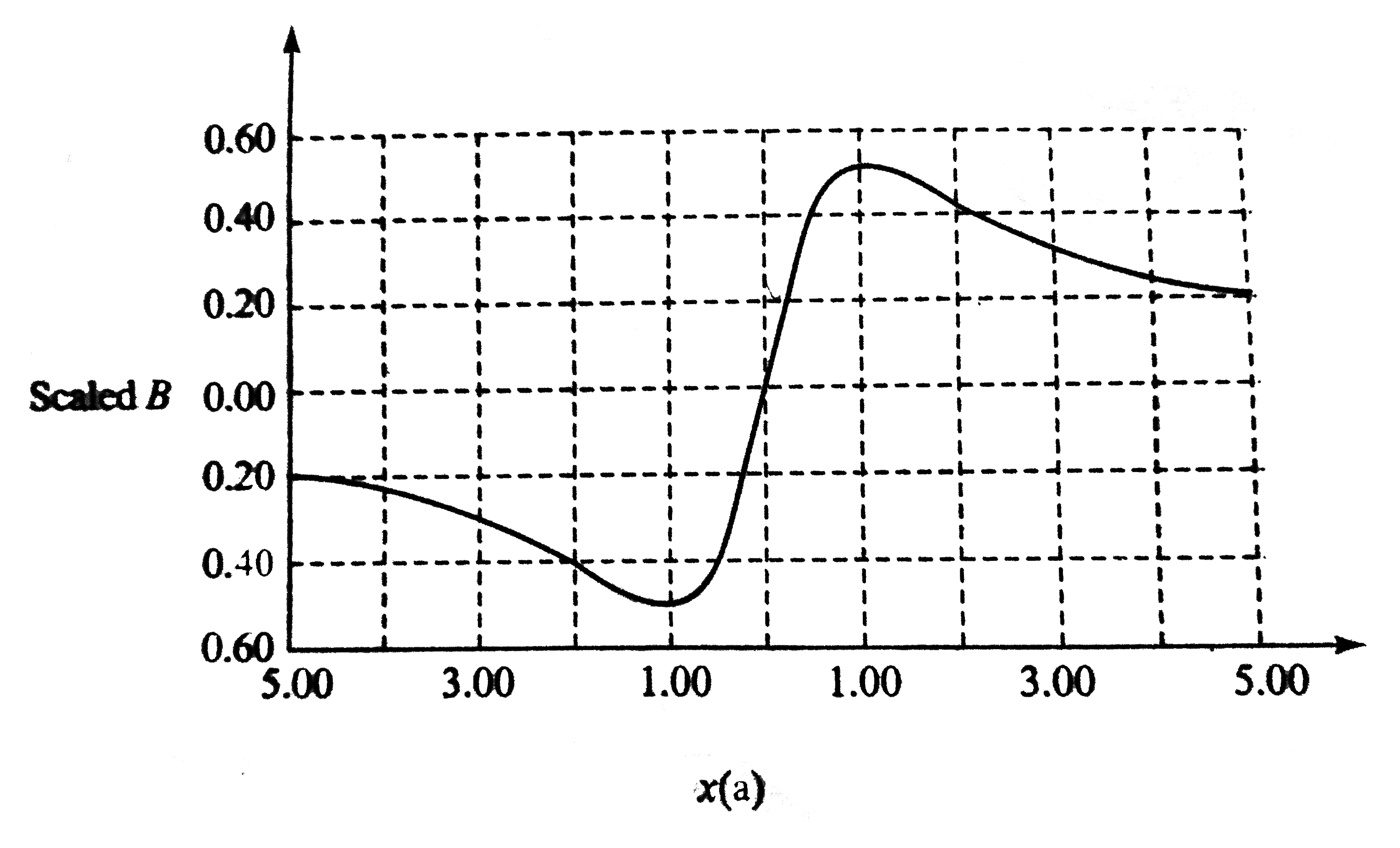
The magnetic field is a maximum when :
$\frac{d B}{d x} = 0 = \frac{C}{x^{2} + a^{2}} - \frac{2 C x^{2}}{{(x^{2} + a^{2})}^{2}}$
$(x^{2} + a^{2}) = 2 x^{2} \Rightarrow x = \pm a$
When $x > > a, B \approx \frac{μ_{0} I}{π x},$
which is just like a wire carrying current 2I.

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Repeat the above problem, but with the current in both wires shown in fig. directed into the plane of the figure.
At what value of x is the magnitude of $\to B$ is maximum?
A $x = 0$
B $x = \sqrt{2} a$
C $x = \pm a / 2$
D $x = \pm a$
Question 2 - Select One
Repeat the above problem, but with the current in both wires shown in fig. directed into the plane of the figure.
Graph the magnitude of $\to B$ at points on the x-axis.
A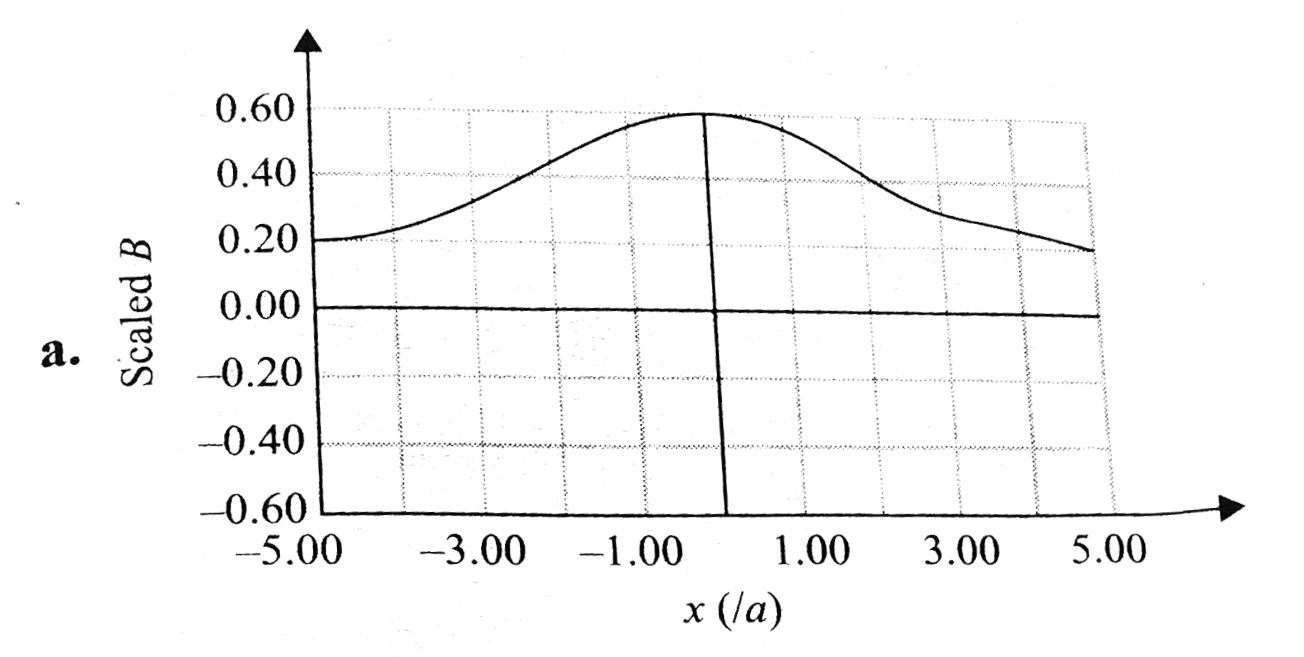
B
C
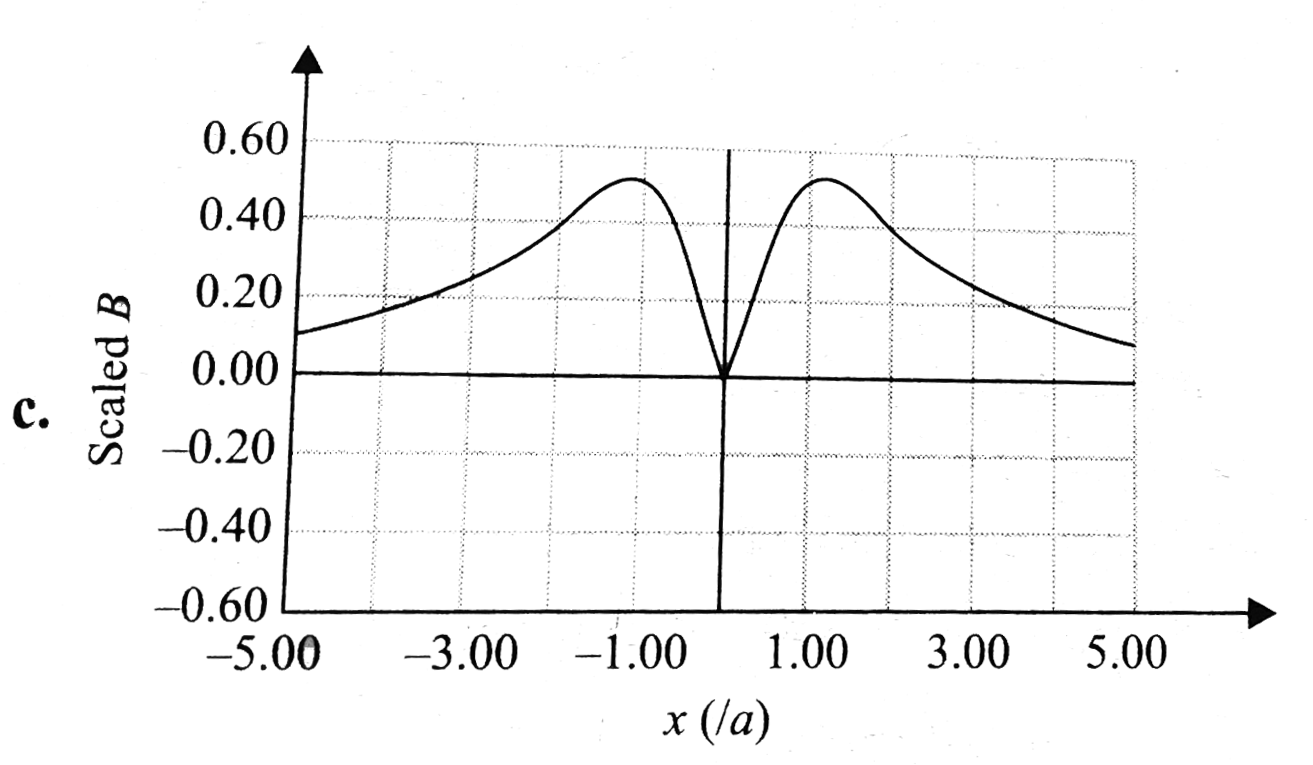D
Question 3 - Select One
Figure shows an end view of two long, parallel wires perpendicular to the xy plane, each carrying a current I, but in opposite directions.

Derive an expression for the magnitude of $\to B$ at any point on the x-axis in terms of the x-coordinate of the point. What is the direction of $\to B$ ?
A $\frac{\sqrt{2} μ_{0} I a}{π (x^{2} + a^{2})}$
B $\frac{3 μ_{0} I a}{π (x^{2} + a^{2})}$
C $\frac{μ_{0} I a}{2 π (x^{2} + a^{2})}$
D $\frac{μ_{0} I a}{π (x^{2} + a^{2})}$

CENGAGE PHYSICS-SOURCES OF MAGNETIC FIELD-Exercise (linked Comprehension)

Home
Ask Doubt
Profile