Sand is being dropped on a conveyor belt at the rate of Mkg/s . The force necessary to kept the belt moving with a constant with a constant velocity of vm/s will be.
Mv newton
2 Mv newton
Mv2 newton
zero
The correct Answer is:A
To solve the problem, we need to determine the force necessary to keep a conveyor belt moving at a constant velocity when sand is being dropped on it at a rate of M kg/s.
1. Understand the Problem:
- We have a conveyor belt moving with a constant velocity v m/s.
- Sand is being dropped onto the belt at a rate of M kg/s.
2. Apply Newton's Second Law:
- According to Newton's second law of motion, the force F is equal to the rate of change of momentum dpdt.
- The momentum p of an object is given by p=mv, where m is the mass and v is the velocity.
3. Differentiate Momentum:
- The change in momentum with respect to time can be expressed as:
F=dpdt=d(mv)dt
- Using the product rule of differentiation, we have:
F=vdmdt+mdvdt
4. Identify Constants:
- Since the conveyor belt moves with a constant velocity, dvdt=0.
- Therefore, the second term mdvdt becomes zero.
5. Substituting Values:
- We know that dmdt=M (the rate at which sand is dropped).
- Substituting these values into the equation gives:
F=v⋅M+0
- Thus, the force required to keep the conveyor belt moving at a constant velocity is:
F=Mv
Final Answer:
The force necessary to keep the belt moving with a constant velocity v m/s is F=Mv.
---
- We have a conveyor belt moving with a constant velocity v m/s.
- Sand is being dropped onto the belt at a rate of M kg/s.
- According to Newton's second law of motion, the force F is equal to the rate of change of momentum dpdt.
- The momentum p of an object is given by p=mv, where m is the mass and v is the velocity.
- The change in momentum with respect to time can be expressed as:
F=dpdt=d(mv)dt
- Using the product rule of differentiation, we have:
F=vdmdt+mdvdt
- Since the conveyor belt moves with a constant velocity, dvdt=0.
- Therefore, the second term mdvdt becomes zero.
- We know that dmdt=M (the rate at which sand is dropped).
- Substituting these values into the equation gives:
F=v⋅M+0
- Thus, the force required to keep the conveyor belt moving at a constant velocity is:
F=Mv
The force necessary to keep the belt moving with a constant velocity v m/s is F=Mv.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - AIIMS Questions
26 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test
30 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Assertion Reasoning
14 Videos
- MOTION IN TWO DIMENSIONBOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test
29 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test
29 Videos
Similar Questions
Sand drops from a stationary hopper at the rate of 5kg/s on to a conveyor belt moving with a constant speed of 2m/s. What is the force required to keep the belt moving and what is the power delivered by the motor, moving the belt?
View SolutionSand drops from a stationary hopper at the rate of 5 kg s−1 on to a conveyor belt moving with a constant speed of 2ms−1 . What is the force required to keep the belt moving and what is the power delivered by the motor moving the belt ?
[ Hint: Tangetial force exerted on the belt = ureldMdt where urel is the tangential relative velocity of the sand .]
View Solution
Knowledge Check
A cart is moving with a velocity 20m/s. Sand is being dropped into the cart at the rate of 50 kg/min. The force required to move the cart with constant velocity will be :-
A50 NB30.33NC26.45ND16.66NGravels are dropped on a conveyor belt at the rate of 0.5 kg / sec . The extra force required in newtons to keep the belt moving at 2 m/sec is
A1B2C4D0.5In the block moves up with constant velocity v m//s. Find F
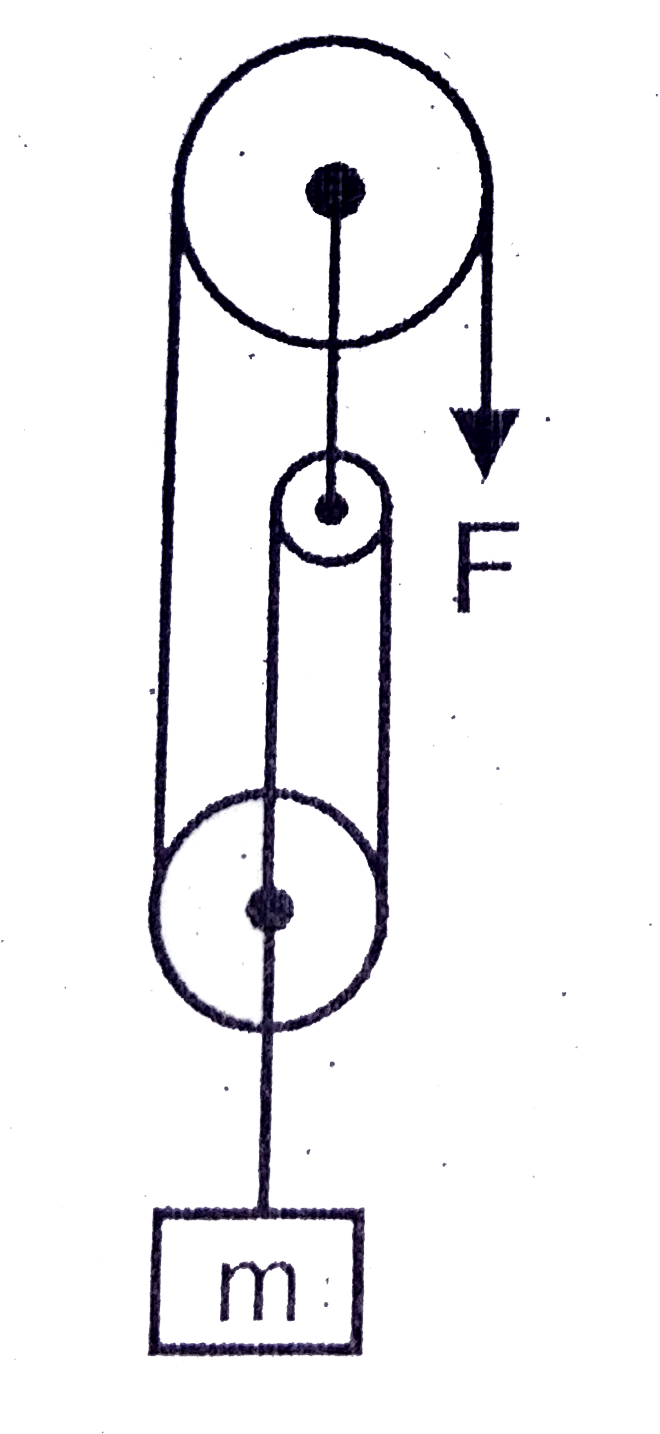 AF=mg2BF=2mg3CF=mg3DF=m3
AF=mg2BF=2mg3CF=mg3DF=m3
A package rest on a conveyor belt which is initially at rest . The belt is started and moves to the right for 1.3s with a constant acceleration of 2m/s2. The belt then moves with a constant deceleration a2 and comes to a stop after a total displacement of 2.2m . Knowing that the coefficient of friction between the package and the belt are μs=0.35 and μk=0.25 , determine (a) the deceleration a2 of the belt , (b) the displacement of the package relative to the belt as the belt comes to a stop. (g=9.8m/s2)

View SolutionA train is moving with velocity 20m/sec . on this dust is falling at the rate of 50 kg / minute . The extra force required to move this train with constant velocity will be
View SolutionA suitcase is gently dropped on a conveyor belt moving at 3m/s. If the coefficient of friction between the belt and the suitcase is 0.5. Find the displacement of suitcase relative to conveyor belt before the slipping between the two is stopped (g=10m/s2)
View SolutionA truck of mass 500kg is moving with constant speed 10ms−1. If sand is dropped into the truck at the constant rate 10kgmin,the force required to mainatain the motion with constant velocity is .
View SolutionAn observer A is at rest in gournd frame, observer B is moving with constant another observer C is moving with constant velocity of 1m/s.
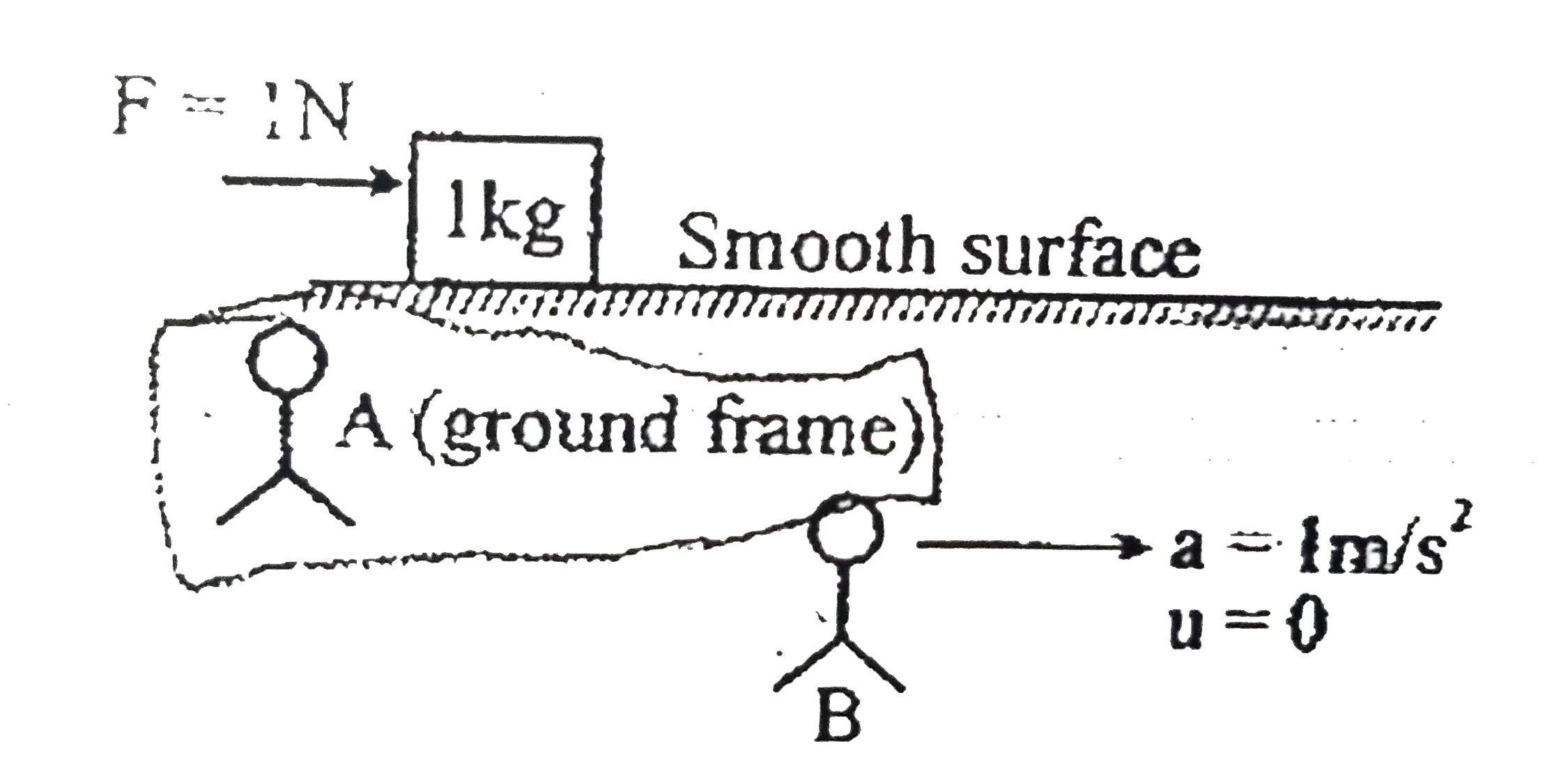
View Solution
