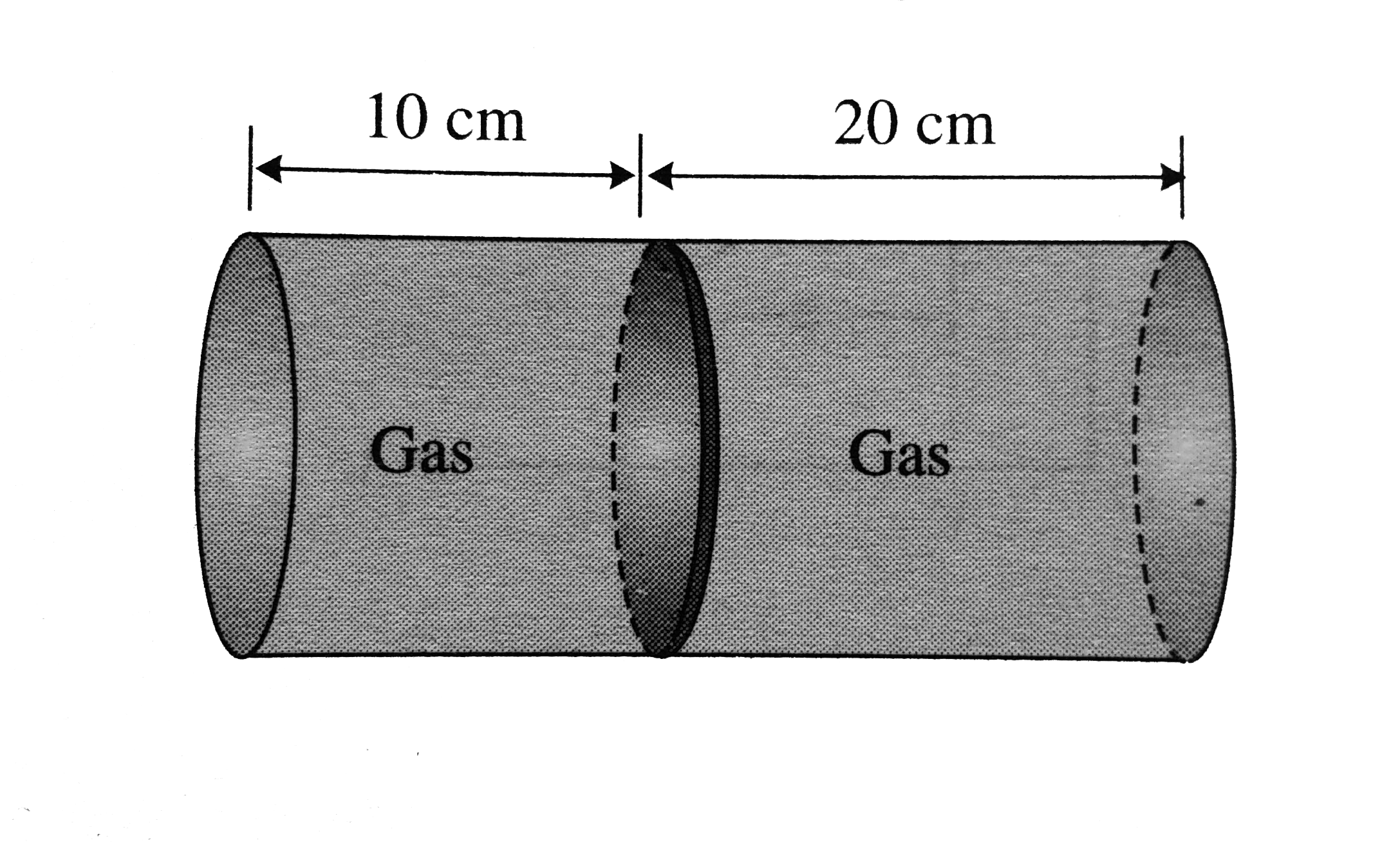
Show a cylindrical tube of volume V0 divided in two parts by a frictionless separator. The walls of the tube are adiabatic but the separator is conducting, Ideal gases are filled in the two parts. When the separator is kept in the middle, the pressure are p1 and p2 in the left part and the right part respectively. The separator is slowly slid and is released at a position where it can stay in equilibrium. Find the volumes if the two parts. Solution in Tamil
As the separator is conducting, the tenperature is T when the separator is in the middle. Let n_(1) and n_(2) be the number of moles of the gas in the left part and the right part respectively. Using ideal gas equation,
p_(1)(v_(0))/(2)=n_(1)RT
and p_(2)(v_(0)=n_(2)RT.
Thus, (n_(1))/(n_(2))=p_(1)/p_(2) ...(i)
The separator will stay in equilibrium at a position wher the pressures on the two sides are equal. Suppose the volume of the left part is V_(1) and of the right part is V_(2) in this situation. Let the common pressure be p'. Also, let the common temperature in this situation be T'. Using ideal gas equation,
p'V1=n1RT'
and p'V2=n2RT'
or, V1V2=n1n2=p1p2 [using(i)]
Also, V1+V2=V0
Thus, V1=p1V0p1+p2 and V2=p2V0p1+p2.
Topper's Solved these Questions
Similar Questions
Find the dimensions of
a. linear momentum
b. frequency and
c. pressure
View SolutionShould the internal energy of a system necessarily increase if heat is added to it?
View SolutionFigure shows a cylindridcal tube with a adibatic walls and fitted with an adiabatic separtor. The separator can be slid into the tube by an external mechanism. An ideal gas(γ=1.5) is injected in the two sides at equal pressures and temperatures . The separator remains in equilibrium at the middel. It is now slid to a position where it divides the tube in the ratio 1:3 Find the ratio of the tempertures in the two parts of the vessel.
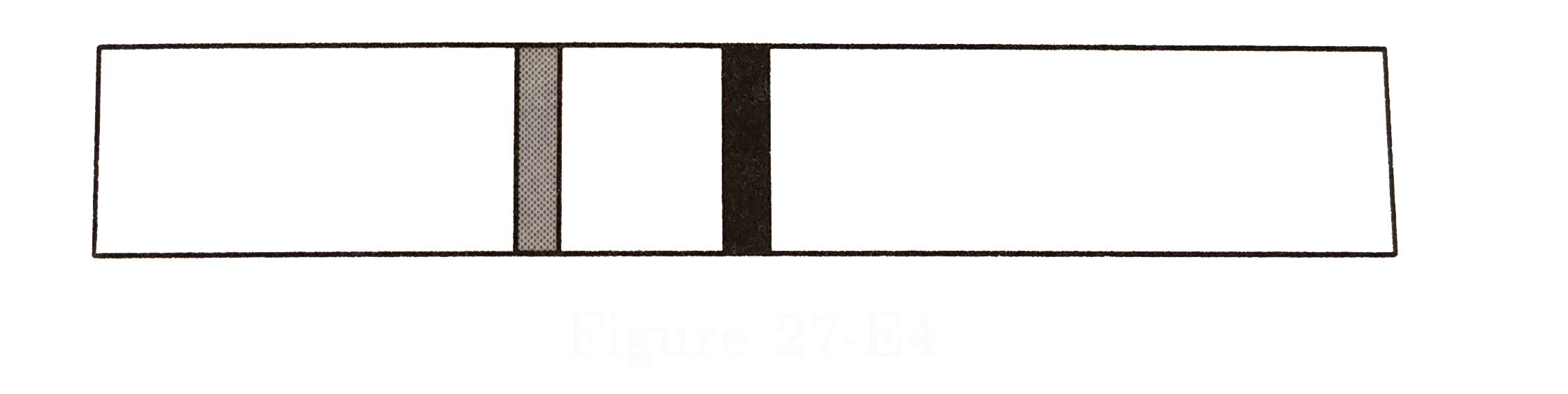
View SolutionFigure shows an adiabatic cylindrical tube of volume (V_0) divided in two parts by a frictionless adibatic separator . Initially, the separator is kept in the middle, an ideal gas at pressure (p_1) and the temperatures (T_1) is injected into the left part and the another ideal gas at pressures (P_2) and temperature (T_2) is injected into the right part. (C_p / C_v = gamma) is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the volumes of the parts, (b) the heat given to the gas in the left part and (c) the final common pressure of the gases.
View SolutionFig. shows a horzontal cylindrical container of length 30 cm , which is partitioned by a tight-fiting separator. The separator is diathermic but conductws heat very slowly. Initially the separator is the state shown in the figure. The temperature of left part of cylinder is 100 K and that on right part is 400 K . Initially the separator is in equilibrium. As heat is conducted from right to left part, of separator after a long when gases on the two displacement of separator after a long when gases on the two parts of cylinder are in thermal equilibrium.
View SolutionAn adiabatic cylindrical tube is fitted with an adiabatic separator as shown in figure. Initial separator is in equilibrium and divides a tube in two equal parts. The seperator can be slide into the tube by an extermal mechanism. An ideal gas (gamma = 1.5) is injected in the two slides at equal pressure and temperature. Now separator is slid to a piston where it divides the tube in the ratio 7 :3 The ratio of the temperature in the two parts of the vessel is sqrt(n) : sqrt(7) find n .
View SolutionIn given figure, an adiabatic cylindrical tube of volume 2V0 is divided in two equal parts by a frictionless adiabatic separator. An ideal gas in left side of a tube having pressure P1 and temperature T1 where as in the right side having pressure P2 and temperature T2.Cp/Cv=γ is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the final volumes of the two parts (b) the heat given to the gas in the left part and (c) the final common pressure of the gases,
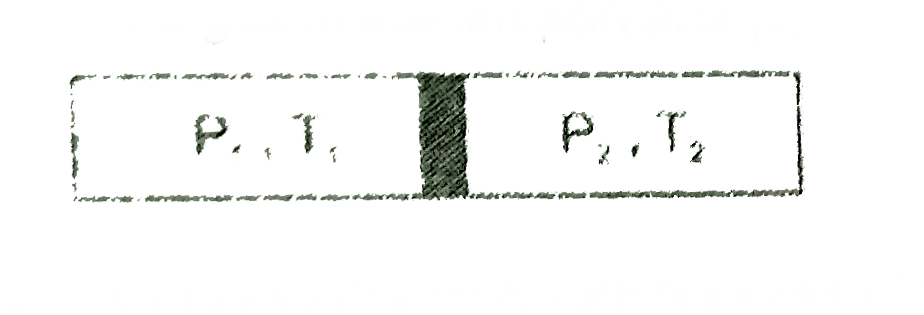
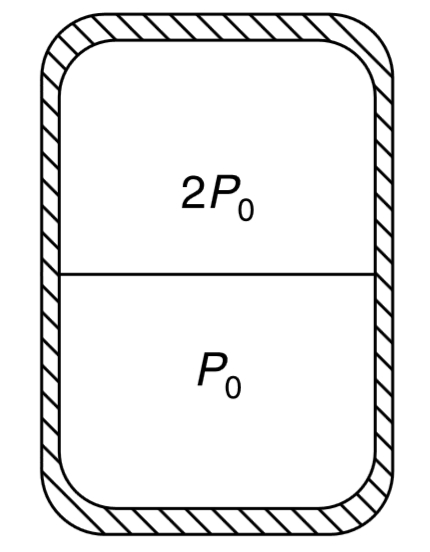
View SolutionA cylindrical container of volume V0 is divided into two parts by a thin conducting separator of negligible mass. The walls of the container are adiabatic. Ideal gases are filled in the two parts such that the pressures are P0 and 2P0 when the separator is held in the middle of the container (see figure). Now the separator is slowly slid and released in a position where it stays in equilibrium. Find the volume of the two parts.
View SolutionFunctional part of pollen tube is separated from the rest by
View SolutionFigure shows a cylindridcal tube with a adibatic walls and fitted with an adiabatic separtor. The separator can be slid into the tube by an external mechanism. An ideal gas(γ=1.5) is injected in the two sides at equal pressures and temperatures . The separator remains in equilibrium at the middel. It is now slid to a position where it divides the tube in the ratio 1:3 Find the ratio of the tempertures in the two parts of the vessel.

View SolutionFigure shows a horizontal cylindrical container of length 30 cm, which is partitioned by a tight fitting separator. The separator is diathermic but conducts heat very slowly. Initially the separator is in the state shown in figure. The temperature of left part of cylinder is 100K and that on right part is 400K. Initially the separator is in equilibrium. As heat is conducted from right to left part, separator displaces to the right. Find the displacement of separator after a long time when gases on the two parts of cylinder are in thermal equilibrium.
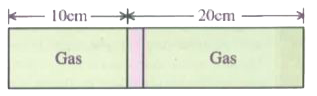
View SolutionA cylindrical tube of length 30 cm is partitioned by a tight-fitting separator. The separator is very thin, very weakly conducting and can freely slide along the tube. Ideal gases are filled in the two parts of the vessel. In the beginning, the temperature in the parts A and B are 400 K and 100 K respectively. The separator slides to a momentary equilibrium position at which the length of the tube at part A is 20 cm. Find the final equilibrium position of the separator, reached after a long time.
View SolutionA cylindrical container having non-conducting walls is partitioned in two equal parts such that the volume of the each parts is eqaul to V0 A movable non-conducting piston is kept between the two parts. Gas on left is slowly heated so that the gas on right is compressed upto volume V0/8. Find pressure and temperature on both sides if initial pressure and temperature, were P0 and T0 respectively. Also find heat given by the heater to the gas (number of moles in each parts is n)

View SolutionFigure shows an adiabatic cylindrical tube of volume (V_0) divided in two parts by a frictionless adiabatic separator . Initially, the separator is kept in the middle, an ideal gas at pressure (P_1) and the temperatures (T_1) is injected into the left part and the another ideal gas at pressures (P_2) and temperature (T_2) is injected into the right part. (C_p / C_v = gamma) is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the volumes of the parts, (b) the heat given to the gas in the left part and (c) the final common pressure of the gases.
View SolutionFig. shows a horzontal cylindrical container of length 30cm, which is partitioned by a tight-fiting separator. The separator is diathermic but conductws heat very slowly. Initially the separator is the state shown in the figure. The temperature of left part of cylinder is 100K and that on right part is 400K. Initially the separator is in equilibrium. As heat is conducted from right to left part, of separator after a long when gases on the two displacement of separator after a long when gases on the two parts of cylinder are in thermal equilibrium.
View SolutionA cylindrical container having non-conducting walls is partitioned in two equal parts such that the volume of the each parts is eqaul to V_(0) A movable non-conducting piston is kept between the two parts. Gas on left is slowly heated so that the gas on right is compressed upto volume V_(0)//8 . Find pressure and temperature on both sides if initial pressure and temperature, were P_(0) and T_(0) respectively. Also find heat given by the heater to the gas (number of moles in each parts is n)
View Solution
