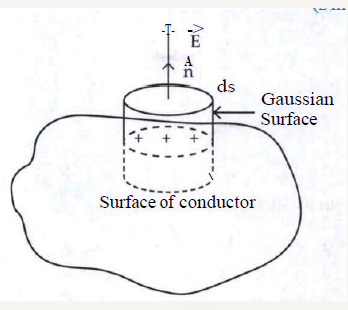
Show that electric field at the surface of a charges conductor is, →E=σE0ˆn where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction.
 Solution in Marathi
Solution in Marathi
Step by step video & image solution for Show that electric field at the surface of a charges conductor is, vecE=sigma/E_0 hatn where sigma is the surface charge density and hatn is a unit vector normal to the surface in the outward direction. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ElectrostaticsBOOK - CHETANA PUBLICATIONCHAPTER - ElectrostaticsEXERCISE - Exercise
73 Videos
- Electromagnetic InductionBOOK - CHETANA PUBLICATIONCHAPTER - Electromagnetic InductionEXERCISE - EXERCISE
109 Videos
- Kinetic Theory of Gases and RadiationBOOK - CHETANA PUBLICATIONCHAPTER - Kinetic Theory of Gases and RadiationEXERCISE - Exercise
110 Videos
Similar Questions
Calculate the total flux coming out from a closed surface enclosing a particle (42He). (Given : e=1.6×l0−19C)
View SolutionA magnetic flux associated with a coil changes by 5×10−2 Wb in 25 second. Find the emf induced in the coil.
View Solution16 gm of oxygen occupied 0.025m3 at 27∘C. It the universal gas constant is 8.311J/molK. Find the pressure exerted by it. (molecular weight of oxygen = 32)
View SolutionShow that the electric field at the surface of a charged conductor is given by vecE = (sigma)/(epsi_(0)) hatn , where sigma is the surface charge density and hatn is a unit vector normal to the surface in the outward direction .
View Solutionएक खोखले आवेशित गोले की सतह पर एक छोटा छिद्र काटा गया है। सिद्ध करो की छिद्र में वैधुत क्षेत्र (σ2ε0ˆn है, जहाँ ˆn अभिलम्बवत दिशा में बहिमुर्खी एकांक सदिश है तथा σ छिद्र के समीप आवेश पृष्ठ घनत्व है।
View Solutionदर्शाइए की आवेशित पृष्ठ के एक पाशर्व पर स्थिरवैद्युत क्षेत्र के अभिलंब घटक में असांतत्य होता है , जिस
(E2−E1).ˆn=σ∈0
द्वारा व्यक्त किया जाता है जहाँ ˆn एक बिन्दु पर पृष्ठ के अभिलंब एकांक सदिश है तथा σ उस बिंदु पर पृष्ठ आवेश धनत्व है (ˆn की दिशा पाशर्व1 से पाशर्व 2 की ओर है ) | अतः
दर्शाइए की चालक के ठीक बहार विद्युत क्षेत्र σˆn/∈0 है |
View Solutionसिद्ध कीजिए कि आवेशित चालक के पृष्ठ पर विद्युत क्षेत्र निम्न सूत्र द्वारा दिया जाता है- →E=σε0ˆn
View SolutionShow that electric field intensity on the surface of a charged conductor is vecE=(sigma)/(epsi_(0))n , where sigma is the surface density of charge and vecn is the outward pointing unit normal vector.
View SolutionWhat is the electric field in between the plates of the capacitor, if σ is the surface charge density?
View Solution(a) Show that the normal component of electrostatic field has a discontinuity from one side of a charged surface to another given by
(E2−E1).n=σε0
where ˆn is a unit vector normal to the surface at a point and σ is the surface charge density at that point. (The direction of ˆn is from side 1 to side 2.) Hence, show that just outside a conductor, the electric field is σˆn/ε0.
(b) Show that the tangential component of electrostatic field is continuous from one side of a charged surface to another. [Hint: For (a), use Gauss’s law. For, (b) use the fact that work done by electrostatic field on a closed loop is zero.]
View Solution(a) Show that the normal component of electrostatic field has a discontinuity from one side of a charged surface to another given by
(E2−E1).n=σε0
where ˆn is a unit vector normal to the surface at a point and σ is the surface charge density at that point. (The direction of ˆn is from side 1 to side 2.) Hence, show that just outside a conductor, the electric field is σˆn/ε0.
(b) Show that the tangential component of electrostatic field is continuous from one side of a charged surface to another. [Hint: For (a), use Gauss’s law. For, (b) use the fact that work done by electrostatic field on a closed loop is zero.]
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hole is (σ2∈0)ˆn , where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near the hole,
View SolutionShow that the electric field at the surface of a charged conductor is given by →E=σ60ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction.
View SolutionShow that the normal component of electrostatic field has a discontinuity from one side of a charged surface to another given by (vecE_2 - vecE_1). Htan = sigma/(epsi_0) where hatn is a unit vector normal to the surface at a point and o is the surface charge density at that point. (The direction of în is from side 1 to side 2). Hence, show that just outside a conductor, the electric field is sigma/(epsi_0) hatn .
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hold is (sigma)/(2pi epsilon_(0))hatn where hatn is the unit vector in the outward normal direction, and a is the surface charge density near the hole.
View Solution
