Show that the electric field at the surface of a charged conductor is given by →E=σ60ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction.
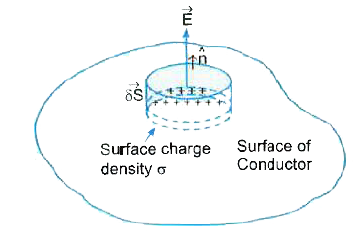
Consider a charged conductor whose surface charge density is σ. To derive electric field at its surface consider a short cylinder (pill box) as the Gaussian surface about a given point . The cylinder is partly inside and party outside the surface of the conductor. It has a small area of cross section δS and negligible height.
Just inside the surface , the electric field is zero but just outside the field has a magnitude E and is directed normal to the surface. Thus , the contribution to the electric flux comes only from the outside circular cross-section of the cylinder.
∴ϕ∈=→E.−→δS=E.δS
As per Gauss theorem.
ϕ∈=1∈0 (charged close) =1∈0.(δσS) ...(ii)
Comparing (i) and (ii) we get
E=σ∈0⇒ →E=σ∈0ˆn
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - U-LIKE SERIESCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - LONG ANSWER QUESTIONS -II
9 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - U-LIKE SERIESCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SELF ASSESSMENT TEST (SECTION-A)
5 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - U-LIKE SERIESCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SHORT ANSWER QUESTIONS
29 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - U-LIKE SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - SELF ASSESSMENT TEST SECTION-C (VERY SHORT ANSWER QUESTIONS)
3 Videos
- ELECTROMAGNETIC WAVESBOOK - U-LIKE SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - SELF ASSESSMENT TEST (SECTION C)
1 Videos
Similar Questions
Show that the electric field at the surface of a charged conductor is given by →E=σε0ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction .
View SolutionShow that the electric field at the surface of a charged conductor is given by →E=σε0ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction .
View Solution
Knowledge Check
The electric intensity at a point near a charged conductor of surface charge density σ is
AE=σ/∈0kBE=σ∈0kCE=σ/2∈0kDE=σ2
Show that the electric field at the surface of a charged conductor is proportional to the surface charge density.
View SolutionWhy the electric field at the outer surface of a hollow charged conductor is normal to the surface?
View SolutionWhy the electric field at the outer surface of a hollow charged conductor is normal to the surface?
View SolutionWhat is the direction of the electric field at the surface of a charged conductor having charge density σ <0?
View SolutionShow that the normal component of electrostatic field has a discontinuly form one side of a charged. Surface to another given by (−→E2−−→E1).ˆn=σ∈0
where ˆn is a unit vector normal to the surface at a point and σ at a point and σ is the surface charge density at that point. (The direction of ˆn is from side 1 to side 2). Hence show that justy outside a conductor, the electric field σˆn/∈0.
(b) Show that the tangential componet of electrostatic field is contionous from one side fo a charged surface to another.
View SolutionShow that the normal component of electrostatic field has a discontinuity from one side of a charged surface to another given by
(→E2−→E1).Htan=σε0
where ˆn is a unit vector normal to the surface at a point and o is the surface charge density at that point. (The direction of în is from side 1 to side 2). Hence, show that just outside a conductor, the electric field is σε0ˆn.
View SolutionThe magnitude of the electric field on the surface of a sphere of radius r having a uniform surface charge density σ is
View Solution
