Show that for a projectile the angle between the velocity and the x-axis as function of time is given
by
(b) Shows that the projection angle for a projectile launched from the origin is given by
where the symbols have their usual meanings.
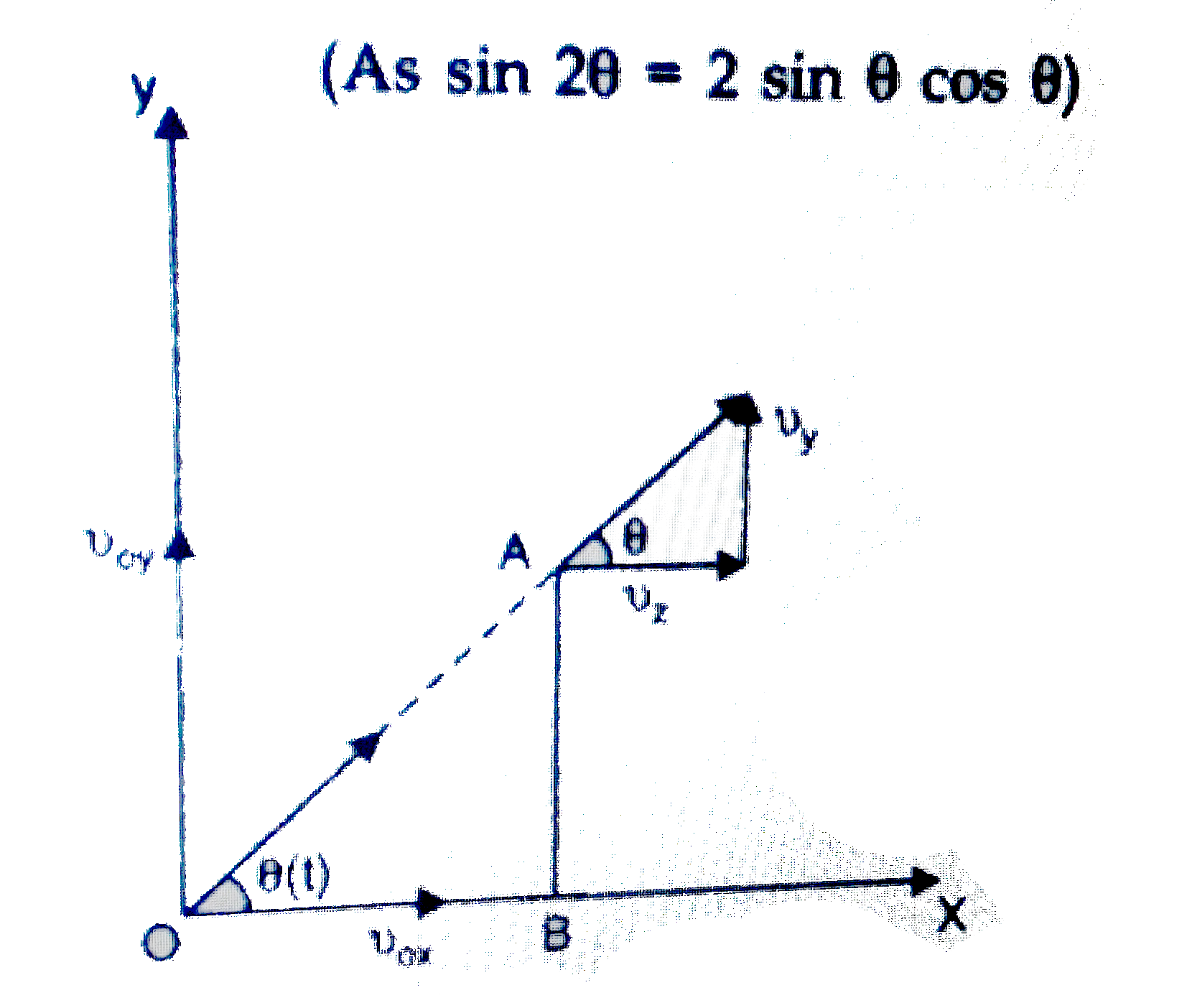
a) Let the principle be fired at an angle with x-axis. As depends on t, (t), at any instant
b) Since,
and
(As
or
or
Topper's Solved these Questions
Similar Questions
(a) Show that for a projectile the angle between the velocity and the X-axis as a function of time is given by
θ(t)=tan−1[voy−gtvox]
(b) Show that the projection angle θ0 for a projectile lunched from the origin is given by θ0=tan−1(4hmR),
where the symbols have their usual meanings .
View Solution(a) Show that for a projectile the angle between the velocity and the x-axis as function of time is given
by theta_(t) = tam ^(-1) ( 9 4 h_m)/R)
where the sybols have thir usual meanings.
View Solution
Knowledge Check
If the angle between the lines √3y−x+4=0 and x+y−6=0 is θ, then tan θ is equal to.
A√3+1B√3−1C2+√3D3+√2The angle between the two lines 1x+my+n=0 and 1'x+m'y+n'=0 is given by tan−1θ. What θ equal to ?
A∣∣∣lm'−l'mll'−mm'∣∣∣B∣∣∣lm'+l'mll'+mm'∣∣∣C∣∣∣lm'−l'mll'+mm'∣∣∣D∣∣∣lm'+l'mll'−mm'∣∣∣A projectile is fired from the origin with a velocity v0 at an angle θ with x-axis. The speed of the projectile at an altitude h is .
Av0cosθB√v20−2ghC√v20sin2θ−2ghDNone of these
Show that projection angle θ0 for a projectile launched from the origin is given by:
θ0=tan−1[4HmR] Where Hm= Maximum height, R=Range
View SolutionIf (θ) is an acute angle between the lines y=2x+3,y=x+1 then the value of tanθ=
View SolutionProve that for a projectile fired from level ground at an angle θ above the horizontal, the ratio of the maximum height H to the range R is given by
HR=14tanθ
View SolutionProve that the time of flight T and the horizontal range R of a projectile are connected by the equation: gT2=2Rtanθ, where θ is the angle of projection.
View SolutionShow that the equation of the passing through the origin and making an angle θwith the y=mx+cis yx=±m+tanθ1−mtanθ.
View Solution
