Show that the area of the triangle contained between the vectors J and $ is one haif0fthe magnitude of $\to a \times \to b$ .

Video Solution

|

Answer

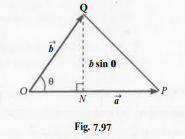
Step by step video & image solution for Show that the area of the triangle contained between the vectors J and $ is one haif0fthe magnitude of oversetrarraxxoversetrarrb. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS SYSTEM OF PARTICLES AND ROTATIONAL MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The angle between the vectors i-j+k and -i+j+2k is
A $45^{\circ}$
B $60^{\circ}$
C $90^{\circ}$
D $135^{\circ}$
Question 2 - Select One
The resultant of the vectors A and B is perpendicular to the vector A and its magnitude is equal to half the magnitude of vector B. The angle between $\to A and \to B$ is :-
A $120^{\circ}$
B $150^{\circ}$
C $135^{\circ}$
DNone of these
Question 3 - Select One
Two vectors of equal magnitude $P$ are inclined at some angle such that the difference in magitude of resultant and magnitude of either of the vectors is $0.732$ times either of the magnitude of vectors. If the angle between them is increased by half of its initial value then find the magnitude of difference of the vectors
A $2 P$
B $\sqrt{2} P$
C $3 P$
D $\sqrt{3} P$

SL ARORA-System of particles & rotational Motion-EXERCISE

Home
Ask Doubt
Profile