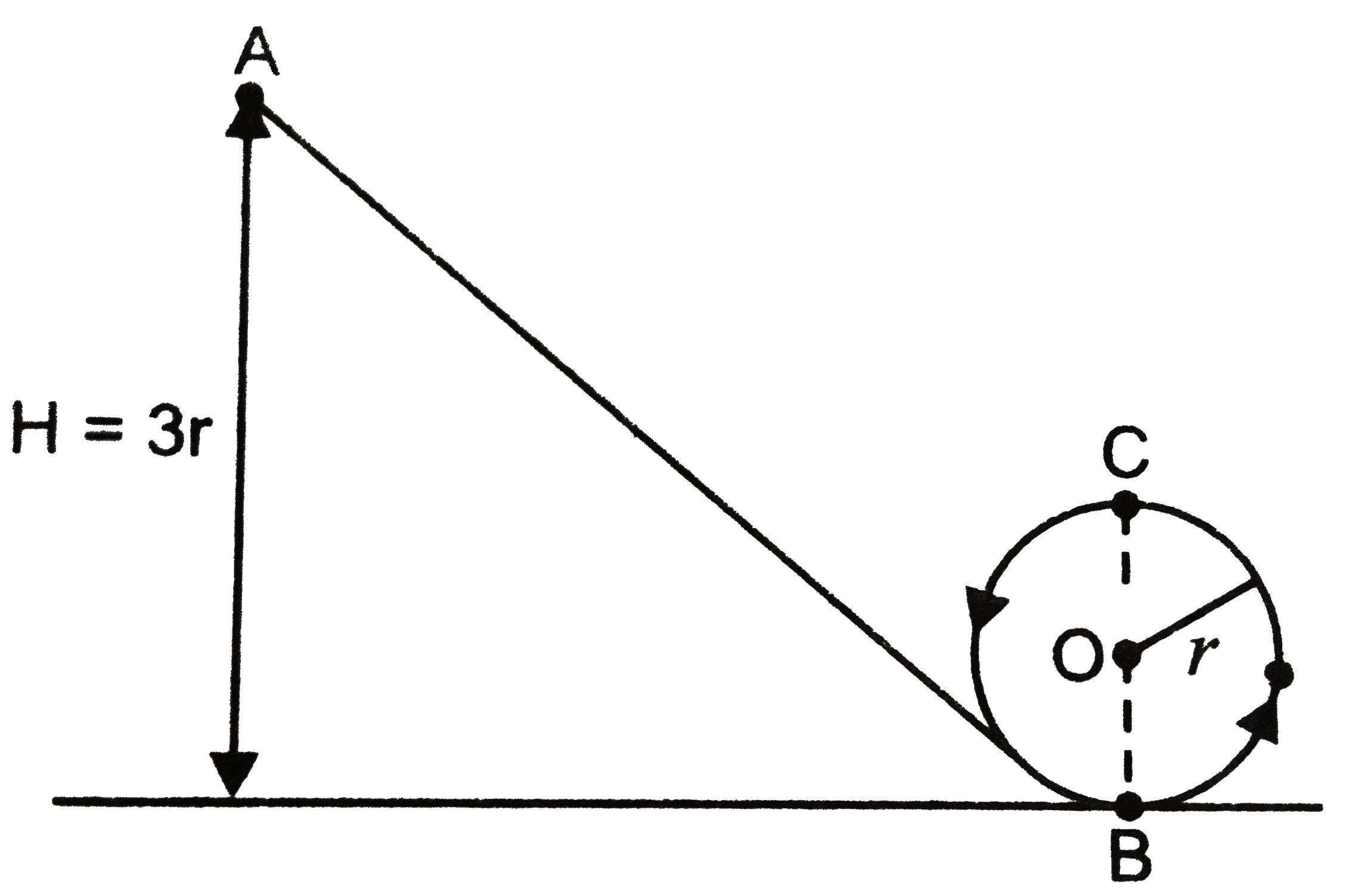
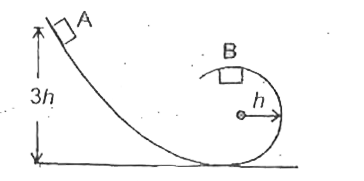
Shown a smooth looping the loop track A particle of mass m is released from oint A , as shown If H = 3 r , whould the particle loop the loop ? What is the force on the circular track when the particle is at point (i) B (ii) C ? .
Here , H=3r
When the particle of mass m is released from A , velocity acquired by it on reaching B is
υB=√2gH=√2g×3r=√6gr
which is greater than sqrt(5 g r ) , the minimum velocity required at B for looping the loop Hence the particle will loop the loop
Force exerted by the circular track on the particle at B
=mg+mv2Br
N1=mg+m×6grr=7mg
Force exerted by circular track on the particle at C
N2=mυ2cr−mg,
where υ2C=υ2B−4gr=6gr−4gr=2gr
∴N2=m×2grr−mg=mg
N1N2 are force exerted on the circular track by the particle at B , C respectively
 .
.
Similar Questions
A particle of mass m is released from point A as shown in the gigure. Would it loop the loop if H=4r? What is the force on the circular track when it is at point (a) B, (b) C?
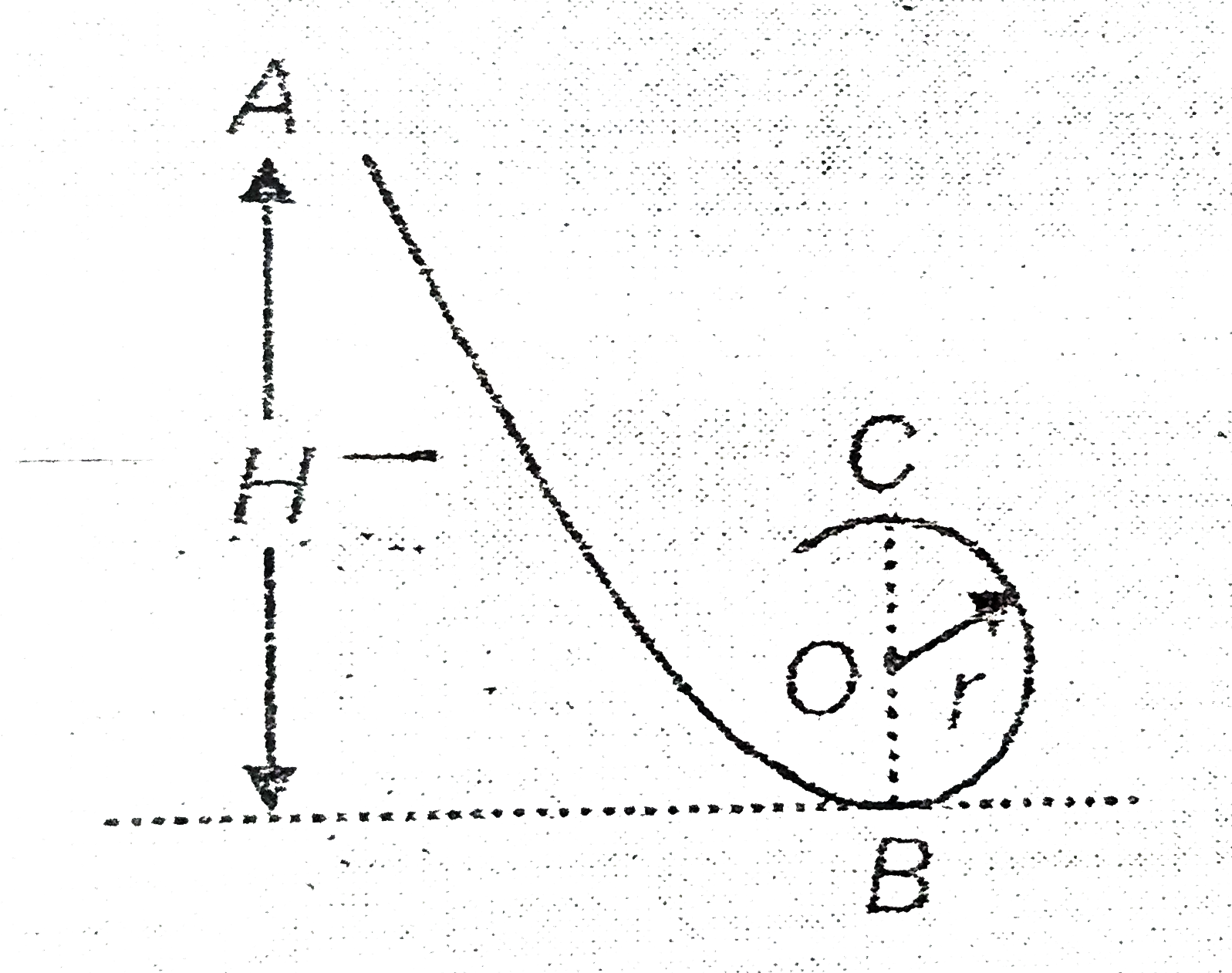
View SolutionIf in the loop-the-loop track of figure a small spherical ball of mass m is released and it rolls without slipping down the loop, calcualte the normal force exerted by the track on the ball when it is at the highest position. Take R: r=6:1
View Solution
Knowledge Check
A particle of mass m is released from a height H on a smooth curved surface which ends into a vertical loop of radius R, as shown. Choose the correct alernative(s) if H=2R.
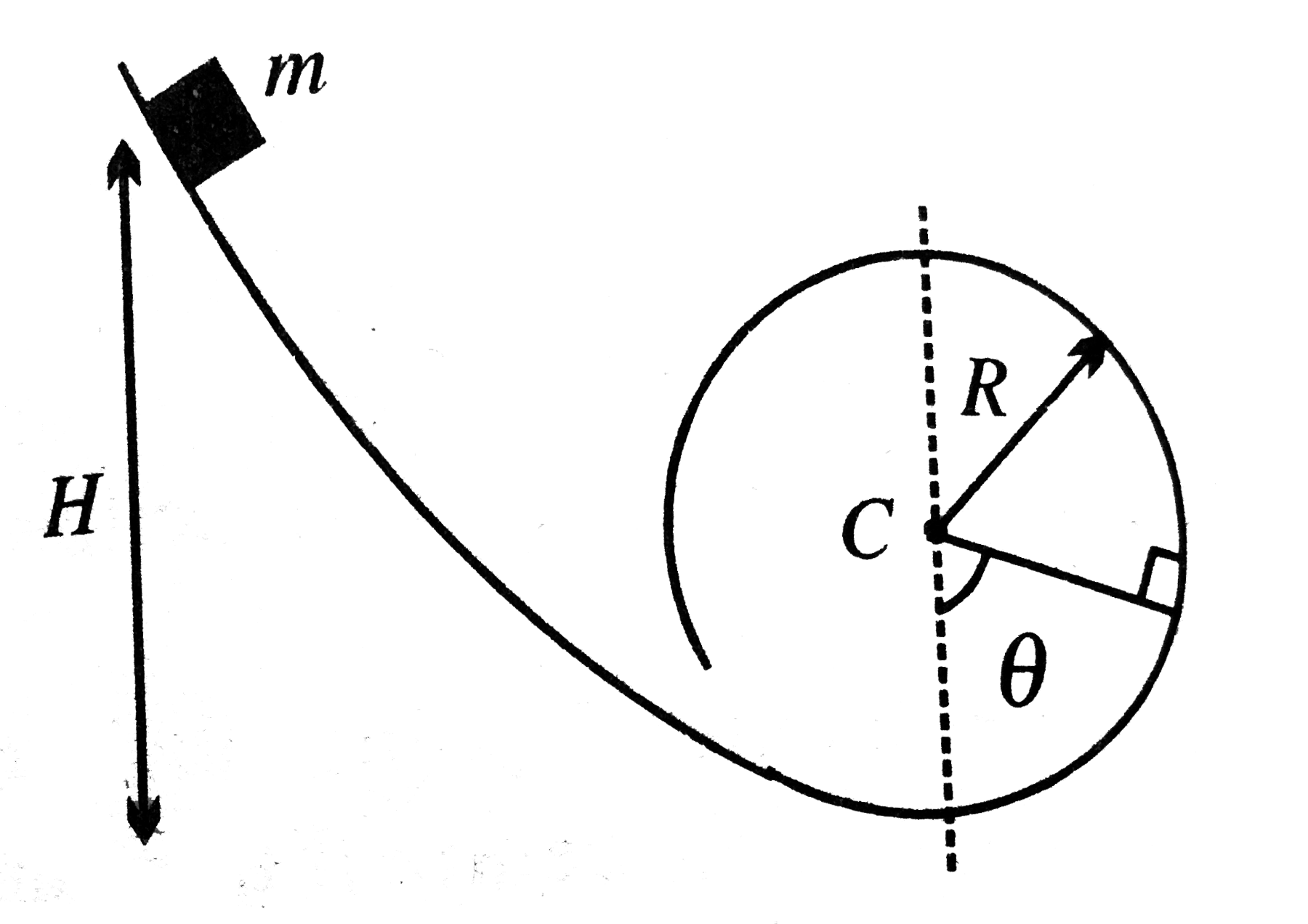 AThe particles reaches the top of the loop with zero velocityBThe particle cannot reach the top of the loop.CThe particle breaks off at a height h=R from the base of the loop.DThe particle breaks off at a height h from the base of the loop such that R<h<2R
AThe particles reaches the top of the loop with zero velocityBThe particle cannot reach the top of the loop.CThe particle breaks off at a height h=R from the base of the loop.DThe particle breaks off at a height h from the base of the loop such that R<h<2RIn the figure shown, a particle is released from the position A on a smooth track. When the particle reaches at B, then normal reaction on it by the track. When the particle reaches at B, then normal reaction on it by the track is
 AmgB2mgC23mgDm2gh
AmgB2mgC23mgDm2ghFigure shows a circular frications trck of radius R, centred at point O. A particle of mass M is released from point A(OA=R/2). After collision with the track, the particle moves along on the track. Then the coefficient of restitution e is.
 A0.2B0.5C1Dzero
A0.2B0.5C1Dzero
A particle of mass 100 g is moving in a vertical circle of radius 2 m The particle is just looping the loop . What is the speed of the particle and tension in the string at the highest point of the circular path ? . (g=10ms−2) .
View SolutionA small block of mass m slides along a frictionless loop-the-loop (loop inside loop) track. What should be the ratio of the radius of the outer loop to the radius of the inner loop so that the the block may not lose contact at the highest point of the inner loop ?
View SolutionAn object of mass m is released from rest at a height h above the surface of a table. The object slides along the inside of the loop. The loop track consisting of a ramp and a circular loop of radius R shown in the figure. Assume that the track is frictionless. When the object is at the top of the circular track it pushes against the track with a force equal to three times its weight. What height was the object dropped from?

View SolutionA small of mass m starts from rest at the position shown and slides along the frictionless loop the loop track of radius R. What is the smallest value of y such that the object will slide without losing contact with the track?

View SolutionA particle of mass 200 g is moving in a circle of radius 2 m. The particle is just looping the loop. The speed of the particle and the tension in the string at highest point of the circule path are (g=10ms−2)
View Solution