Sketch graph showing the variation of (i) the kinetic energy, (ii) the potential energy and (iii) the total energy of a simple harmonic oscillation with displacement.
To sketch the graphs showing the variation of kinetic energy, potential energy, and total energy of a simple harmonic oscillator with displacement, we can follow these steps:
Step 1: Understand the formulas for kinetic and potential energy
- The potential energy (PE) in simple harmonic motion (SHM) is given by:
PE=12kx2
where k is the spring constant and x is the displacement from the mean position.
- The kinetic energy (KE) is given by:
KE=12mv2
where v is the velocity. For SHM, the velocity can be expressed as:
v=ω√A2−x2
where A is the amplitude and ω is the angular frequency. Therefore, the kinetic energy can be expressed as:
KE=12mω2(A2−x2)
Step 2: Determine the maximum and minimum values
- At maximum displacement (x=A or x=−A):
- Potential Energy (PE) is maximum:
PEmax=12kA2
- Kinetic Energy (KE) is zero:
KE=0
- At the mean position (x=0):
- Kinetic Energy (KE) is maximum:
KEmax=12mω2A2
- Potential Energy (PE) is zero:
PE=0
Step 3: Sketch the graphs
1. Potential Energy (PE) Graph:
- The graph of potential energy versus displacement is a parabola opening upwards, starting from the origin (0,0) and reaching a maximum at x=A and x=−A.
2. Kinetic Energy (KE) Graph:
- The graph of kinetic energy versus displacement is also a parabola, but it opens downwards. It reaches its maximum value at x=0 and drops to zero at x=A and x=−A.
3. Total Energy (TE) Graph:
- The total energy remains constant throughout the motion. It can be represented as a horizontal line at the level equal to the maximum potential energy (or maximum kinetic energy) since:
TE=PE+KE=constant
Final Graphs
- On the same set of axes, plot:
- The potential energy curve (upward parabola).
- The kinetic energy curve (downward parabola).
- The total energy line (horizontal line).
- The potential energy (PE) in simple harmonic motion (SHM) is given by:
PE=12kx2
where k is the spring constant and x is the displacement from the mean position.
- The kinetic energy (KE) is given by:
KE=12mv2
where v is the velocity. For SHM, the velocity can be expressed as:
v=ω√A2−x2
where A is the amplitude and ω is the angular frequency. Therefore, the kinetic energy can be expressed as:
KE=12mω2(A2−x2)
- At maximum displacement (x=A or x=−A):
- Potential Energy (PE) is maximum:
PEmax=12kA2
- Kinetic Energy (KE) is zero:
KE=0
- Kinetic Energy (KE) is maximum:
KEmax=12mω2A2
- Potential Energy (PE) is zero:
PE=0
1. Potential Energy (PE) Graph:
- The graph of potential energy versus displacement is a parabola opening upwards, starting from the origin (0,0) and reaching a maximum at x=A and x=−A.
- The graph of kinetic energy versus displacement is also a parabola, but it opens downwards. It reaches its maximum value at x=0 and drops to zero at x=A and x=−A.
- The total energy remains constant throughout the motion. It can be represented as a horizontal line at the level equal to the maximum potential energy (or maximum kinetic energy) since:
TE=PE+KE=constant
- On the same set of axes, plot:
- The potential energy curve (upward parabola).
- The kinetic energy curve (downward parabola).
- The total energy line (horizontal line).
Topper's Solved these Questions
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - VERY SHORT ANSWER QUESTIONS13videos
OSCILLATIONS
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - SELECTED PROBLEMS (FROM THE CHARACTERISTIC OF SHM)14videos
OSCILLATIONS
- BOOK - ICSECHAPTER - OSCILLATIONS EXERCISE - LONG ANSWER QUESTIONS3videos
OSCILLATIONS
- BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)19videos
MOTION IN FLUIDS
- BOOK - ICSECHAPTER - PROPERTIES OF MATTEREXERCISE - MODULE 4 ( TEMPERATURE ) UNSOLVED PROBLEMS12videos
PROPERTIES OF MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
The total energy of a simple harmonic oscillation is proportional to
AamplitudeBsquare of amplitudeCfrequencyDvelocity - Question 2 - Select One
The kinetic energy and potential energy of a particle executing simple harmonic motion will be equal when displacement (amplitude = a) is
Aa/2Ba√2Ca/√2Da√2/3
In the conditions of the previous problem find the kinetic, the potential and the total energy of the oscillator.
View SolutionAt what displacement the kinetic is equal to the potential energy ?
View SolutionDifferentiate between Kinetic energy and potential energy.
View SolutionA linear harmonic oscillator has a total mechanical energy of 200J. Potential energy of it at mean position is 50J. Find
(i) the maximum kinetic energy,
(ii)the minimum potential energy,
(iii) the potential energy at extreme positions.View SolutionA linear harmonic oscillator has a total mechanical energy of 200J. Potential energy of it at mean position is 50J. Find
(i) the maximum kinetic energy,
(ii)the minimum potential energy,
(iii) the potential energy at extreme positions.View SolutionDraw the graph showing thervariation of binding energy per nucleon with mass number. Give therason for the decrease of binding energy per nucleon for nuclei with high mass numbers.
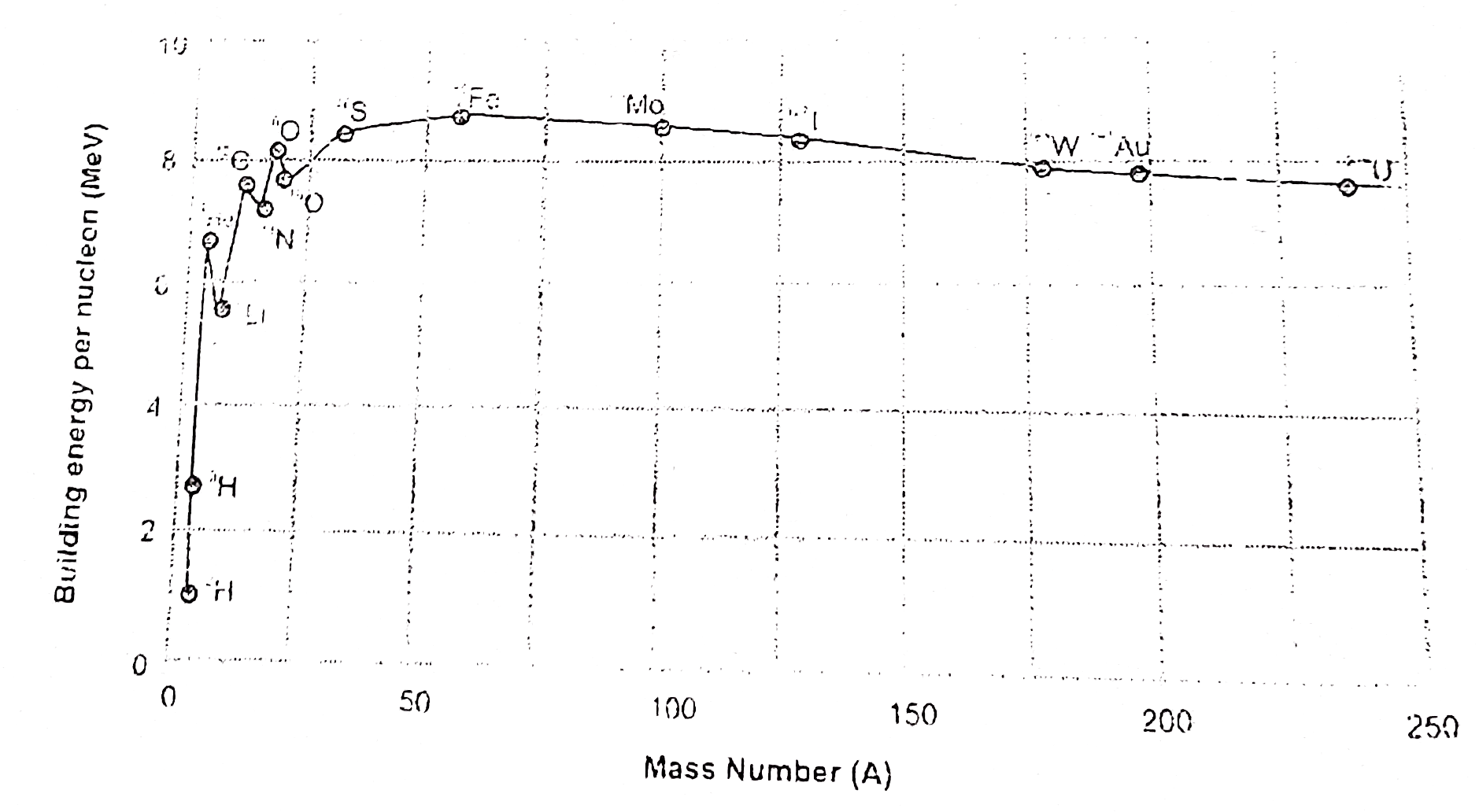
The binding energy per nucleon for nuclei with high mass number is low due to the large coulomb repulsion between the protons inside these nuclei.View Solution

