State Torricelli's theorem.
Step-by-Step Solution:
1. Definition of Torricelli's Theorem:
Torricelli's Theorem states that the speed (velocity) of a fluid flowing out of an orifice (a small hole) at the bottom of a container filled with liquid is equal to the speed that the liquid would attain if it were to fall freely from the height of the liquid surface above the orifice.
2. Understanding the Setup:
Consider a container filled with liquid to a height h. An orifice is created at the bottom of the container. The liquid will flow out of this orifice due to the gravitational force acting on it.
3. Application of Bernoulli's Equation:
To derive the velocity of the fluid exiting the orifice, we can apply Bernoulli's equation, which relates the pressure, velocity, and height of a fluid in motion. For the fluid at the surface of the liquid, the pressure is atmospheric, and the height is h.
4. Velocity Calculation:
According to Bernoulli's principle, the velocity v of the fluid exiting the orifice can be derived as:
v=√2gh
where g is the acceleration due to gravity, and h is the height of the liquid column above the orifice.
5. Interpretation:
This equation shows that the speed of the fluid exiting the orifice is equivalent to the speed a body would gain when falling freely from a height h under the influence of gravity.
Final Statement of Torricelli's Theorem:
Thus, Torricelli's Theorem can be summarized as: "The speed of efflux of a fluid through an orifice is equal to the speed that it would attain if falling freely from a height equal to the height of the liquid above the orifice."
---
Torricelli's Theorem states that the speed (velocity) of a fluid flowing out of an orifice (a small hole) at the bottom of a container filled with liquid is equal to the speed that the liquid would attain if it were to fall freely from the height of the liquid surface above the orifice.
Consider a container filled with liquid to a height h. An orifice is created at the bottom of the container. The liquid will flow out of this orifice due to the gravitational force acting on it.
To derive the velocity of the fluid exiting the orifice, we can apply Bernoulli's equation, which relates the pressure, velocity, and height of a fluid in motion. For the fluid at the surface of the liquid, the pressure is atmospheric, and the height is h.
According to Bernoulli's principle, the velocity v of the fluid exiting the orifice can be derived as:
v=√2gh
where g is the acceleration due to gravity, and h is the height of the liquid column above the orifice.
This equation shows that the speed of the fluid exiting the orifice is equivalent to the speed a body would gain when falling freely from a height h under the influence of gravity.
Thus, Torricelli's Theorem can be summarized as: "The speed of efflux of a fluid through an orifice is equal to the speed that it would attain if falling freely from a height equal to the height of the liquid above the orifice."
Topper's Solved these Questions
- Mechanical Properties of fluidsBOOK - SL ARORACHAPTER - Mechanical Properties of fluidsEXERCISE - Exercise
459 Videos
- Mathematical toolsBOOK - SL ARORACHAPTER - Mathematical toolsEXERCISE - Exercise
20 Videos
- Mechanical Properties of SolidsBOOK - SL ARORACHAPTER - Mechanical Properties of SolidsEXERCISE - Example
113 Videos
Similar Questions
Knowledge Check
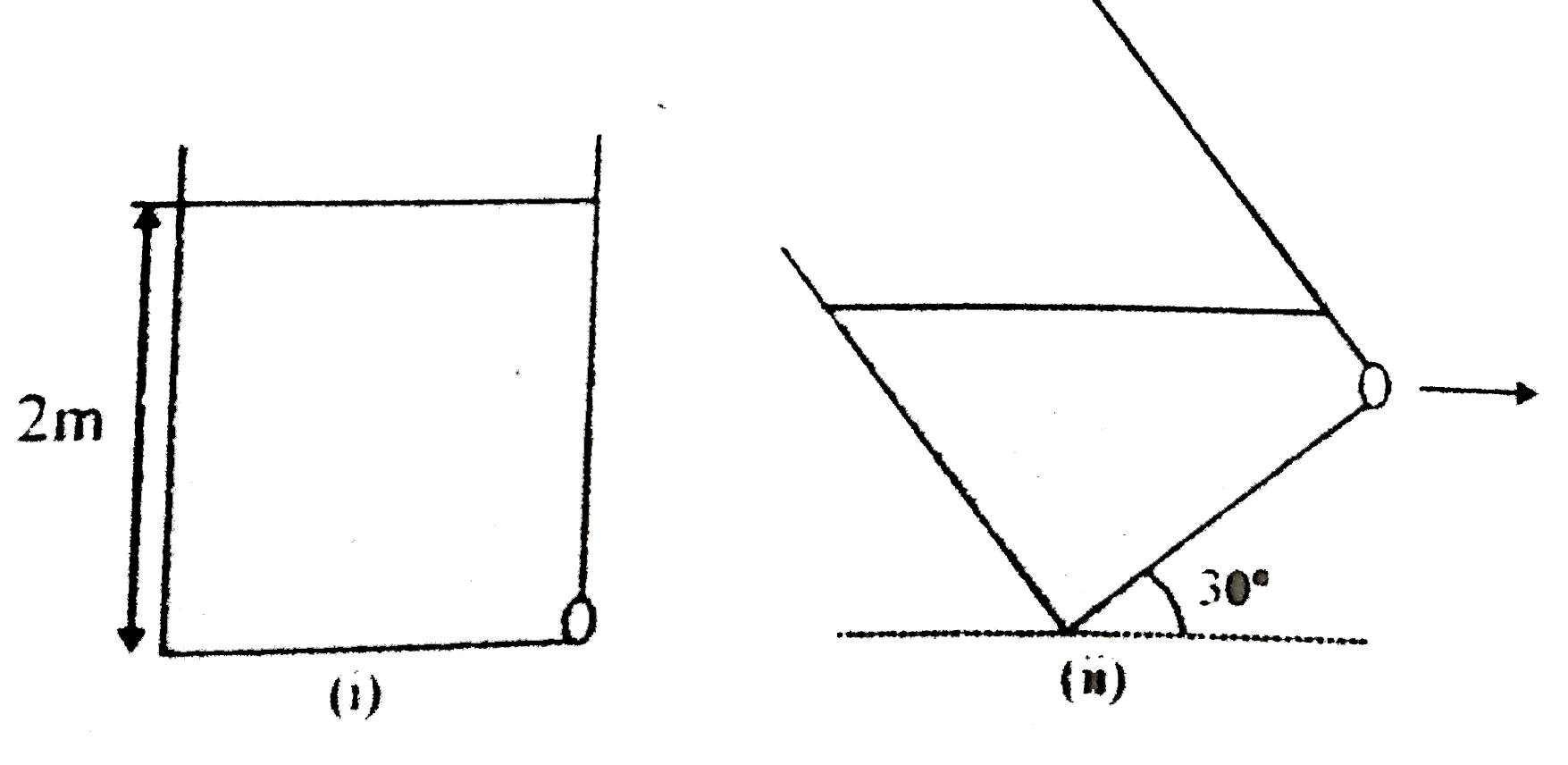
Velocity of efflux in torricelli's theorem is given by v=√2gh, here h is the height of hole from the top surface, after that, motion of liquid can be treated as projectile motion. Liquid is filled in a vessel of square base (2m×2m) upto a height of 2m as shown in figure (i). in figure (ii) the vessel is tilted from horizontal at 30∘ what is the velocity of efflux in this case ? Liquid does not spills out?A3.29m/sB4.96m/sC5.67m/sD2.68m/s
Theorems
View Solution_S01_074_Q01.png)
Velocity of efflux in torricelli's theorem is given by v=√2gh here h is the height of hole from the top surface, after that motion of liquid can be treated as projectile motion.
Q. At what distance from point O, will be strike on the ground?
(a). 5.24 m
(b). 6.27 m
4.93 m
(d). 3.95 m
View Solution_S01_073_Q01.png)
Velocity of efflux in torricelli's theorem is given by v=√2gh here h is the height of hole from the top surface, after that motion of liquid can be treated as projectile motion.
Q. What is its time of fall of liquid on the ground?
(a). 1√2s
(b). 1√3s
(c). 1√5s
(d). √2s
View SolutionState work-energy theorem. Prove it for a variable force.
View SolutionState Apollonius Theorem and Centroid divides circumcentre and orthocentre in 1:2
View SolutionState work-energy theorem.
View SolutionState Rolle’s theorem.
View Solution
