STATEMENT -1 : For an observer looking out through the window of a fast moving train , the nearby objects appear to move in the opposite direction to the train , while the distant objects appear to be stationary .
STATEMENT - 2 : If the observer and the object are moving at velocities →v1 and →v2 respecttively with refrence to a laboratory frame , the velocity of the object with respect to a laboratory frame , the velocity of the object with respect to the observer is →v2−→v(1) .
(a) Statement -1 is True, statement -2 is true , statement -2 is a correct explanation for statement -1
(b) Statement 1 is True , Statement -2 is True , statement -2 is NOT a correct explanation for statement -1
(c) Statement - 1 is True , Statement -2 is False
(d) Statement -1 is False, Statement -2 is True
The correct Answer is:B
Statement -1 is true . For a moving observer, the near by objects appear to move in the opposite direction at a large speed . This is because the angular speed of the near by object w.r.t. observer is large . As the object moves away the angular velocity decreases and therefore its speed seems to be less . The distant object almost remains stationary.
Statement -2 is the concept of relative velocity which states that
→v21=→v2G−→v1G
where G is the laboratory frame .
Thus both the statement are true statement - 2 is not the correct explanation of statement -1.
Topper's Solved these Questions
Similar Questions
Explain this common observation clearly : If you look out of the window of a fast moving train, the nearby tress, houses etc. seem to move rapidly in a direction opposite to the train's motion, but the distant objects (hill tops, the Moon, the starts etc.) seem to be stationary. (In fact, since you are aware that you are moving, these distant objects seem to move with you).
View SolutionWhat is the momentum of an object of mass m moving with a velocity v?
View Solution
Knowledge Check
The v-t plot of a moving object ios shown in the figure. The average velocity of the object during the first 10 s is
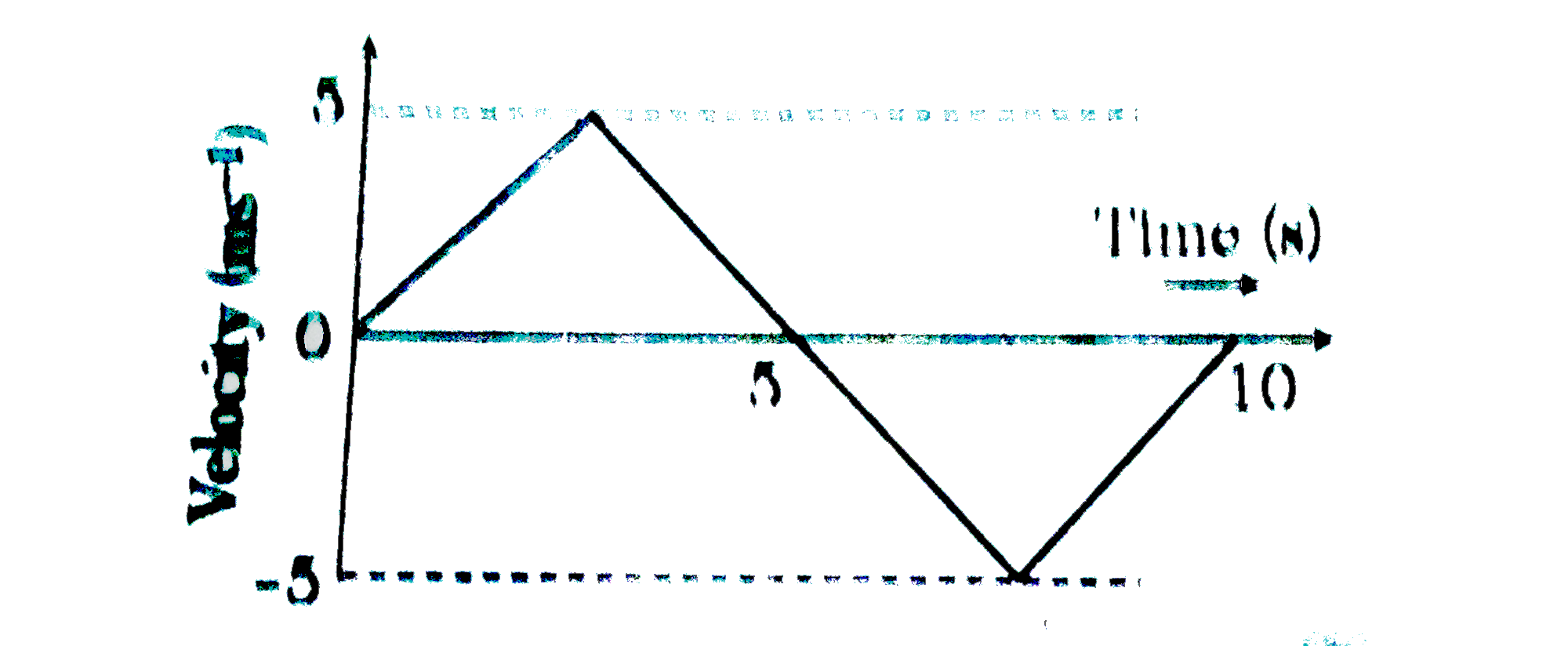 AzeroB2.5ms−1C5ms−1D2ms−1
AzeroB2.5ms−1C5ms−1D2ms−1An object may appear to be moving to one person and the same object may appear to be at rest to another person. This statement is
Aalways correctBalways falseCsometimes correct and sometimes falseDcannot say.Two objects A and B are moving in opposite directions with velocities vAandvB respectively, the magnitude of relative velocity of A w.r.t. B is
AvA−vBBvA+vBCvB−vADvA/vB
Explain this common observation clearly : If you look out of the window of a fast moving train, the nearby tress, houses etc. seem to move rapidly in a direction opposite to the train's motion, but the distant objects (hill yops, the Moon, the starts etc.) seem to be stationary. (In fact, since you are aware that you are moving, these distant objects seem to move with you).
View SolutionExplain why, if we look at objects through the hot air over a fire, the objects appear to be moving (or shaking) slightly.
View SolutionWhat is the momentum of an object of mass m , moving with a velocityv ?
View SolutionThe momentum of an object of mass 'm' moving with a velocity 'v' is given by
View SolutionA man is sitting inside a moving train and observes the stationary objects outside of the train. Then choose the single correct choice from the following statements−
View Solution
