The acceleration of a bus is given by ax(t)=at, where a=1.2ms−2.
If the bus's velocity at time t=1.0s is 5.0ms−1, what is its velocity at time t=2.0s?
To find the velocity of the bus at time t=2.0s, we can use the given acceleration function and the initial velocity. Let's break down the solution step by step.
Step 1: Understand the given information
We know:
- The acceleration of the bus is given by ax(t)=at, where a=1.2m/s2.
- The initial velocity at t=1.0s is V1=5.0m/s.
- We need to find the velocity at t=2.0s.
Step 2: Set up the equation for velocity
The final velocity can be found using the formula:
V2=V1+∫t2t1ax(t)dt
where t1=1.0s and t2=2.0s.
Step 3: Substitute the acceleration function
Substituting the acceleration function into the integral:
V2=V1+∫21atdt
Substituting a=1.2m/s2:
V2=5.0+∫211.2tdt
Step 4: Calculate the integral
To calculate the integral:
∫1.2tdt=1.2⋅t22=0.6t2
Now, evaluate the definite integral from t=1 to t=2:
∫211.2tdt=0.6(22)−0.6(12)=0.6(4)−0.6(1)=2.4−0.6=1.8
Step 5: Substitute back to find V2
Now substitute the result of the integral back into the equation for V2:
V2=5.0+1.8=6.8m/s
Final Answer
The velocity of the bus at t=2.0s is V2=6.8m/s.
---
We know:
- The acceleration of the bus is given by ax(t)=at, where a=1.2m/s2.
- The initial velocity at t=1.0s is V1=5.0m/s.
- We need to find the velocity at t=2.0s.
The final velocity can be found using the formula:
V2=V1+∫t2t1ax(t)dt
where t1=1.0s and t2=2.0s.
Substituting the acceleration function into the integral:
V2=V1+∫21atdt
Substituting a=1.2m/s2:
V2=5.0+∫211.2tdt
To calculate the integral:
∫1.2tdt=1.2⋅t22=0.6t2
Now, evaluate the definite integral from t=1 to t=2:
∫211.2tdt=0.6(22)−0.6(12)=0.6(4)−0.6(1)=2.4−0.6=1.8
Now substitute the result of the integral back into the equation for V2:
V2=5.0+1.8=6.8m/s
The velocity of the bus at t=2.0s is V2=6.8m/s.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.52videos
BASIC MATHEMATICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Integer4videos
ARCHIVES 2 VOLUME 6
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
Similar Questions
The acceleration of a motorcycle is given by ax(t)=At−Bt2, where A=1.50ms−3 and B=0.120ms−4. The motorcycle is at rest at the origin at time t=0.
a. Find its position and velocity as funcitons of time.
b. Calculate the maximum velocity it attains.View SolutionThe acceleration of a particle is given by a=t3−3t2+5, where a is in ms−2 and t is in second. At t=1s, the displacement and velocity are 8.30m and 6.25ms−1, respectively. Calculate the displacement and velocity at t=2s.
View Solution
Knowledge Check
- Question 1 - Select One
The position of an object moving along x-axis is given by x=a+bt2, where a=8.5m and b=2.5 ms^(-2) and (t) is measured in seconds. What is the velocity at t=1= 0sandt=2.0 s? What is the average velocity between t=2.0s and t=4.0s? a) 5 m/s b) 10 m/s c) 15 m/s d) 20 m/s
A5ms−1B10ms−1C15ms−1D20ms−1 - Question 2 - Select One
The position of and object moving along x-axis is given by x=a+bt2, where a=8.5m and b=2.5 ms^(-2) and (t) is measured in seconds. What is the velocity at t=0s and t=2.0s? What is the average velocity between t=2.0s and t=4.0s?
A5ms−1B10ms−1C15ms−1D20ms−1
The acceleration time graph of a particle is shown in the Fig. 2 (CF). 11. At time t=0s velocity of the particle is 8ms−1. Its velocity t=10s is.
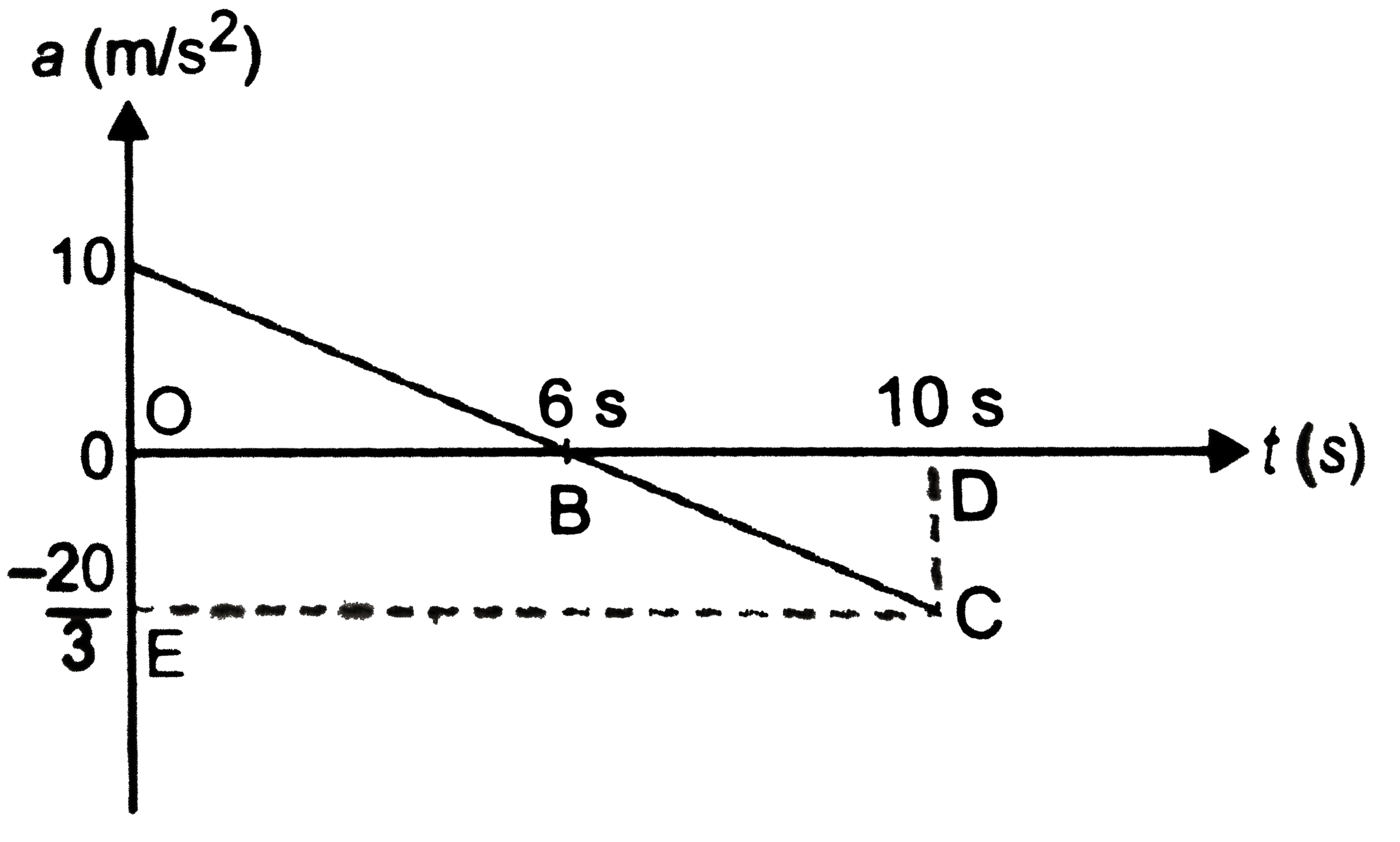 .View Solution
.View SolutionAcceleration of a particle is given by
a=3t2+2t+1
Where t is time . Find
(i) its velocity at time t assuming velocity to be 10 m/s at t = 0.
(ii) its position at time t assuming that the particle is at origin at t = 0.
(iii) What is the average speed of the particle in the duration t = 0 to t = 1s?
(iv) What is the average acceleration of the particle in the duration t = 0 to t = 1s?View SolutionThe acceleration a in ms−2 of a particle is given by a=3t2+2t+2, where t is the time. If the particle starts out with a velocity v=2ms−1 at t=0, then find the velocity at the end of 2s.
View SolutionThe position of and object moving along x-axis is gi en by x=a+bt2, wher a=8.5m and b=2.5 ms^(-2) and (t) is measured in ceconds. What is the velcoity att=1=0s and t=2.0s? What is the average velocity between t=2.0s and t=4.0s?
View SolutionAcceleration of a particle is given by
a=1/V
Where V is the velocity . Find
(i) Velocity of particle at time t, given that v = 10 m/s at t = 0.
(ii) Velocity of particle when its position is x, given that speed is 3 m/s at x = 0.View SolutionAcceleration - time graph of a particle moving in a straight line is shown in figure. Velocity of particle at time t = 0 is 2ms−1. Find velocity at the end of fourth second.
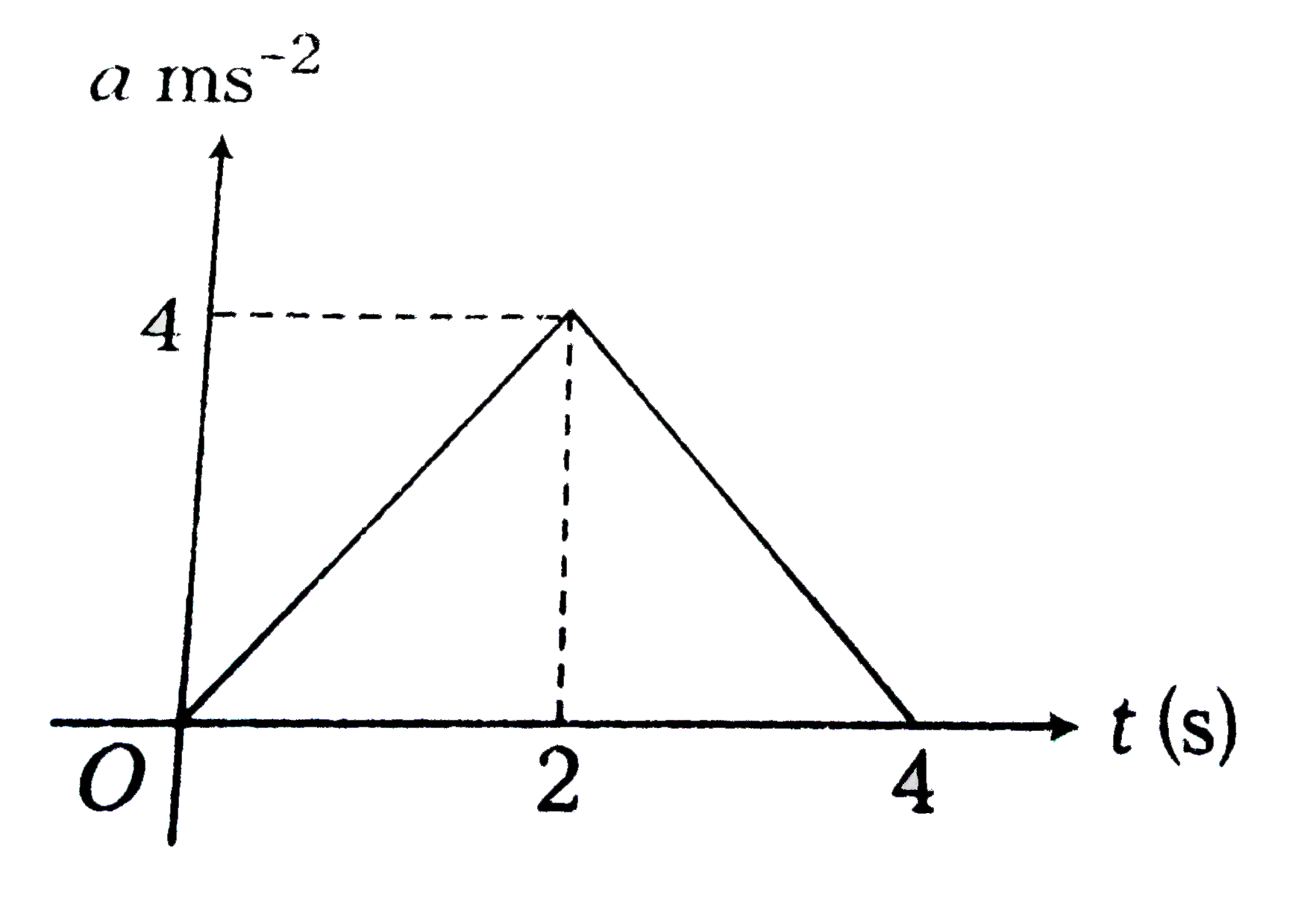 View Solution
View Solution
