The annular disc of copper, with inner radius a and outer radius b is rotating with a uniform angular speed ω, in a region where a uniform magnetic field B along the axis of rotation exists. Then, the emf induced between inner side and the outer rim of the disc is-
Zero
12Bωa2
12Bωb2
12Bω(b2−a2)
Step by step video & image solution for The annular disc of copper, with inner radius a and outer radius b is rotating with a uniform angular speed omega, in a region where a uniform magnetic field B along the axis of rotation exists. Then, the emf induced between inner side and the outer rim of the disc is- by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
A conducting disc of radius r rotates with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
View SolutionA metallic rod of length lis hinged at one end and the other end is rotated with a constant angular speed ω about an axis passing through the hinge. A constant and uniform magnetic field B, parallel to the axis of rotation exists everywhere. The induced emf between the two ends of rod is 12Blω2.
View Solution
Knowledge Check
- Question 1 - Select One
A circular metal plate of radius R is rotating with a uniform angular velocity ω with its plane perpendicular to a uniform magnetic field B. Then the emf developed between the centre and the rim of the plate is :
AπωBR2BωBR2CπωBR22DωBR2/2 - Question 2 - Select One
A circular metal plate of radius R is rotating with a uniform angular velocity ω with its plane perpendicular to a uniform magnetic field B. Then the emf developed between the centre and the rim of the plate is :
AπωBR2BωBR2CπωBR22DωBR2/2 - Question 3 - Select One
A conducting rod of length 2l is rotating with constant angular speed w about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
 ABωl2B12Bωl2C18Bωl2Dzero
ABωl2B12Bωl2C18Bωl2Dzero
A rod of length l rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the centre of the rod and an end is
View SolutionA conducting rod of length 2l is rotating with constant angular speed ω about is perpendicular bisector. A uniform magnetic field →B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
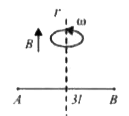 View Solution
View SolutionA non-conducting disk of radius R is rotating about its own axis with constant angular velocity ω in a perpendicular uniform magnetic field B as shown in figure. The emf induced between centre and rim of disk is
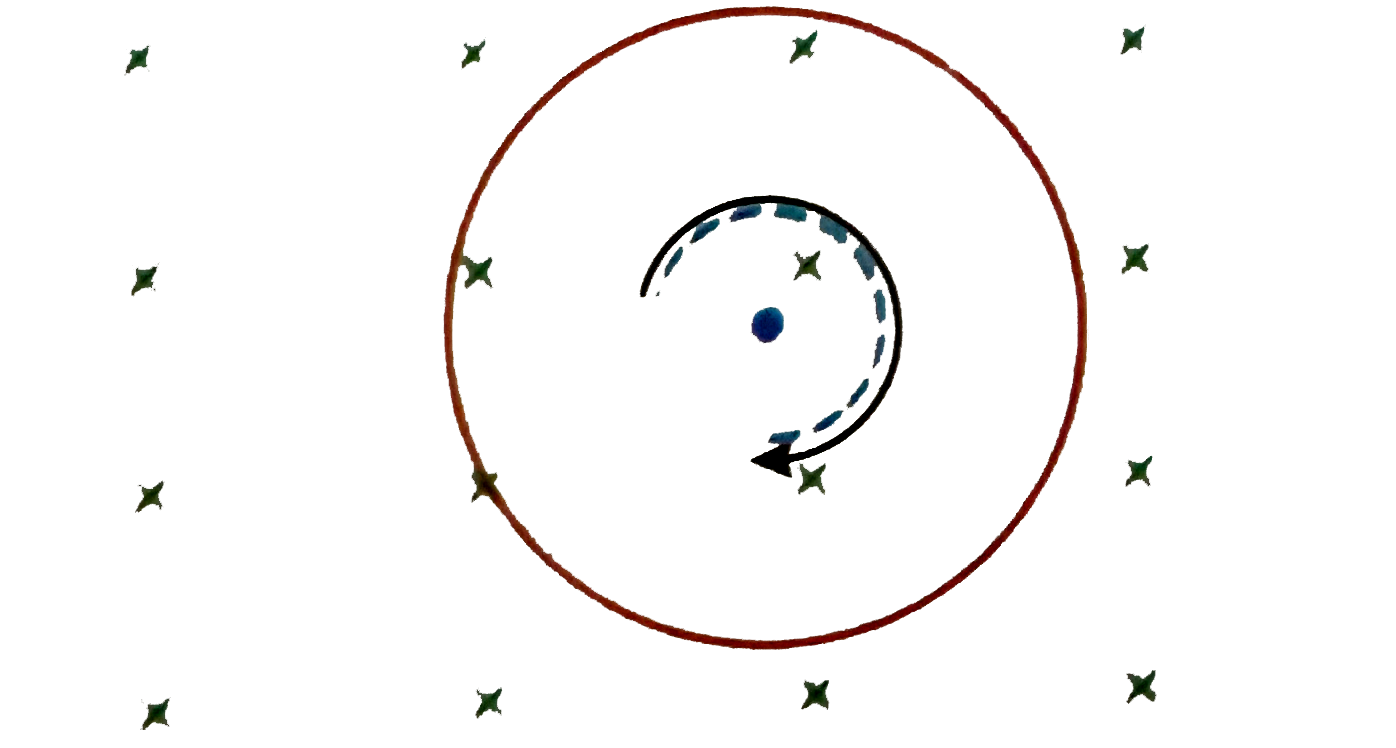 View Solution
View SolutionA rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is
View SolutionA coil of N turns and mean area A is rotating with uniform angular velocity ω about an axis ai right angles to a uniform magnetic field B. The induced emfe in the coil will be
View Solution

