The density of a liquid varies with depth h from the free surface as . A small body of density is released from the surface of liquid. The body will
come to a momentary rest at a depth from the free surface
execute S.H.M. about a point at a depth from the surface
execute S.H.M. of amplitude
all of these
The correct Answer is:D
Let V be the volume of body which is released on the surface of liquic. When body reaches at depth x from the surface of liquid, then upward thrust on body due to liquid
Weight of body
At equilibrium ,
If the body comes to rest at depth h, then work done on body by the upward thrust dur to liquid
change in potential energy of body
Now, the body will move upwards towards equilibrium position due to displacemtn x from equilibrium position . Hence the body will execute S.H.M. Choice (d) is right.
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBook:PRADEEPChapter:OSCILLATIONS AND WAVESExercise:integer type question
Explore 8 Videos
- OSCILLATIONS AND WAVESBook:PRADEEPChapter:OSCILLATIONS AND WAVESExercise:Assertion-Reason Type Questions
Explore 23 Videos
- OSCILLATIONS AND WAVESBook:PRADEEPChapter:OSCILLATIONS AND WAVESExercise:Multiple Choice Question-II
Explore 14 Videos
- MATHEMATICAL TOOLSBook:PRADEEPChapter:MATHEMATICAL TOOLSExercise:Fill in the blanks
Explore 5 Videos
- PHYSICAL WORLD AND MEASUREMENTBook:PRADEEPChapter:PHYSICAL WORLD AND MEASUREMENTExercise:Competiton Focus Jee Medical Entrance
Explore 18 Videos
Similar Questions
(a). Discuss two situations in daily life where we apply the concept of pressure.
(b). A body of density ρ is dropped gently on the surface of a liquid of density ρ' (ρ' being less than ρ). Show That it will reach the bottom of the liquid after a time √2dρg(ρ−ρ'), where g is acceleration due to gravity and d is the depth of the liquid.
View SolutionA stream line body with relative density ρ1 falls into air from a height h1 on the surface of a liquid of realtive density ρ2, where ρ2>ρ1. Find the time of immersion of the body into the liquid.
View Solution
Knowledge Check
Density of a liquid varies with depth as ρ=αh. A small ball of density ρ0 is released from the free surface of the liquid. Then
Athe ball will execute SHM of amplitude ρ0αBthe mean position of the ball will be at a depth ρ02αCthe ball will sink to a maximum depth of ρ0αDThe ball sink to a maximum depth of ρ0αA ball of density ρ is released from deep inside of a liquid of density 2ρ. It will move up
Awith an increasing accelerationBwith a decreasing accelerationCwith a constant accelerationDwith zero accelerationA uniform solid sphere of radius R is in equilibrium inside a liquid whose density varies with depth from free surface as ρ=ρ0(1+hh0) where h is depth from free surface. Density of sphere σ will be :
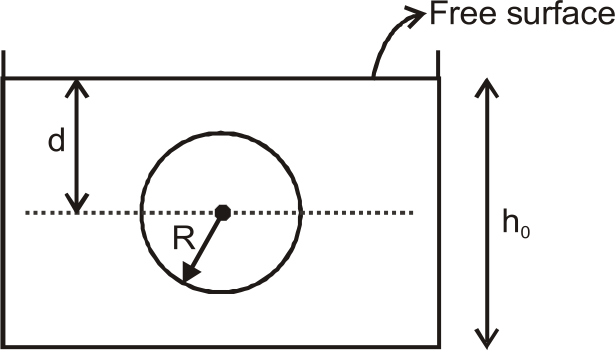 Aσ=ρ0(1+d2h0)Bσ=ρ0(1−d2h0)Cσ=ρ0(1+2dh0)Dσ=ρ0(1+dh0)
Aσ=ρ0(1+d2h0)Bσ=ρ0(1−d2h0)Cσ=ρ0(1+2dh0)Dσ=ρ0(1+dh0)
The escape velocity from the surface of the earth of radius R and density ρ
View SolutionThe pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
View SolutionCalculate the pressure inside a small air bubble of radus r situated at a depth h below the free surface of liquids of densities ρ1 and ρ2 and surface tennsions T1 and T2. The thickness of the first and second liquids are h1 and h2 respectively. Take atmosphere pressure =P0.
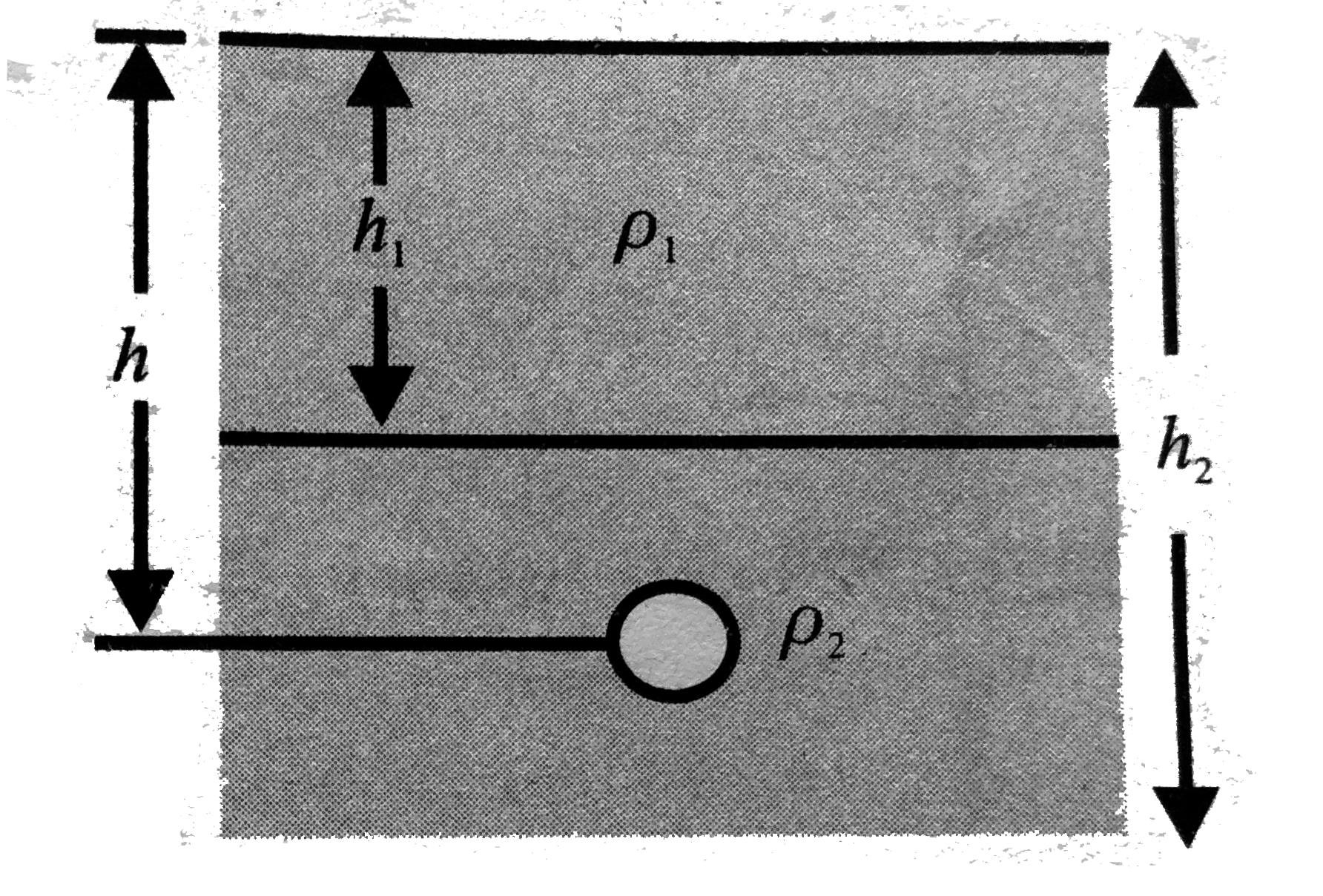
View SolutionThe density ρ of water of buk modulus B at a depth y then ocean is related to the density at surface ρ0 by the relation
View SolutionA liquid of density ρ is filled in a vessel up to height H and a hole of cross section area A is made at a depth h below the free surface of liquid. The speed of liquid coming out of the hole is independent of
View Solution