The displacement of a particle in simple harmonic motion in one time period is
A
2A
4A
zero
The correct Answer is:D
Step by step video, text & image solution for The displacement of a particle in simple harmonic motion in one time period is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Objective 2
14 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Objective-2
2 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Short Answer
13 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Exercises
32 Videos
Similar Questions
Figure shows the graph of velocity versus displacement of a partciel executing simple harmonic motion. Find the period of oscillation of the particle.
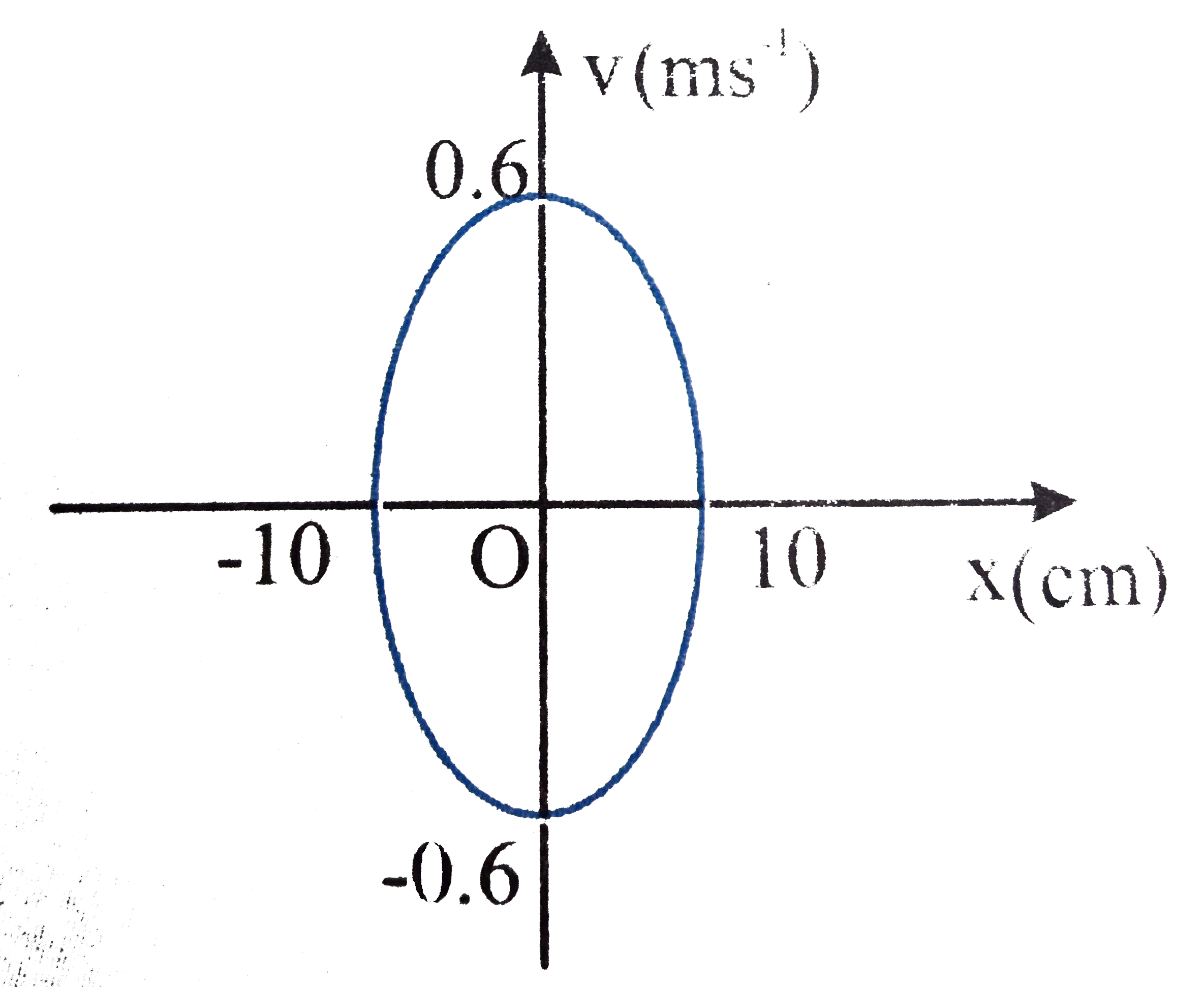
View SolutionThe plot of velocity (v) versus displacement (x) of a particle executing simple harmonic motion is shown in figure. The time period of oscillation of particle is :-

View Solution
Knowledge Check
The distance moved by a particle in simple harmonic motion in one time period is
AAB2AC4ADzeroIf x, and a denote the displacement, the velocity and the acceler of a particle executing simple harmonic motion of time period T, then, which of the following does not change with time?
A(a) aT/xB(b) aT+2πvC(c ) aT/vD(d) a2T2+4π2v2If x,v and a denote the displacement, the velocity and the acceleration of a particle executing simple harmonic motion of time period T, then, which of the following does not change with time ?
AaTxBaT+2πvCaTvDa2T2+4π2v2
The velocity of a particle executing simple harmonic motion is
View SolutionOn the basis of dimensional, decide which of the following relation for the displacement of a particle undergoing simple harmonic motion is not correct :
View SolutionThe ratio of the maximum velocity and maximum displacement of a particle executing simple harmonic motion is equal to
View SolutionOn the basis of dimensions, decide which of the following relation for the displacement of a particle undergoing simple harmonic motion is not correct :
View SolutionThe phase (at a time t) of a particle in simple harmonic motion tells
View Solution
