The element of an electric heater is in the form of a coil. Once it is heated by dc volatage and then by ac voltage of equal potential difference. Will the production of heat in both cases be same of different?
To determine whether the production of heat in an electric heater coil is the same when heated by DC voltage and AC voltage of equal potential difference, we can analyze the situation step by step.
1. Understanding Heat Production:
The heat produced in a resistor (or coil) can be calculated using the formula:
H=I2RT
where H is the heat produced, I is the current, R is the resistance, and T is the time for which the current flows.
2. Resistance:
In both cases (DC and AC), the resistance R of the coil remains the same since it is a property of the material and geometry of the coil.
3. Current in DC Circuit:
When a DC voltage V is applied, the current IDC can be calculated using Ohm's law:
IDC=VR
Thus, the heat produced by the DC current is:
HDC=I2DCRT=(VR)2RT=V2RT
4. Current in AC Circuit:
When an AC voltage V is applied, the situation is different due to the presence of inductance in the coil. The total impedance Z in an AC circuit is given by:
Z=√R2+(Lω)2
where L is the inductance of the coil and ω is the angular frequency of the AC source.
5. Calculating AC Current:
The current IAC in the AC circuit is:
IAC=VZ
Since Z>R (due to the inductive component), it follows that:
IAC<IDC
6. Heat Produced by AC Current:
The heat produced by the AC current can be calculated as:
HAC=I2ACRT=(VZ)2RT
Since Z>R, we can conclude that:
HAC<HDC
7. Conclusion:
The heat produced when the coil is heated by DC voltage will be greater than the heat produced when it is heated by AC voltage of the same potential difference. Therefore, the production of heat in both cases will be different.
Summary:
- Heat produced by DC: HDC=V2RT
- Heat produced by AC: HAC=(VZ)2RT where Z>R
- Final Result: HDC>HAC
The heat produced in a resistor (or coil) can be calculated using the formula:
H=I2RT
where H is the heat produced, I is the current, R is the resistance, and T is the time for which the current flows.
In both cases (DC and AC), the resistance R of the coil remains the same since it is a property of the material and geometry of the coil.
When a DC voltage V is applied, the current IDC can be calculated using Ohm's law:
IDC=VR
Thus, the heat produced by the DC current is:
HDC=I2DCRT=(VR)2RT=V2RT
When an AC voltage V is applied, the situation is different due to the presence of inductance in the coil. The total impedance Z in an AC circuit is given by:
Z=√R2+(Lω)2
where L is the inductance of the coil and ω is the angular frequency of the AC source.
The current IAC in the AC circuit is:
IAC=VZ
Since Z>R (due to the inductive component), it follows that:
IAC<IDC
The heat produced by the AC current can be calculated as:
HAC=I2ACRT=(VZ)2RT
Since Z>R, we can conclude that:
HAC<HDC
The heat produced when the coil is heated by DC voltage will be greater than the heat produced when it is heated by AC voltage of the same potential difference. Therefore, the production of heat in both cases will be different.
- Heat produced by DC: HDC=V2RT
- Heat produced by AC: HAC=(VZ)2RT where Z>R
- Final Result: HDC>HAC
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Exercises Subjective
15 Videos
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Exercises Single Correct
63 Videos
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Solved Examples
6 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - ddp.4.3
15 Videos
Similar Questions
Two heater wires, made of the same material and having the same length and the same radius, are first connected in series and then in parallel to a constant potential difference. If the rate of heat produced in the two cases are Hs and Hp respectively then HsHp will be
View SolutionPlot a graph comparing the variation of potential ‘V’ and electric field ‘E’ due to a point charge ‘Q’ as a function of distance r from point charge
(ii) Find the ratio of the potential differences that must be applied across the parallel and the series combination of two identical capacitors so that the energy stored, in the two cases, becomes the same.
View Solution
Knowledge Check
(a) Estimate the speed with which electrons emitted from a heated cathode of an evacuated tube impinge on the anode maintained at a potential difference of 500 V with respect to the cathode. Ignore the small initial speeds of the electrons. The specific charge to of the electron, i.e., its e/m is given to 1.76×1011Ckg−1.
(b) Use the same formula you employ in (a) to obtain electron speed for an anode potential of 10 MV. Do you see what is wrong? In what way is the formula to be modified?A1.33×106ms−1B1.33×107ms−1C2.66×107ms−1D2.66×106ms−1
(a) Estimate the speed with which electrons emitted from a heated cathode of an evacuated tube impinge on the anode maintained at a potential difference of 500 V with respect to the cathode. Ignore the small initial speeds of the electrons. The specific charge to of the electron, i.e., its e/m is given to 1.76×1011Ckg−1.
(b) Use the same formula you employ in (a) to obtain electron speed for an anode potential of 10 MV. Do you see what is wrong? In what way is the formula to be modified?
View SolutionAnswer the following:
(i) Use Gauss' law to find the electric field due to a uniformly charged infinite plane sheet. What is the direction of field for positive and negative charge densities?
(ii) Find the ratio of the potential differences that must be applied across the parallel and series combination of two capacitors C1 and C2 with their capacitance in the ratio 1:2 so that the energy stored in the two cases becomes the same.
View SolutionThe circuit shown in the figure is a series LCR circuit connected to an AC source. This circuit has many important applications in practical electronic systems. In the given circuit, L=20mH and frequency of applied alternating potential difference is ω=8000rad/s. Initial phase of applied potential difference has such a minimum value that initial value of instantaneous potential difference is half of is peak value while initial phase of current in the circuit has such a minimum value that initial value of instantaneous current equals its peak value. We also observe that current in the circuit leads the net voltage across the circuit. It is given that peak values of potential difference across R and L are I VR(peak))=25V
V(L)peak=20V
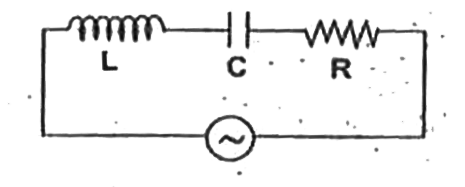
Phase difference between current in the circuit and voltage in the circuit
View SolutionThe circuit shown in the figure is a series LCR circuit connected to an AC source. This circuit has many important applications in practical electronic systems. In the given circuit, L=20mH and frequency of applied alternating potential difference is ω=8000rad/s. Initial phase of applied potential difference has such a minimum value that initial value of instantaneous potential difference is half of is peak value while initial phase of current in the circuit has such a minimum value that initial value of instantaneous current equals its peak value. We also observe that current in the circuit leads the net voltage across the circuit. It is given that peak values of potential difference across R and L are I VR(peak))=25V
V(L)peak=20V

rms value of potential difference across capacitor is nearly
View SolutionThe circuit shown in the figure is a series LCR circuit connected to an AC source. This circuit has many important applications in practical electronic systems. In the given circuit, L=20mH and frequency of applied alternating potential difference is ω=8000rad/s. Initial phase of applied potential difference has such a minimum value that initial value of instantaneous potential difference is half of is peak value while initial phase of current in the circuit has such a minimum value that initial value of instantaneous current equals its peak value. We also observe that current in the circuit leads the net voltage across the circuit. It is given that peak values of potential difference across R and L are I VR(peak))=25V
V(L)peak=20V

Instantaneous value of potential difference applied
View SolutionThe circuit shown in the figure is a series LCR circuit connected to an AC source. This circuit has many important applications in practical electronic systems. In the given circuit, L=20mH and frequency of applied alternating potential difference is ω=8000rad/s. Initial phase of applied potential difference has such a minimum value that initial value of instantaneous potential difference is half of is peak value while initial phase of current in the circuit has such a minimum value that initial value of instantaneous current equals its peak value. We also observe that current in the circuit leads the net voltage across the circuit. It is given that peak values of potential difference across R and L are I VR(peak))=25V
V(L)peak=20V

Instantaneous potential difference across the inductor can be expressed as
View SolutionOxidation number is the charge which an atom of an element has in its ion or appears to have when present in the combined state. It is also called oxidation state. Oxidation number of any atom in the elementary state is zero. Oxidation number of a monoatomic ion is equal to the charge on it. In compounds of metals with non metals, metals have positive oxidation number while non metals have negative oxidation numbers. In compounds of two difference elements, the more electronegative element has negative oxidation number whereas the other has positive oxidation number. In complex ions, the sum of the oxidation number of all the atoms is equal to the charge on the ion. If a compound contains two or more atoms of the same element, they may have same or different oxidation states according as their chemical bonding is same or different.
The oxidation state of the most electronegative element in the products of the reaction between BaO2 and H2SO4 are
View Solution
