The equivalent resistance of network of three 4Ω resistors can not be
12Ω
1.33Ω
6Ω
4Ω
The correct Answer is:D
To determine the equivalent resistance of a network of three 4-ohm resistors, we will analyze different configurations: series, parallel, and a combination of both.
1. Understanding Series Connection:
- In a series connection, the total or equivalent resistance (R_eq) is the sum of the individual resistances.
- Formula:
Req=R1+R2+R3
- For three 4-ohm resistors:
Req=4Ω+4Ω+4Ω=12Ω
- Conclusion: The equivalent resistance can be 12 ohms.
2. Understanding Parallel Connection:
- In a parallel connection, the total or equivalent resistance is given by:
- Formula:
1Req=1R1+1R2+1R3
- For three 4-ohm resistors:
1Req=14Ω+14Ω+14Ω=34Ω
- Therefore,
Req=43Ω≈1.33Ω
- Conclusion: The equivalent resistance can be approximately 1.33 ohms.
3. Combination of Series and Parallel:
- We can also have combinations of series and parallel connections. For example, if two resistors are in parallel and one is in series:
- First, calculate the equivalent resistance of the two resistors in parallel:
Rparallel=1(14+14)=42=2Ω
- Then, add the series resistor:
Req=Rparallel+R=2Ω+4Ω=6Ω
- Conclusion: The equivalent resistance can also be 6 ohms.
4. Final Consideration:
- We have found the possible equivalent resistances:
- 12 ohms (series)
- 1.33 ohms (parallel)
- 6 ohms (two in parallel and one in series)
- The only value that cannot be achieved with any configuration of three 4-ohm resistors is 4 ohms.
Final Answer:
The equivalent resistance of a network of three 4-ohm resistors cannot be 4 ohms.
- In a series connection, the total or equivalent resistance (R_eq) is the sum of the individual resistances.
- Formula:
Req=R1+R2+R3
- For three 4-ohm resistors:
Req=4Ω+4Ω+4Ω=12Ω
- Conclusion: The equivalent resistance can be 12 ohms.
- In a parallel connection, the total or equivalent resistance is given by:
- Formula:
1Req=1R1+1R2+1R3
- For three 4-ohm resistors:
1Req=14Ω+14Ω+14Ω=34Ω
- Therefore,
Req=43Ω≈1.33Ω
- Conclusion: The equivalent resistance can be approximately 1.33 ohms.
- We can also have combinations of series and parallel connections. For example, if two resistors are in parallel and one is in series:
- First, calculate the equivalent resistance of the two resistors in parallel:
Rparallel=1(14+14)=42=2Ω
- Then, add the series resistor:
Req=Rparallel+R=2Ω+4Ω=6Ω
- Conclusion: The equivalent resistance can also be 6 ohms.
- We have found the possible equivalent resistances:
- 12 ohms (series)
- 1.33 ohms (parallel)
- 6 ohms (two in parallel and one in series)
- The only value that cannot be achieved with any configuration of three 4-ohm resistors is 4 ohms.
The equivalent resistance of a network of three 4-ohm resistors cannot be 4 ohms.
Topper's Solved these Questions
- MISCELLANEOUS QUESTIONSBOOK - MCGROW HILL PUBLICATIONCHAPTER - MISCELLANEOUS QUESTIONSEXERCISE - PART-B
99 Videos
- MEASUREMENTBOOK - MCGROW HILL PUBLICATIONCHAPTER - MEASUREMENTEXERCISE - ELEMENTARY QUESTIONS (HIGHER ORDER THINKING QUESTIONS)
13 Videos
- MOTIONBOOK - MCGROW HILL PUBLICATIONCHAPTER - MOTIONEXERCISE - HIGHER ORDER THINKING QUESTIONS
48 Videos
Similar Questions
Establish a relationship to determine the equivalent resistance R of a combination of three resistors having resistances R1,R2andR3 connected in series.
(b) Calculate the equivalent resistance R of a combination of three resistors of 2Ω,3Ωand6Ω joined in parallel.
View SolutionFind an expression for the equivalent resistance of three resistors joined in series.
View Solution
Knowledge Check
The equivalent resistance of network of three 4Ω resistors can not be
A12ΩB1.33ΩC6ΩD4ΩThe equivalent resistance of network of three 2Ω resistors can not be
A0.67B2ΩC3ΩD6ΩThe equivalent resistance of three equal resistances connected in series is 27Ω. There equivalent resistance when connected in parallel is
A54ΩB270ΩC3ΩD9Ω
Twelve equal resistors each R Ω are connected to from the edges of a cube . Find the equivalent resistance of the network.
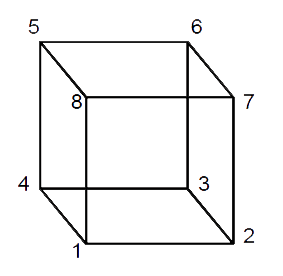
When current enters at 1 and leaves at 2
View SolutionTwelve equal resistors each R Ω are connected to from the edges of a cube . Find the equivalent resistance of the network.
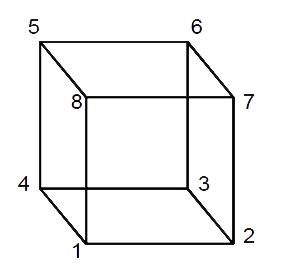
When current enters at 1 and leaves at 3
View SolutionFind an expression for equivalent resistance of three resistors joined in parallel.
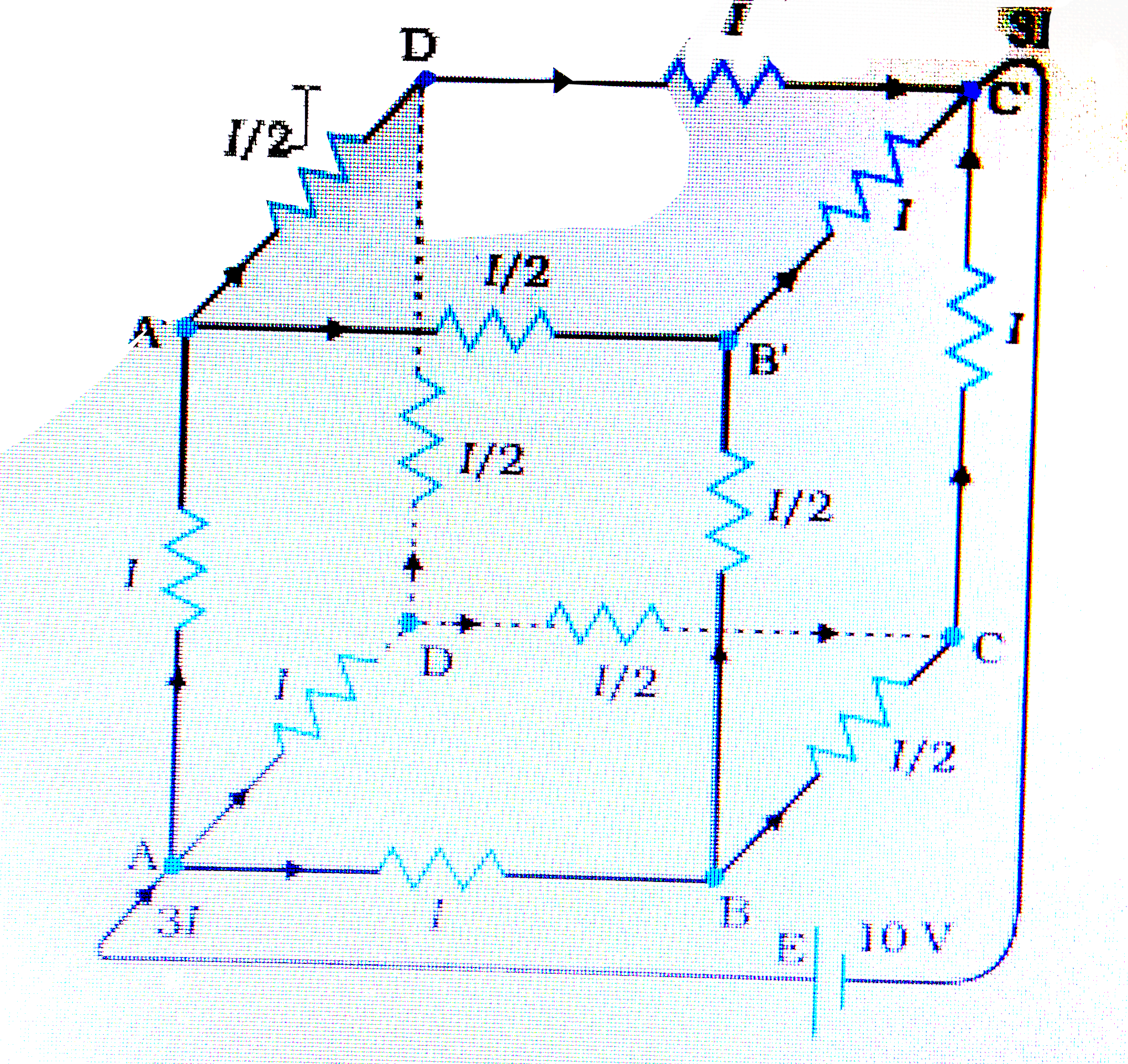
View SolutionBattery of 10 V and negligible internal resistance is connected across the diagonally opposite corners of a cubical network consisting of 12 resistors each of resistance 1Ω (Fig. 3.23). Determine the equivalent resistance of the network and the current along each edge of the cube.
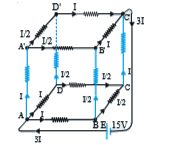
View SolutionA battery of 15 V and negligible internal resistance is connected across the diagonally opposite corners of a cubical network consisting of 12 resistors each of resistance 5Ω 22. Determine the equivalent resistance of the network and the current along each edge of the cube.
View Solution
