The force constant of a weightless spring is 16Nm−1. A body of mass 1.0kg suspended from it is pulled down through 5cm and then released. The maximum energy of the system (spring + body) will be
2×10−2J
4×10−2J
8×10−2J
16×10−2J
The correct Answer is:A
ω=√Km=√161=4rads−1
Now, Kmax=12mω2A2
=12×1×(4)2×(5×10−2)2
=2×10−2J
Topper's Solved these Questions
- OSCILLATIONSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - OSCILLATIONSEXERCISE - EXERCISE 2
49 Videos
- OSCILLATIONSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - OSCILLATIONSEXERCISE - MHT CET CORNER
29 Videos
- OSCILLATIONSBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - OSCILLATIONSEXERCISE - MHT CET CORNER
29 Videos
- MOCK TEST 5BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - MOCK TEST 5EXERCISE - MCQs
42 Videos
- PRACTICE SET 01BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - PRACTICE SET 01EXERCISE - PAPER 1 (PHYSICS & CHEMISTRY)
50 Videos
Similar Questions
Force constant of a weightless spring is 16 N/m. A body of mass 1.0 kg suspended from it is pulled down through 5 cm from its mean position and the released. The maximum kinetic energy of the system (spring+body) will be (0.0x) J. Find value of x.
View SolutionA spring has a natural length of 50 cm and a force constant of 2.0×103 Nm−1. A body of mass 10 kg is suspended from it and the spring is stretched. If the body is pulled down to a length of 58 cm and released, it executes simple harmonic motion. The net force on the body when it is at its lowermost position of its oscillation is (10x) newton. Find value of x. (Take g=10m/s2)
View Solution
Knowledge Check
The force constant of a weightless spring is 16Nm−1. A body of mass 1.0kg suspended from it is pulled down through 5cm and then released. The maximum energy of the sysytem (spring + body) will be
A2×102JB4×102JC8×102JD16×102JForce constant of a weightless spring is 16Nm−1. A body if mass 1 kg suspended from it is pulled down through 5 cm from its mean position and then released. The maximum kinetic energy of the body will be
A2×10−2JB4×10−2JC8×10−2JD16×10−2JMass suspended to a spring is pulled down by 2.5 cm and let go. The mass oscillates with an amplitude of
A2.5 cmB5 cmC7.5 cmD10 cm
Two bodies of masses 1 kg and 3 kg respectively are connected rigidly by a vertcal spring. The body of mass 3 kg rests on a smototh horizontal surface. The force constant of the spring is 484Nm−1. From the equilibrium position, the mass of 1 kg is displaced vertically through a distance of 0.02 m and then released. calculate (i) and frequency of oscillation of the mass of 1 kg (ii) the maximum velocity of this mass (iii) its oscillation energy and (iv) the reaction of the table on the mass of 3 kg, when mass 1 kg is having harmonic oscillations.
View SolutionIf a body of mass 0.98 kg is made to oscillate on a spring of force constant 4.84 N/m the angular frequency of the body is
View SolutionA body of mass m is suspended from a massless spring of natural length l. It stretches the spring through a vertical distance y. The potential energy of the stretched spring is
View SolutionA spring of force constant 600Nm−1 is mounted on a horizontal table. A mass of 1.5 kg is attached to the free end of the spring,pulled sideways to a distance of 2 cm and released . The speed of the mass when the spring is compressed by 1 cm is
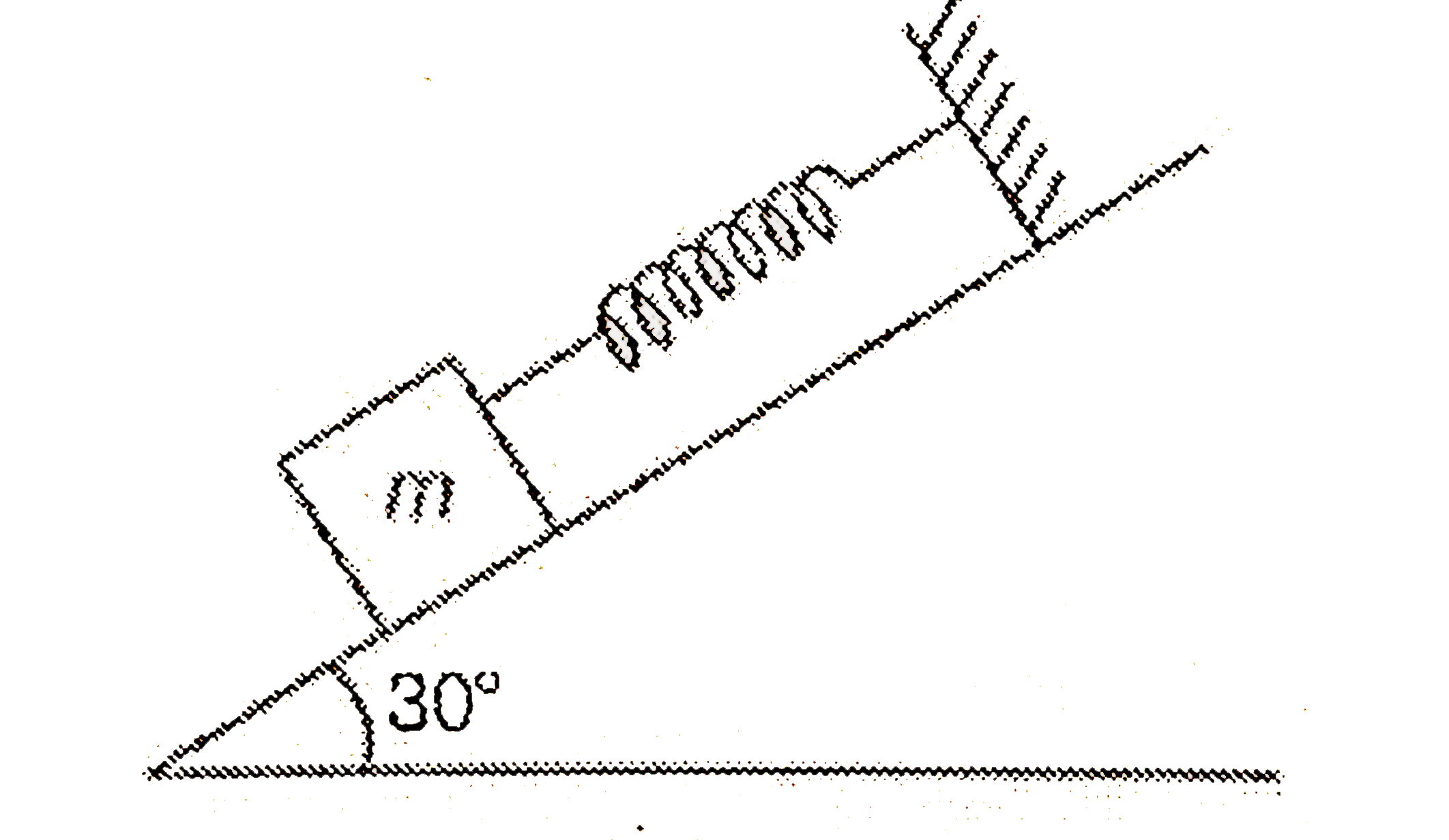
View SolutionA body of mass 5 kg is suspended by a spring balance on an inclined plane as shown in figure. The spring balance measure
View Solution
