The hale telescope of mount Polamor has a diameter of 200 inches. What is its limiting angle of resolution for 600 nm light?
7.2×10−8rad
7.2×10−4rad
1.44×10−7rad
14.4×10−10rad
The correct Answer is:C
To find the limiting angle of resolution for the Hale telescope, we will use the Rayleigh criterion formula. Here is a step-by-step solution:
Step 1: Convert the diameter from inches to meters
The diameter of the telescope is given as 200 inches. We need to convert this measurement into meters.
1. Conversion factor: 1 inch = 2.54 cm
2. Convert inches to centimeters:
200 inches×2.54 cm/inch=508 cm
3. Convert centimeters to meters:
508 cm÷100=5.08 m
Step 2: Identify the wavelength of light
The wavelength of light is given as 600 nm. We need to convert this into meters.
1. Conversion:
600 nm=600×10−9 m=6.00×10−7 m
Step 3: Apply the Rayleigh criterion formula
The limiting angle of resolution (Δθ) can be calculated using the Rayleigh criterion formula:
Δθ=1.22λD
Where:
- Δθ = limiting angle of resolution in radians
- λ = wavelength of light in meters
- D = diameter of the telescope in meters
Step 4: Substitute the values into the formula
Now, we will substitute the values of λ and D into the formula.
1. Substituting values:
Δθ=1.22×(600×10−9 m)5.08 m
Step 5: Calculate the angle of resolution
Now we will perform the calculation.
1. Calculating:
Δθ=1.22×600×10−95.08
=7.32×10−75.08≈1.44×10−7 radians
Conclusion
The limiting angle of resolution for the Hale telescope for 600 nm light is approximately 1.44×10−7 radians.
---
The diameter of the telescope is given as 200 inches. We need to convert this measurement into meters.
2. Convert inches to centimeters:
200 inches×2.54 cm/inch=508 cm
3. Convert centimeters to meters:
508 cm÷100=5.08 m
The wavelength of light is given as 600 nm. We need to convert this into meters.
600 nm=600×10−9 m=6.00×10−7 m
The limiting angle of resolution (Δθ) can be calculated using the Rayleigh criterion formula:
Δθ=1.22λD
Where:
- Δθ = limiting angle of resolution in radians
- λ = wavelength of light in meters
- D = diameter of the telescope in meters
Now, we will substitute the values of λ and D into the formula.
Δθ=1.22×(600×10−9 m)5.08 m
Now we will perform the calculation.
Δθ=1.22×600×10−95.08
=7.32×10−75.08≈1.44×10−7 radians
The limiting angle of resolution for the Hale telescope for 600 nm light is approximately 1.44×10−7 radians.
Topper's Solved these Questions
- WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -II (POLARISATION)
10 Videos
- WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -III (DOPPLER EFFECT IN LIGHT, INTERFERENCE)
25 Videos
- WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -II (INTERFERENCE)
26 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Doppler effect :)
15 Videos
Similar Questions
Light of wavelength 589 nm is used to view an object under a microscope. The aperature of the objective has a diameter of 0.900cm. Find
What effect would this have on the resolving power, if water (μ=1.33) fills the space between the object and objective.
View SolutionAn objective of a telescope has diameter 250 cm. The limit of resolution of telescope for wavelength 600 nm is nearly
View Solution
Knowledge Check
A circle with a diameter of 8 inches is divided into a number of equal sectors such that each sector has an area of 4π3 square inches. What is the central angle of each sector, in radians
Aπ12Bπ6Cπ3D2π3A 3-inch-tall rectangular box with a square base is constructed to hold a circular pie that has a diameter of 8 inches. Both are shown below. What is the volume, in cubic inches, of the smallest such box that can hold this pie?
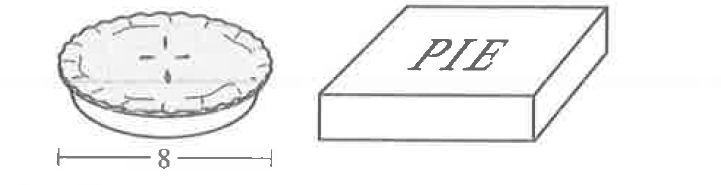 A24B64C72D192
A24B64C72D192A gaint refrecting telescope at an observatory has an objective lens of focal length 15m. If an eye piece lens of focal length 1cm is used, find the angular magnification of the telescope.
If this telescope is used to view the moon, what is the diameter of image of moon formed by objective lens ? The diameter of the moon is 3.42×106m and radius of lunar orbit is 3.8×108m.A1000B1500C2000D3000
Light of wavelength 589 nm is used to view an object under a microscope. The aperature of the objective has a diameter of 0.900cm. Find
What effect would this have on the resolving power, if water (μ=1.33) fills the space between the object and objective.
View SolutionCalculate the limit of resolution of a telescope objective having a diameter of 200cm , if it has detect light of wavelength 500nm coming from a star.
View SolutionDiameter of the objective lens of a telescope is 250 cm. For light of wavelength 600 nm coming from a distance object, the limit of resolution of the telescope is close to :
View SolutionThe diameter of the objective lens of a telescope is 5.0m and wavelength of light is 6000Å. The limit of resolution of this telescope will be
View SolutionThe wavelength of light is 589 nm. What is its wavelength in Å ?
View Solution
