The image of a needle placed 45cm from a lens is formed on a screen placed 90cm on the other side of lens. Find displacement of image if object is moved 5cm away from lens.
10cm, towards the lens
15cm, away from the lens
15cm, towards the lens
10cm, away from the lens
The correct Answer is:C
To solve the problem step by step, we will use the lens formula and the information provided in the question.
Step 1: Identify the given values
- Initial object distance (U) = -45 cm (negative because it is on the same side as the object)
- Initial image distance (V) = +90 cm (positive because it is on the opposite side of the lens)
- Object is moved 5 cm away, so the new object distance (U') = -45 cm - 5 cm = -50 cm
Step 2: Use the lens formula to find the focal length (F)
The lens formula is given by:
1F=1V−1U
Substituting the initial values:
1F=190−1−45
This simplifies to:
1F=190+145
Finding a common denominator (which is 90):
1F=190+290=390
Thus,
F=903=30 cm
Step 3: Calculate the new image distance (V') when the object is moved to U' = -50 cm
Using the lens formula again with the new object distance:
1F=1V′−1U′
Substituting the values:
130=1V′−1−50
This simplifies to:
130=1V′+150
Rearranging gives:
1V′=130−150
Finding a common denominator (which is 150):
1V′=5150−3150=2150
Thus,
V′=1502=75 cm
Step 4: Calculate the displacement of the image
The displacement of the image is the difference between the initial image distance and the new image distance:
Displacement=V−V′=90 cm−75 cm=15 cm
Conclusion
The displacement of the image when the object is moved 5 cm away from the lens is 15 cm towards the lens.
---
- Initial object distance (U) = -45 cm (negative because it is on the same side as the object)
- Initial image distance (V) = +90 cm (positive because it is on the opposite side of the lens)
- Object is moved 5 cm away, so the new object distance (U') = -45 cm - 5 cm = -50 cm
The lens formula is given by:
1F=1V−1U
Substituting the initial values:
1F=190−1−45
This simplifies to:
1F=190+145
Finding a common denominator (which is 90):
1F=190+290=390
Thus,
F=903=30 cm
Using the lens formula again with the new object distance:
1F=1V′−1U′
Substituting the values:
130=1V′−1−50
This simplifies to:
130=1V′+150
Rearranging gives:
1V′=130−150
Finding a common denominator (which is 150):
1V′=5150−3150=2150
Thus,
V′=1502=75 cm
The displacement of the image is the difference between the initial image distance and the new image distance:
Displacement=V−V′=90 cm−75 cm=15 cm
The displacement of the image when the object is moved 5 cm away from the lens is 15 cm towards the lens.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:NCERT FINGERTPS ENGLISHChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:Reflection Of Light By Spherical Mirrors
Explore 5 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:NCERT FINGERTPS ENGLISHChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:Refraction
Explore 10 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:NCERT FINGERTPS ENGLISHChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:ASSERTION AND REASON
Explore 15 Videos
- PRACTICE PAPPERBook:NCERT FINGERTPS ENGLISHChapter:PRACTICE PAPPERExercise:Practice Paper 3
Explore 50 Videos
- SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS Book:NCERT FINGERTPS ENGLISHChapter:SEMICONDUCTOR ELECTRONICS : MATERIALS , DEVICES AND SIMPLE CIRCUITS Exercise:Assertion And Reason
Explore 15 Videos
Similar Questions
The image of a candle flame formed by a lens is obtained on a screen placed on the other side of the lens. If the image is three times the size of the flame and the distance between lens and image is 80cm, at what distance should the candle be placed from the lens ? What is the nature of the image at a distance of 80cm from the lens ?
View SolutionA convex lens forms an image of an object placed 20cm away from it at a distance of 20cm on the other side of the lens. If the object is moves 5cm toward the lens, the image will be
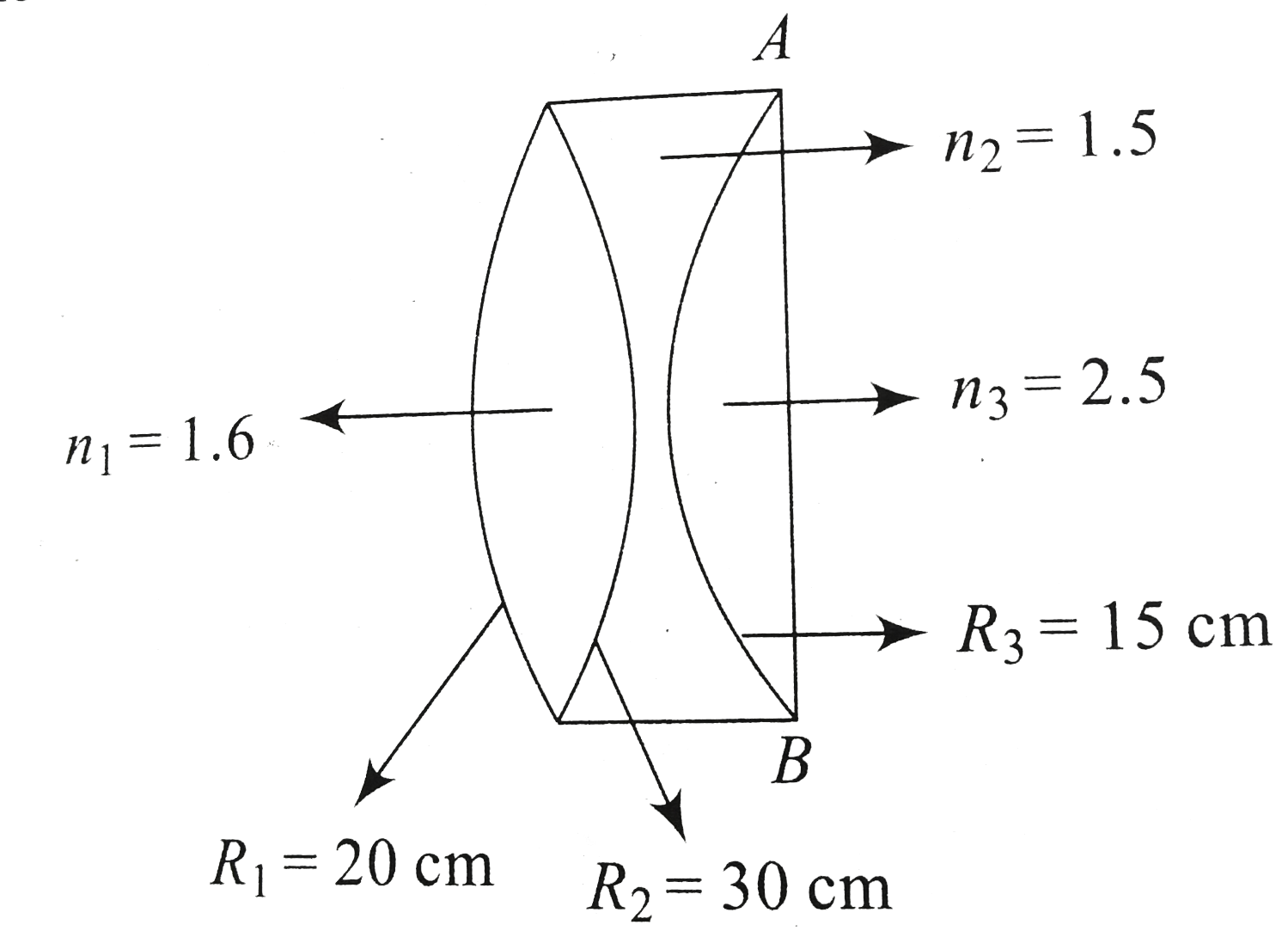
View SolutionA lens is made of three thin different mediums. Radius of curvature and refractive index of each medium is shown iin Figure., Surface AB is straight. An object is placed at some distance from the lens by which a real image is formed on the screen placed at a distance of 10cm from the lens. Find the distance of the object from the lens.
View SolutionA convex lens forms a real and inverted image of a needle at a distance of 50cm from it. Where is the needle placed in front of the convex lens if the image is equal to size of the object ? Also, find the power of the lens.
View SolutionA parallel beam of light is incident on a lens of focal length 10 cm. A parallel slab of refractive index 1.5 and thickness 3 cm is placed on the other side of the lens. Find the distance of the final image from the lens.
 .
.
View SolutionA point object is placed at a distance of 12cm from a convex lens of focal length 10cm. On the other side of the lens, a convex mirror is placed at a distance of 10cm from the lens such that the image formed by the combination coincides with the object itself. The focal length of the convex mirror is
View SolutionA point source of light is kept at a distance of 15cm from a converging lens,on its optical axis.The focal length of the lens is 10cm and its diameter is 3cm ,A screen is placed on the other side of the lens ,perpendicular to the axis of lens,at a distance 20cm from it.Then find the area of the illuminated part of the screen?
View SolutionAn object is placed 30cm away from a convex lens of focal length 10cm and a sharp image is formed on a screen. Now, a convex lens is placed In contact with the convex lens. The screen now has to be moved by 45cm to get a sharp image again. The magnitude of focal length of the convex lends in (in cm)
View SolutionWhen an object is placed 40cm from a diverging lens, its virtual image is formed 20cm from the lens. The focal length and power of lens are
View SolutionAn object is placed at 10 cm from a lens and real image is formed with magnification of 0.5. Then the lens is
View Solution
