The image of an object placed on the principal axis of a concave mirror of focal length 12 cm is formed at a point which is 10cm more distance form the mirror than the object. The magnification of the image is
8/3
2.5
2
−1.5
The correct Answer is:d.
To solve the problem step by step, we will follow the principles of optics related to concave mirrors.
1. Identify Given Values:
- Focal length of the concave mirror, f=−12cm (negative because it is a concave mirror).
- Let the object distance be u=−x (the object distance is negative in mirror convention).
- The image distance is given as v=−x+10.
2. Apply the Mirror Formula:
The mirror formula is given by:
1f=1v+1u
Substituting the values we have:
1−12=1(−x+10)+1(−x)
3. Simplify the Equation:
Rearranging the equation gives:
1−12=−x+10+x−x(−x+10)
This simplifies to:
1−12=10−x(−x+10)
4. Cross-Multiply to Eliminate Fractions:
Cross-multiplying gives:
−x(−x+10)=−12×10
Simplifying this results in:
x2−10x=120
Rearranging gives:
x2−10x−120=0
5. Solve the Quadratic Equation:
We can factor or use the quadratic formula. The quadratic can be factored as:
(x−20)(x+6)=0
Thus, the solutions for x are:
x=20orx=−6
Since x represents the object distance, we take x=20 cm (the positive value).
6. Calculate the Image Distance:
Using x=20:
u=−20cmandv=−20+10=−30cm
7. Calculate the Magnification:
The magnification m is given by:
m=−vu=−−30−20=3020=32=1.5
The negative sign indicates that the image is inverted.
Final Answer:
The magnification of the image is −1.5.
- Focal length of the concave mirror, f=−12cm (negative because it is a concave mirror).
- Let the object distance be u=−x (the object distance is negative in mirror convention).
- The image distance is given as v=−x+10.
The mirror formula is given by:
1f=1v+1u
Substituting the values we have:
1−12=1(−x+10)+1(−x)
Rearranging the equation gives:
1−12=−x+10+x−x(−x+10)
This simplifies to:
1−12=10−x(−x+10)
Cross-multiplying gives:
−x(−x+10)=−12×10
Simplifying this results in:
x2−10x=120
Rearranging gives:
x2−10x−120=0
We can factor or use the quadratic formula. The quadratic can be factored as:
(x−20)(x+6)=0
Thus, the solutions for x are:
x=20orx=−6
Since x represents the object distance, we take x=20 cm (the positive value).
Using x=20:
u=−20cmandv=−20+10=−30cm
The magnification m is given by:
m=−vu=−−30−20=3020=32=1.5
The negative sign indicates that the image is inverted.
The magnification of the image is −1.5.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
Knowledge Check
An object is kept on the principal axis of a concave mirror of focal length 10cm, at a distance of 15 cm from its pole. The image formed by the mirror is:
AVirtual and magnifiedBVirtual and diminishedCReal and magnifiedDReal and diminished
An object is placed at 20 cm from a convex mirror of focal length 10 cm. The image formed by a mirror is
View SolutionAn object is placed on the principle axis of a concave mirror of focal length 10cm at a distance of 21cm from it. A glass slab is placed between the mirror and the object as shown in figure.
Find the distance of final image formed by the mirror.

View SolutionA 1 cm object is placed perpendicular to the principal axis of a convex mirror of focal length 7.5 cm. Find its distance from the mirror if the image formed is 0.6 cm in size.
View SolutionAn object is present on the principal axis of a concave mirror at a distance 30cm from it. Focal length of mirror is 20cm.
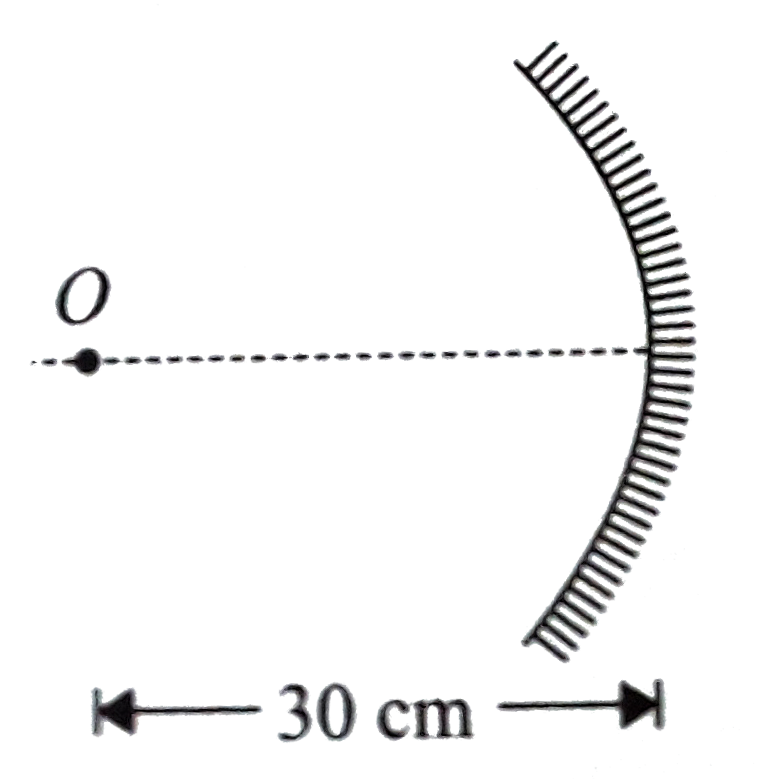
Q. Image formed by mirror is
View SolutionA point object is moving on the principal axis of a concave mirror of focal length 24cm towards the mirror. When it is at a distance of 60cm from the mirror, its velocity is . 9sec/cm What is the velocity of the image at that instant
View SolutionThe image image of an object placed at a distance of 30 cm on the principal axis of a concave mirror from its pole is formed on the object itself. Find (a) the focal length and (b) linear magnification of mirror:
View SolutionAn object is at 20 cm from a concave mirror of focal length 10 cm, the nature of image is
View Solution
