The initial state of a certain gas is . It undergoes explansion till its volume becomes . Consider the following two cases
(a) the expansion takes place at constant temperature.
(b) the expansion takes place at constant pressure.
Plot the diagram for each case. In which of the two cases, is the work done by the gas more ?
To solve the problem, we need to analyze the two cases of gas expansion, one at constant temperature (isothermal process) and the other at constant pressure (isobaric process). We will also plot the p-V diagram for both cases and determine in which case the work done by the gas is greater.
1. Identify Initial and Final States:
- Initial state: (pi,Vi,Ti)
- Final state: (pf,Vf) where Vf>Vi
2. Case A: Isothermal Expansion:
- In an isothermal process, the temperature remains constant.
- According to the ideal gas law, pV=nRT, where n is the number of moles and R is the gas constant.
- As the volume increases from Vi to Vf, the pressure decreases.
- The relationship can be expressed as:
p=nRTiV
- The p-V curve will be a hyperbola.
3. Case B: Isobaric Expansion:
- In an isobaric process, the pressure remains constant.
- The pressure during expansion remains at pi until the volume reaches Vf.
- The p-V curve will be a horizontal line at pi from Vi to Vf.
4. Plotting the p-V Diagram:
- For Case A (Isothermal): Draw a hyperbolic curve starting from (Vi,pi) to (Vf,pf).
- For Case B (Isobaric): Draw a horizontal line from (Vi,pi) to (Vf,pi).
5. Calculating Work Done:
- Work done by the gas in an expansion is given by the area under the p-V curve.
- For Case A (Isothermal):
WA=∫VfVipdV=nRTiln(VfVi)
- For Case B (Isobaric):
WB=pi(Vf−Vi)
6. Comparison of Work Done:
- To determine which work done is greater, we can analyze the expressions:
- WA=nRTiln(VfVi)
- WB=pi(Vf−Vi)
- The comparison will depend on the specific values of n,R,Ti,pi,Vi, and Vf. However, generally, for a significant volume change, the work done in the isobaric process tends to be greater because it involves a constant pressure over a larger volume change.
Conclusion:
- The work done by the gas in the isobaric process (Case B) is generally greater than that in the isothermal process (Case A).
- Initial state: (pi,Vi,Ti)
- Final state: (pf,Vf) where Vf>Vi
- In an isothermal process, the temperature remains constant.
- According to the ideal gas law, pV=nRT, where n is the number of moles and R is the gas constant.
- As the volume increases from Vi to Vf, the pressure decreases.
- The relationship can be expressed as:
p=nRTiV
- The p-V curve will be a hyperbola.
- In an isobaric process, the pressure remains constant.
- The pressure during expansion remains at pi until the volume reaches Vf.
- The p-V curve will be a horizontal line at pi from Vi to Vf.
- For Case A (Isothermal): Draw a hyperbolic curve starting from (Vi,pi) to (Vf,pf).
- For Case B (Isobaric): Draw a horizontal line from (Vi,pi) to (Vf,pi).
- Work done by the gas in an expansion is given by the area under the p-V curve.
- For Case A (Isothermal):
WA=∫VfVipdV=nRTiln(VfVi)
- For Case B (Isobaric):
WB=pi(Vf−Vi)
- To determine which work done is greater, we can analyze the expressions:
- WA=nRTiln(VfVi)
- WB=pi(Vf−Vi)
- The comparison will depend on the specific values of n,R,Ti,pi,Vi, and Vf. However, generally, for a significant volume change, the work done in the isobaric process tends to be greater because it involves a constant pressure over a larger volume change.
- The work done by the gas in the isobaric process (Case B) is generally greater than that in the isothermal process (Case A).
Topper's Solved these Questions
- THERMODYNAMICS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - THERMODYNAMICS EXERCISE - LONG ANSWER TYPE QUESTIONS
5 Videos
- THERMODYNAMICS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - THERMODYNAMICS EXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Very short Answer type Questions
15 Videos
- UNITS AND MEASUREMENTS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - UNITS AND MEASUREMENTS EXERCISE - Long Answer Type Questions
9 Videos
Similar Questions
The work done by a weightless piston in causing an expansing ΔV (at constant temperature), when the opposing pressure P is variable, is given by :
View SolutionA quantity of gas occupies an initial volume V0 at pressure p0 and temperature T0. It expands to a volume V (a) constant temperature and (b) constant pressure. In which case does the gas do more work ?
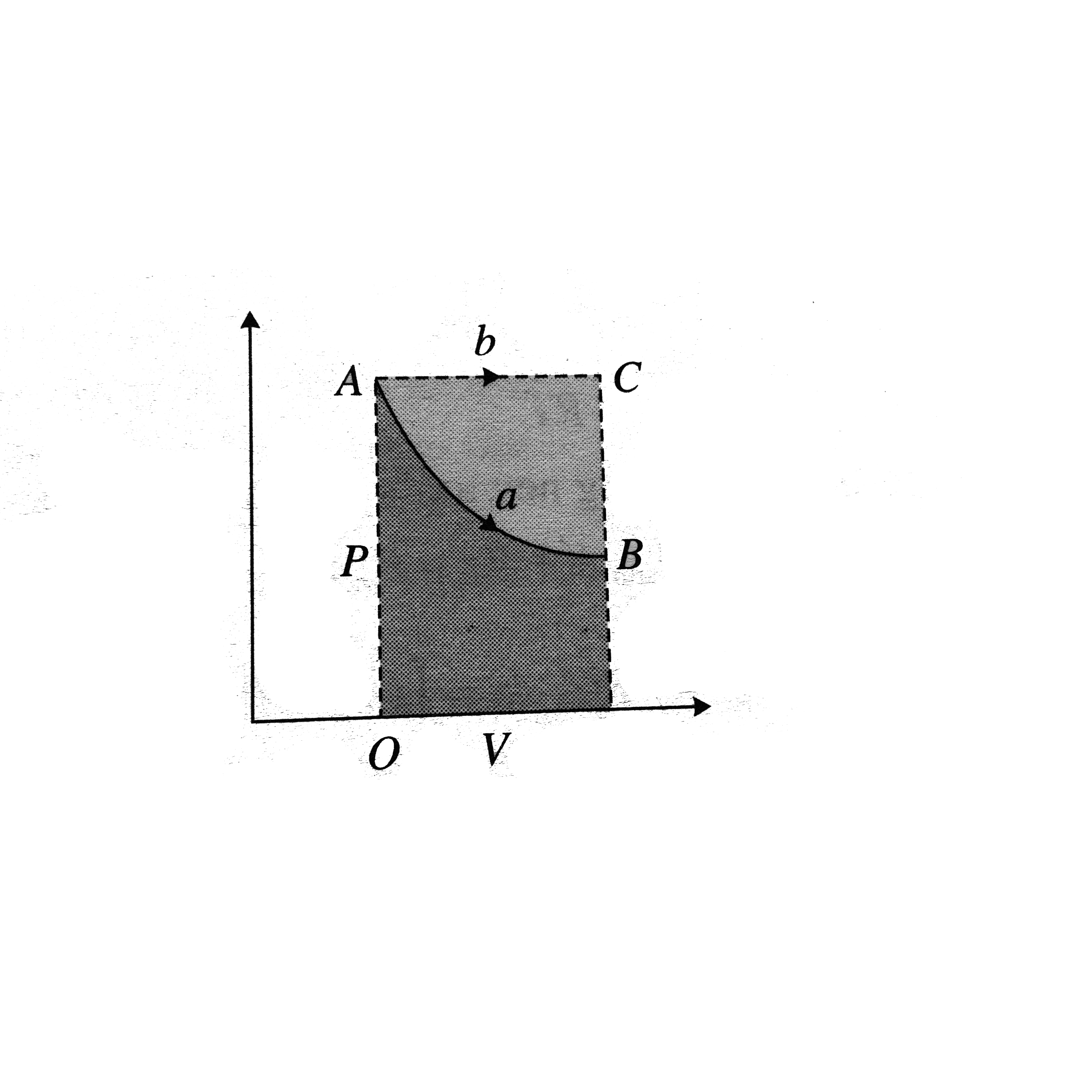
View Solution
Knowledge Check
The initial state of certain gas (PiViTi).It undergoes expansion till its volume becomes Vf at constant temperature T. The correct plot of P-V diagram for it is
A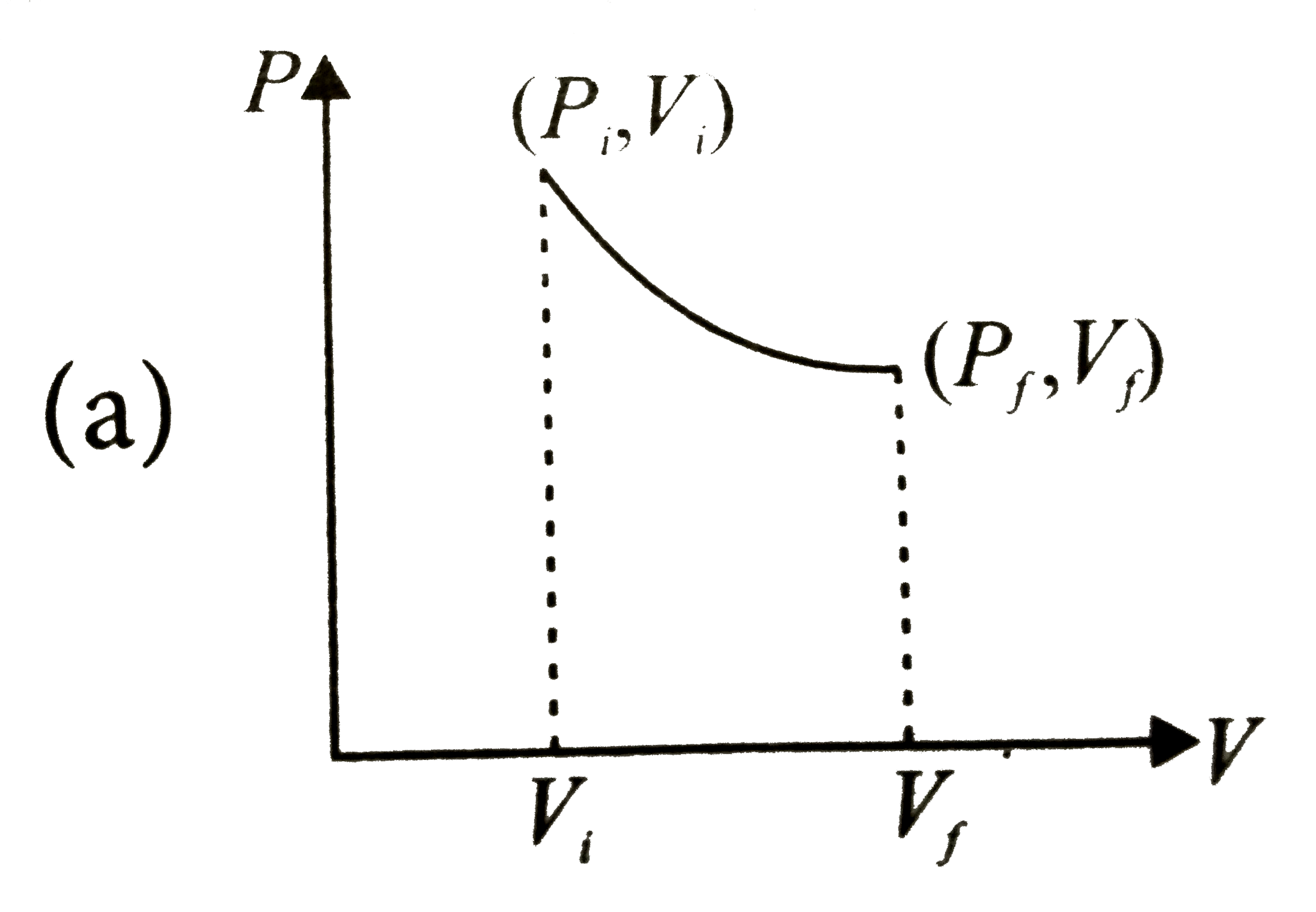 B
B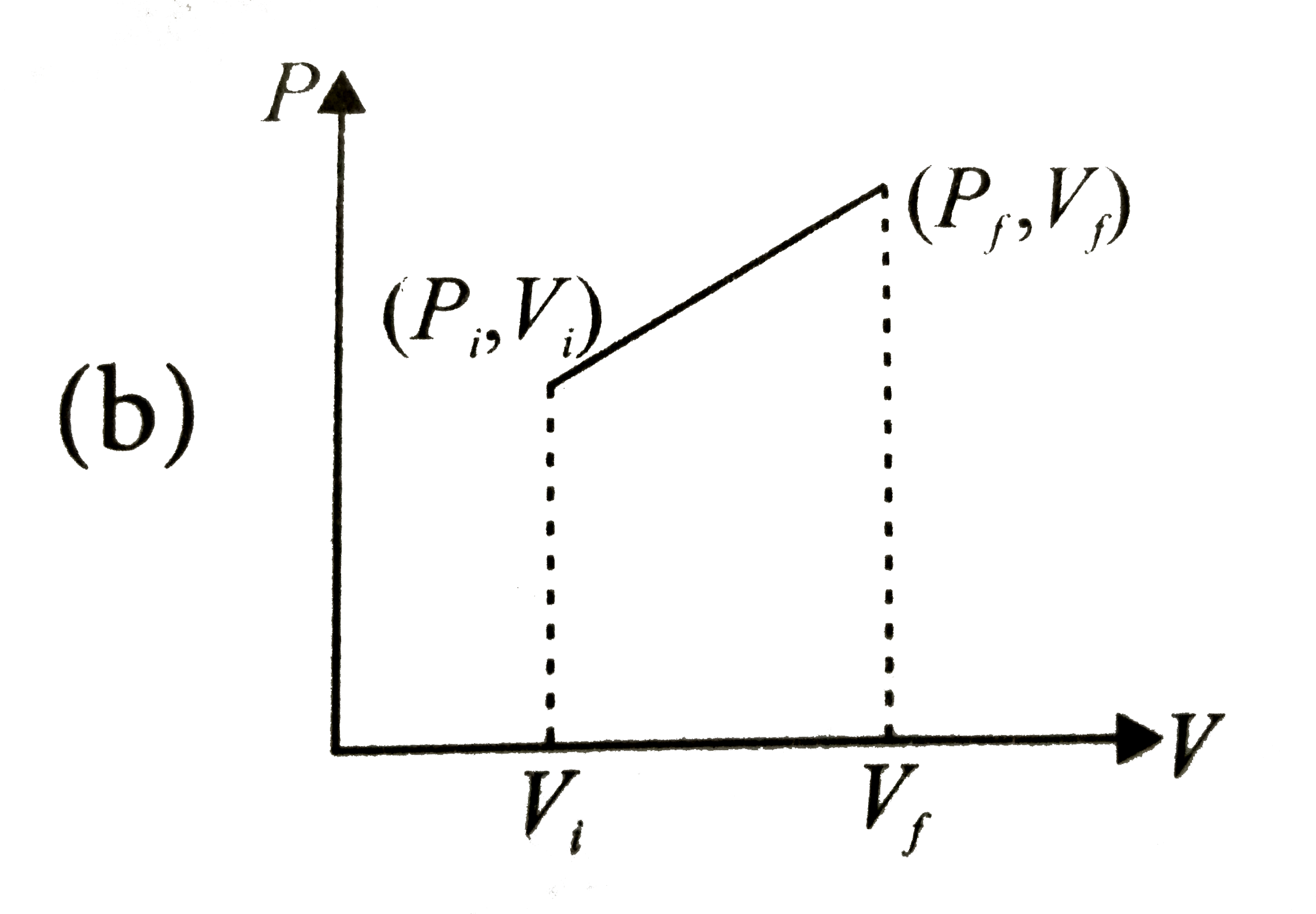 C
C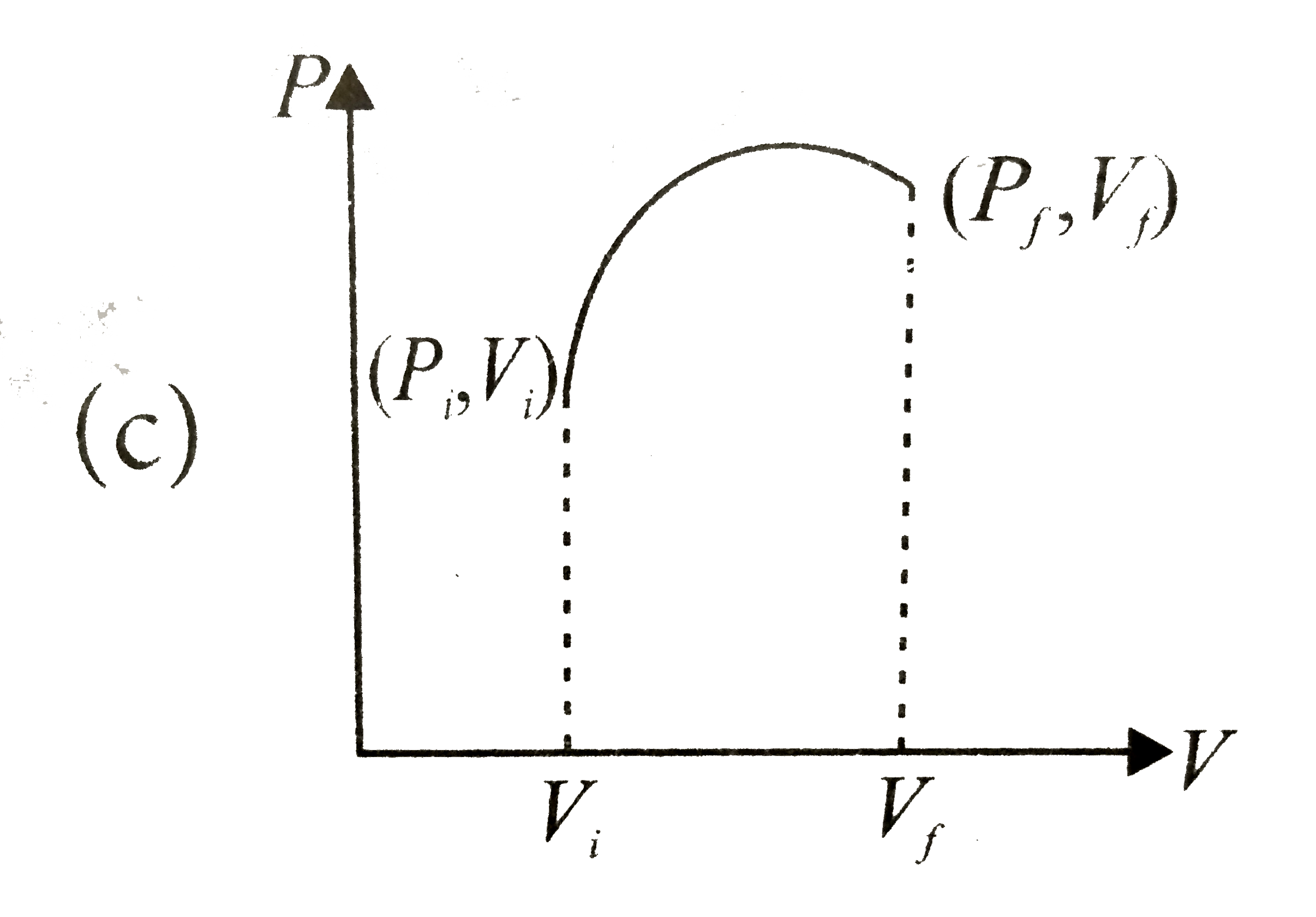 D
D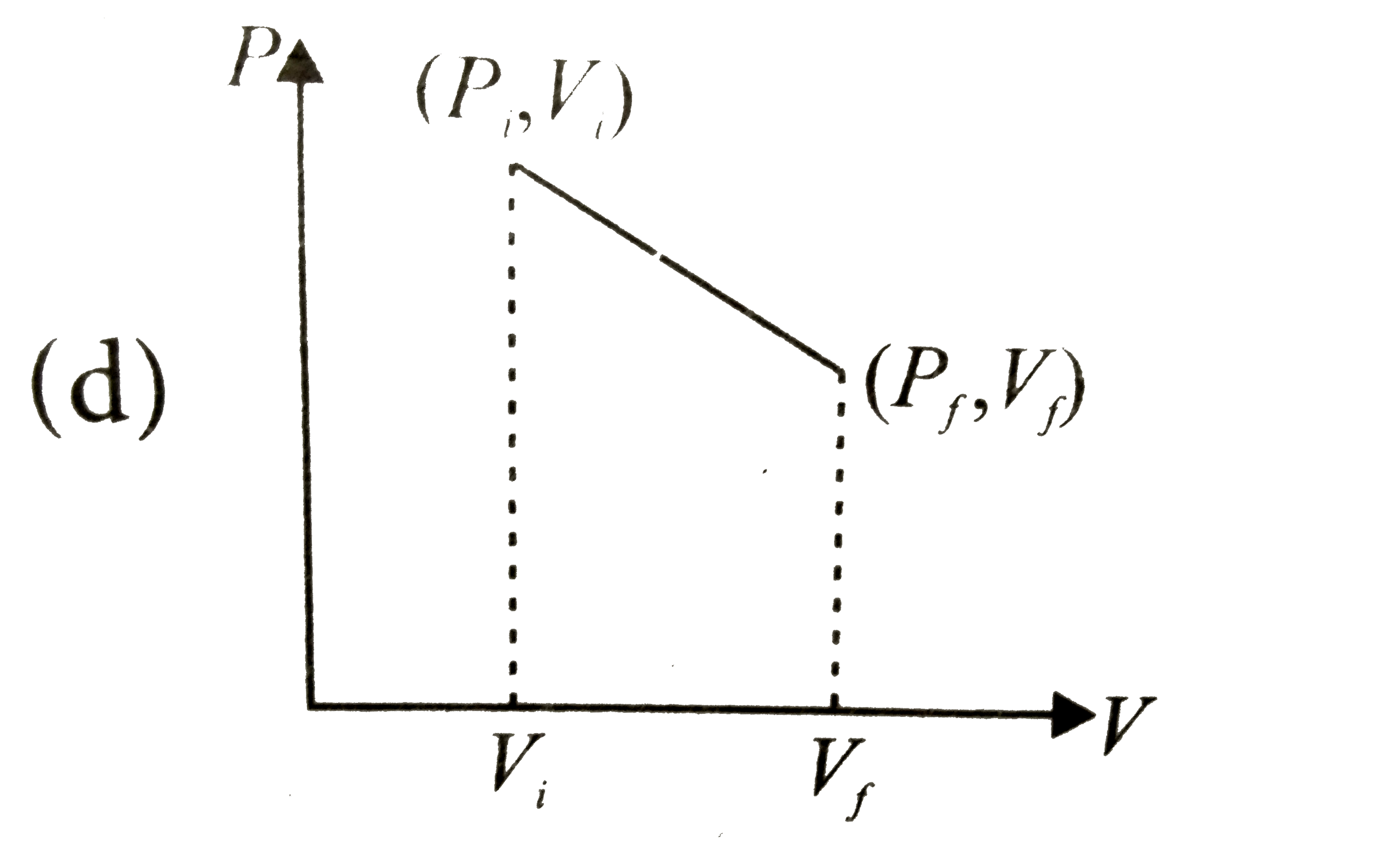
One mole of an ideal gas goes from an initial state A to final state B via two processs : It first undergoes isothermal expansion from volume V to 3V and then its volume is reduced from 3V to V at constant pressure. The correct P−V diagram representing the two process in (figure)
A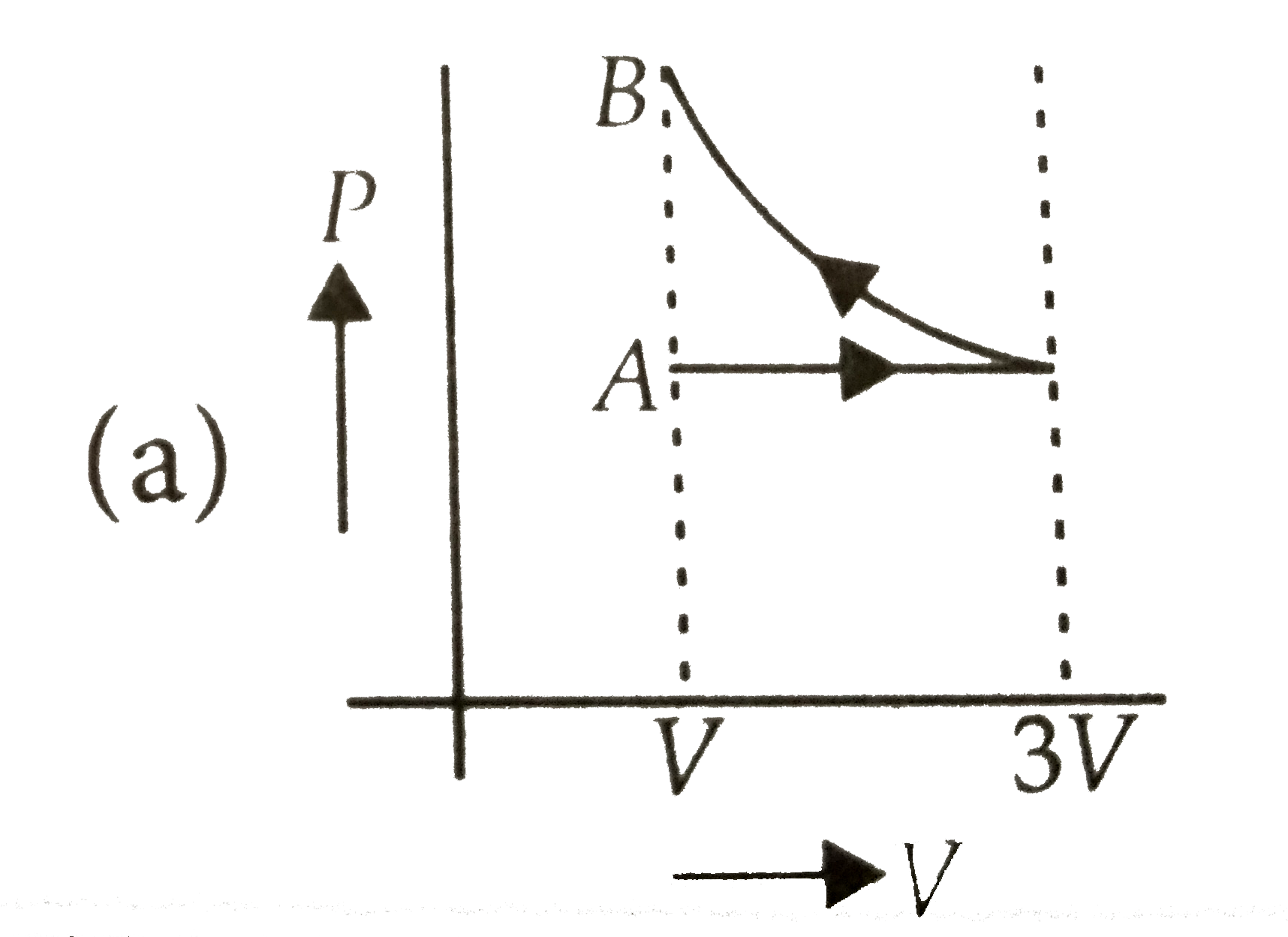 B
B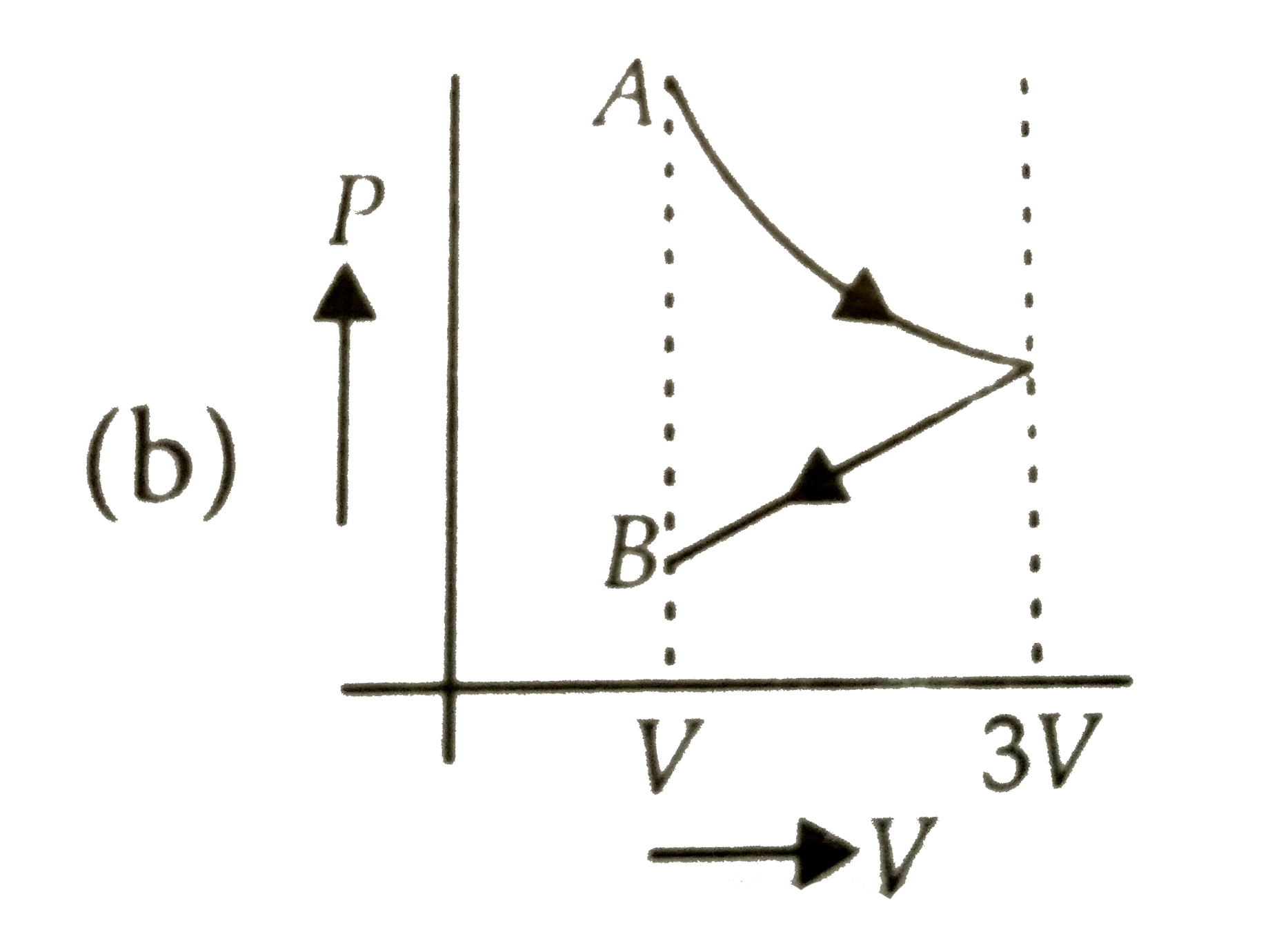 C
C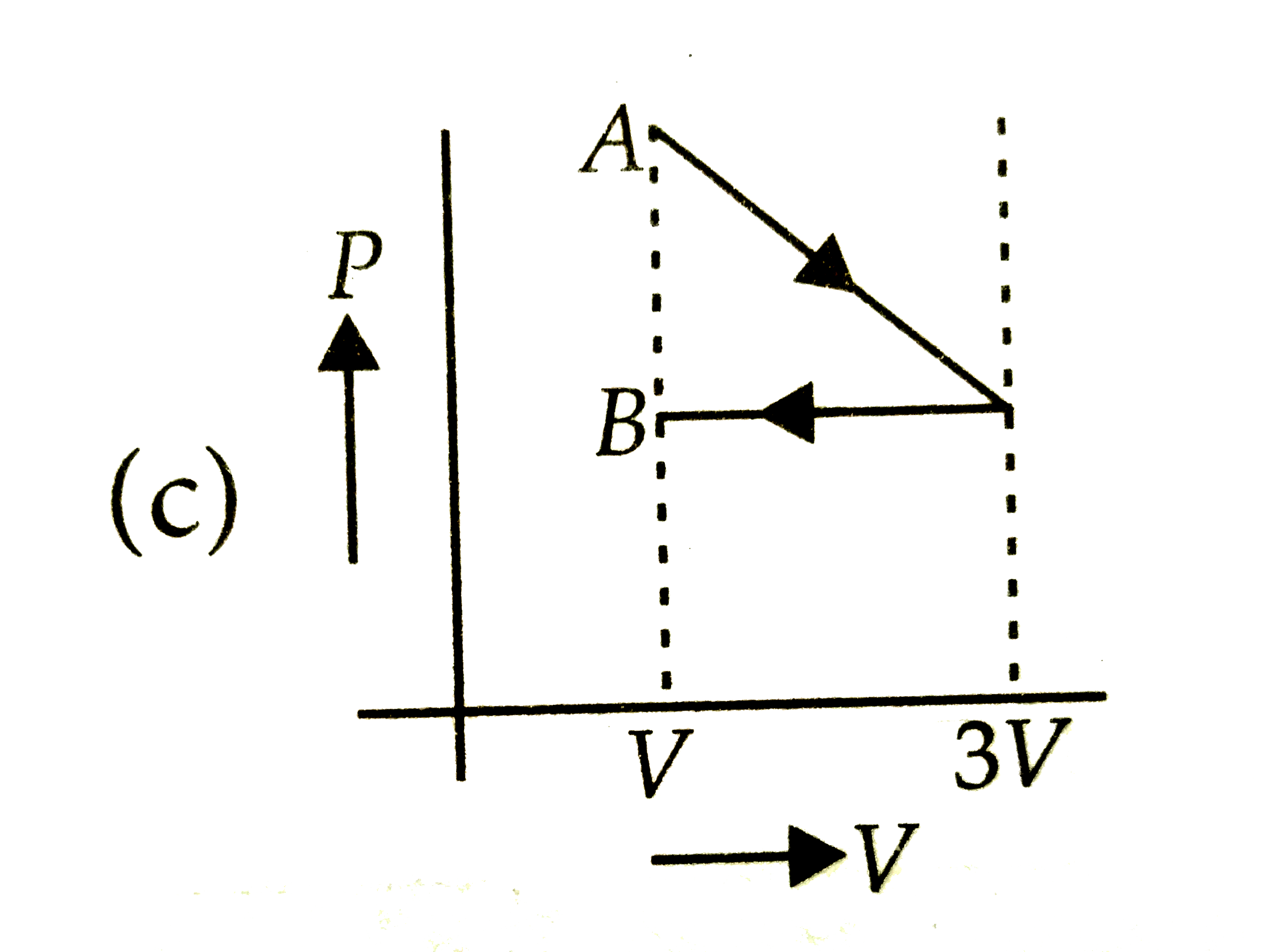 D
D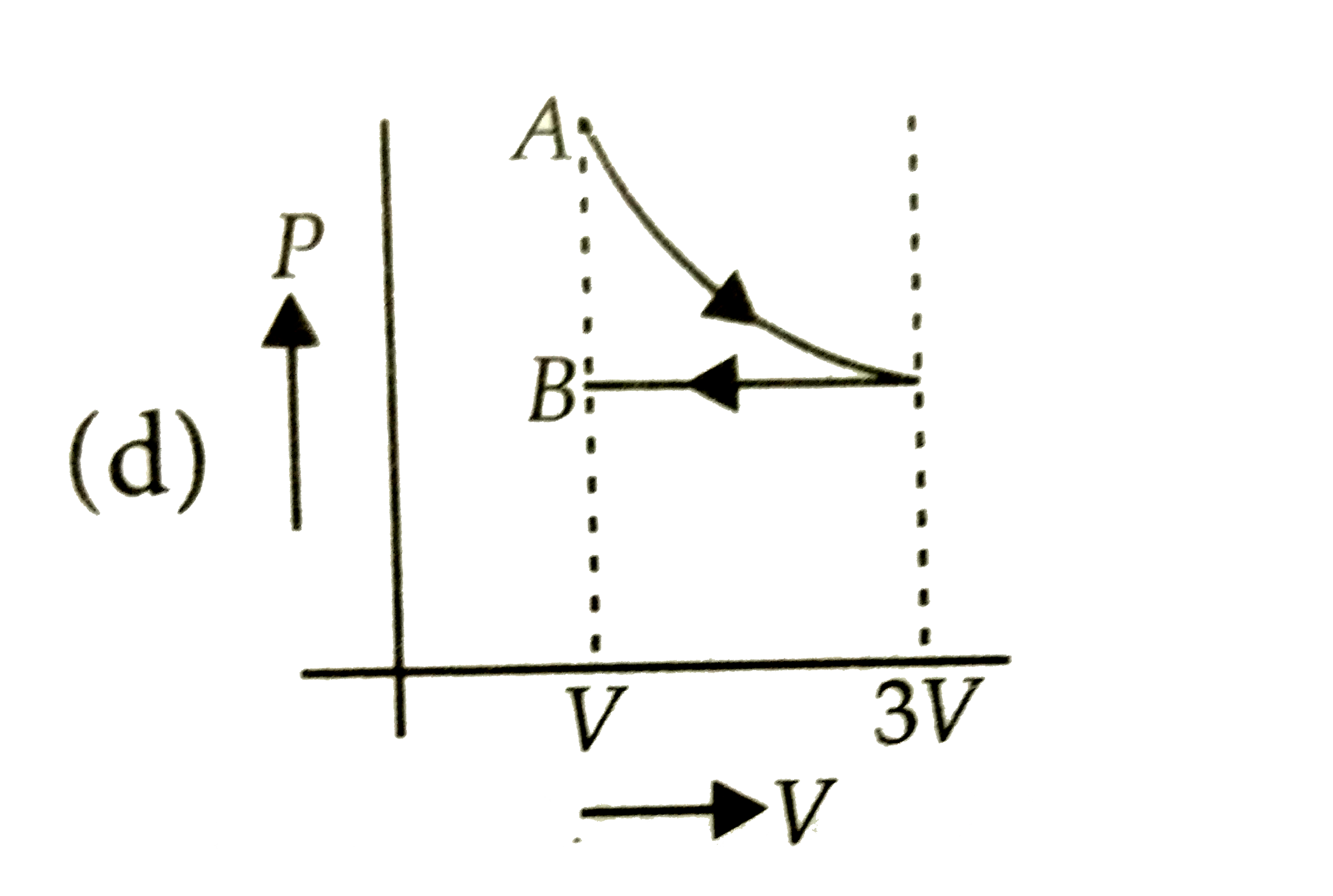
Two moles of a gas at temperature T and volume V are heated to twice its volume at constant pressure. If CpCv=γ then increase in internal energy of the gas is-
View SolutionAt constant temperature, the effect of change of pressure on volume of a gas was as given below

Plot the following graphs :
P vs V
View SolutionAt constant temperature, the effect of change of pressure on volume of a gas was as given below

Plot the following graphs :
P vs 1/V
View SolutionThree moles of an ideal gas at a pressure PA and temperature TA is isothermally expanded to twice its initial volume. It is then compressed at constant pressure to its original , volume. Finally the gas is compressed at constant volume to its original pressure PA
(a) Sketch the P-V and P-T diagrams for the complete process.
(b) Calculate the net work done by the gas, and net heat supplied to the gas during the complete process.
View SolutionOne mole of a gas is carried through the cycle shown in Fig. The gas expands at constant temperature T from volume V to 2V. It is them compressed to the initial volume at constant pressure and is finally brought back to its original state by heating at constant volume. Calculate the work done by the gas in complete cycle.

View SolutionAn ideal gas has its volume doubled under (i) isothermal conditions and (ii) isobaric (constant pressure) conditions:
(i) Write down expressions for the work done by the gas in the two cases and calculate their ratio.
(ii) In which case does the internal energy of the gas increase?
View Solution
