The internal energy of a gas is given by U=2pV. It expands from V0 to 2V0 against a constant pressure p0. The heat absorbed by the gas in the process is
(a) 2p0V0
(b) 4p0V0
(c) 3p0V0
(d) p0V0
The correct Answer is:C
To solve the problem, we will use the first law of thermodynamics, which states:
Q=ΔU+W
where Q is the heat absorbed by the gas, ΔU is the change in internal energy, and W is the work done by the gas.
Step 1: Calculate the Work Done (W)
The gas expands against a constant pressure p0 from an initial volume V0 to a final volume 2V0. The work done by the gas during this expansion can be calculated using the formula:
W=p0×(Vfinal−Vinitial)
Substituting the values:
W=p0×(2V0−V0)=p0×V0
Step 2: Calculate the Change in Internal Energy (ΔU)
The internal energy U of the gas is given by:
U=2pV
We need to find the change in internal energy as the volume changes from V0 to 2V0:
ΔU=Ufinal−Uinitial
Calculating Ufinal and Uinitial:
Ufinal=2p0×(2V0)=4p0V0
Uinitial=2p0×V0=2p0V0
Now, substituting these into the change in internal energy:
ΔU=4p0V0−2p0V0=2p0V0
Step 3: Substitute into the First Law of Thermodynamics
Now we can substitute the values of ΔU and W into the first law equation:
Q=ΔU+W
Q=2p0V0+p0V0
Combining the terms:
Q=3p0V0
Final Answer
The heat absorbed by the gas in the process is:
Q=3p0V0
---
The gas expands against a constant pressure p0 from an initial volume V0 to a final volume 2V0. The work done by the gas during this expansion can be calculated using the formula:
The internal energy U of the gas is given by:
Uinitial=2p0×V0=2p0V0
Now we can substitute the values of ΔU and W into the first law equation:
Q=2p0V0+p0V0
The heat absorbed by the gas in the process is:
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 More Than One Correct
6 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Passage I
2 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 1 Subjective
24 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Integer type Questions
10 Videos
Similar Questions
The internal energy of a gas is given by U = 3 pV. It expands from V0 to 2V0 against a constant pressure p0.
The heat absorbed by the gas in the process is
View SolutionThe internal energy of a gas is given by U=5+2PV. It expands from V0 to 2V0 against a constant pressure P0. The heat absorbed by the gas in the process is :-
View SolutionThe internal energy of a gas is given by U = 3 pV. It expands from V0 to 2V0 against a constant pressure p0.
What is the value of γ(=CP/CV) for the given gas?
View SolutionThe internal energy of a gas is given by U=1.5pV. It expands from 100cm3→200cm3 against a constant pressure of 1.0×105 Pa. calculate the heat absorbed by the gas in the process.
View SolutionThe internal energy of a gas is given by U=PV2. At constant volume, state of gas changes from (P0,V0) to (3P0,V0) then
View SolutionA diatomic gas with rigid molecules does 10J of work when expanded at constant pressure. The heat energy absorbed by the gas, in this process is ___________ (in J).
View SolutionA diatomic gas with rigid molecules does 10J of work when expanded at constant pressure. The heat energy absorbed by the gas, in this process is ___________ (in J).
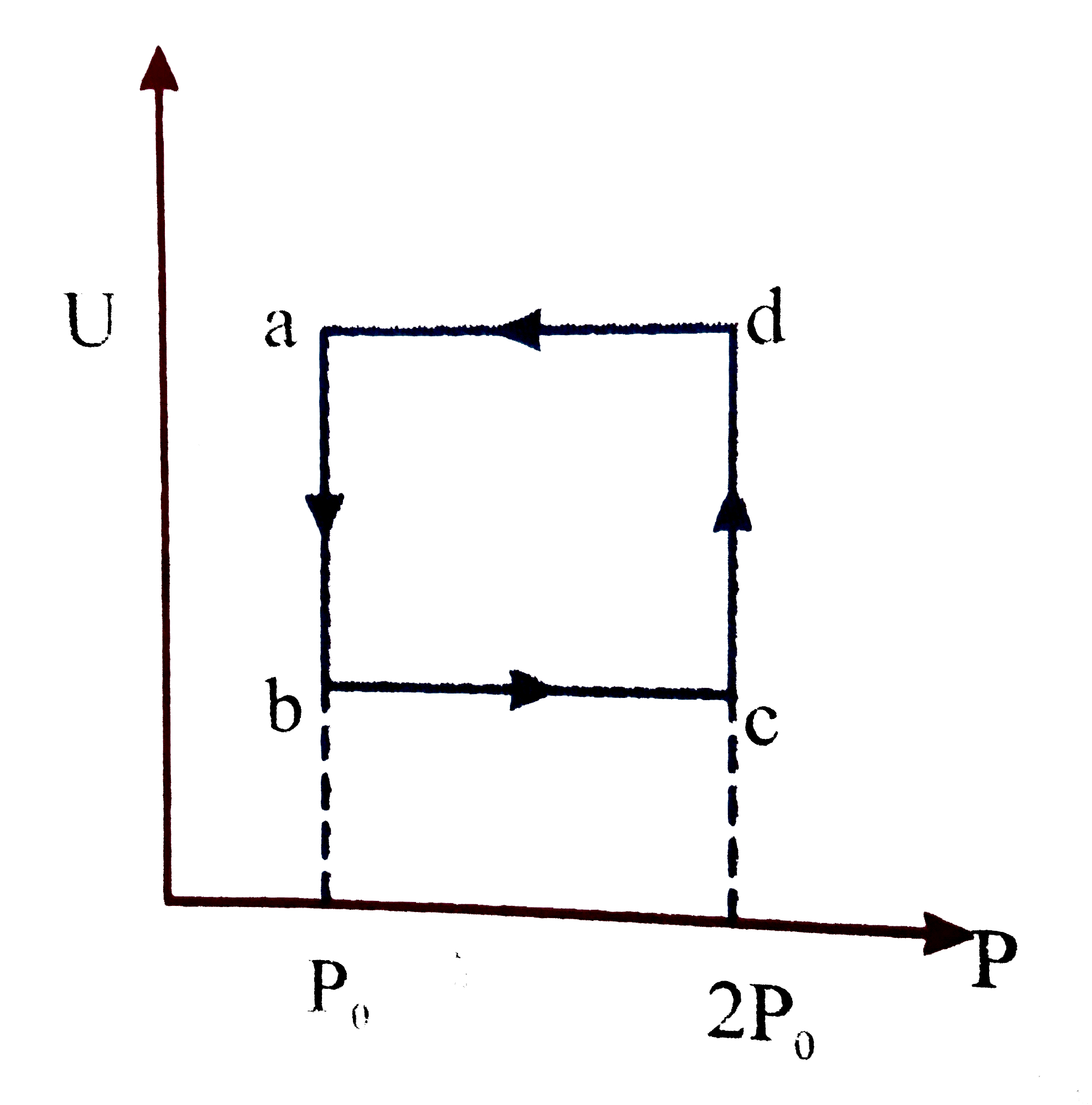
View SolutionFigure shows the variation of internal energy (U) with the pressure (P) of 2.0 mole gas in cyclic process abcda.The temperature of gas at c and d are 300and500K respectively. The heat absorbed by the gas during the process is X×10Rln2 . Find the value of x.
View SolutionPressure and volume of a gas changes from (p0V0) to (p04,2V0) in a process pV2= constant. Find work done by the gas in the given process.
View SolutionIf a gas absorbs 200J of heat and expands by 500cm3 against a constant pressure of 2×105Nm−2, then the change in internal energy is
View Solution
