A gas follows a process TVn−1=constant, where T= absolute temperature of the gas and V= volume of the gas. The bulk modulus of the gas in the process is given by
(a) (n−1)p
(b) p/(n−1)
(c) np
(d) p/n
The correct Answer is:C
To find the bulk modulus of the gas that follows the process TV(n−1)=constant, we will proceed step by step.
Step 1: Understand the definition of bulk modulus
The bulk modulus B is defined as:
B=−VdpdV
where p is the pressure and V is the volume of the gas.
Step 2: Use the given process equation
The process is given by:
TV(n−1)=constant
We can express this in terms of pressure using the ideal gas law, which states:
PV=nRT⇒T=PVnR
Step 3: Substitute T in the process equation
Substituting T into the process equation:
(PVnR)V(n−1)=constant
This simplifies to:
PVnnR=constant
Thus, we can say:
PVn=constant⋅nR
Step 4: Differentiate both sides
Now, we differentiate both sides with respect to V:
P⋅nV(n−1)dVdV+VndpdV=0
This simplifies to:
nPV(n−1)+VndpdV=0
Step 5: Rearranging the equation
Rearranging gives:
VndpdV=−nPV(n−1)
Dividing both sides by Vn:
dpdV=−nPV
Step 6: Substitute into the bulk modulus formula
Now substituting dpdV into the bulk modulus formula:
B=−V(−nPV)=nP
Conclusion
Thus, the bulk modulus of the gas in the process is:
B=nP
The bulk modulus B is defined as:
B=−VdpdV
where p is the pressure and V is the volume of the gas.
The process is given by:
TV(n−1)=constant
We can express this in terms of pressure using the ideal gas law, which states:
PV=nRT⇒T=PVnR
Substituting T into the process equation:
(PVnR)V(n−1)=constant
This simplifies to:
PVnnR=constant
Thus, we can say:
PVn=constant⋅nR
Now, we differentiate both sides with respect to V:
P⋅nV(n−1)dVdV+VndpdV=0
This simplifies to:
nPV(n−1)+VndpdV=0
Rearranging gives:
VndpdV=−nPV(n−1)
Dividing both sides by Vn:
dpdV=−nPV
Now substituting dpdV into the bulk modulus formula:
B=−V(−nPV)=nP
Thus, the bulk modulus of the gas in the process is:
B=nP
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 More Than One Correct6videos
LAWS OF THERMODYNAMICS
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Passage I2videos
LAWS OF THERMODYNAMICS
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 1 Subjective24videos
LAWS OF THERMODYNAMICS
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery39videos
LAWS OF MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Integer type Questions10videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
Similar Questions
ρ-T equation of a gas in adiabatic process is given by
View SolutionA gas undergoes a process in which its pressure P and volume V are related as VPn= constant. The bulk modulus of the gas in the process is:
View SolutionThe bulk modulus of an ideal gas at constant temperature
View SolutionA given quantity of a ideal gas is at pressure P and absolute temperature T. The isothermal bulk modulus of the gas is
View SolutionA given quantity of a ideal gas is at pressure P and absolute temperature T. The isothermal bulk modulus of the gas is
View SolutionIf ideal diatomic gas follows the process, as shown in graph, where T is temperature in kelvin and V is volume (m3), then molar heat capacity for this process will be [in terms of gas constant R]:
 View Solution
View SolutionFigure shows the adiabatic curve for n moles of an ideal gas, the bulk modulus for the gas corresponding to the point P will be
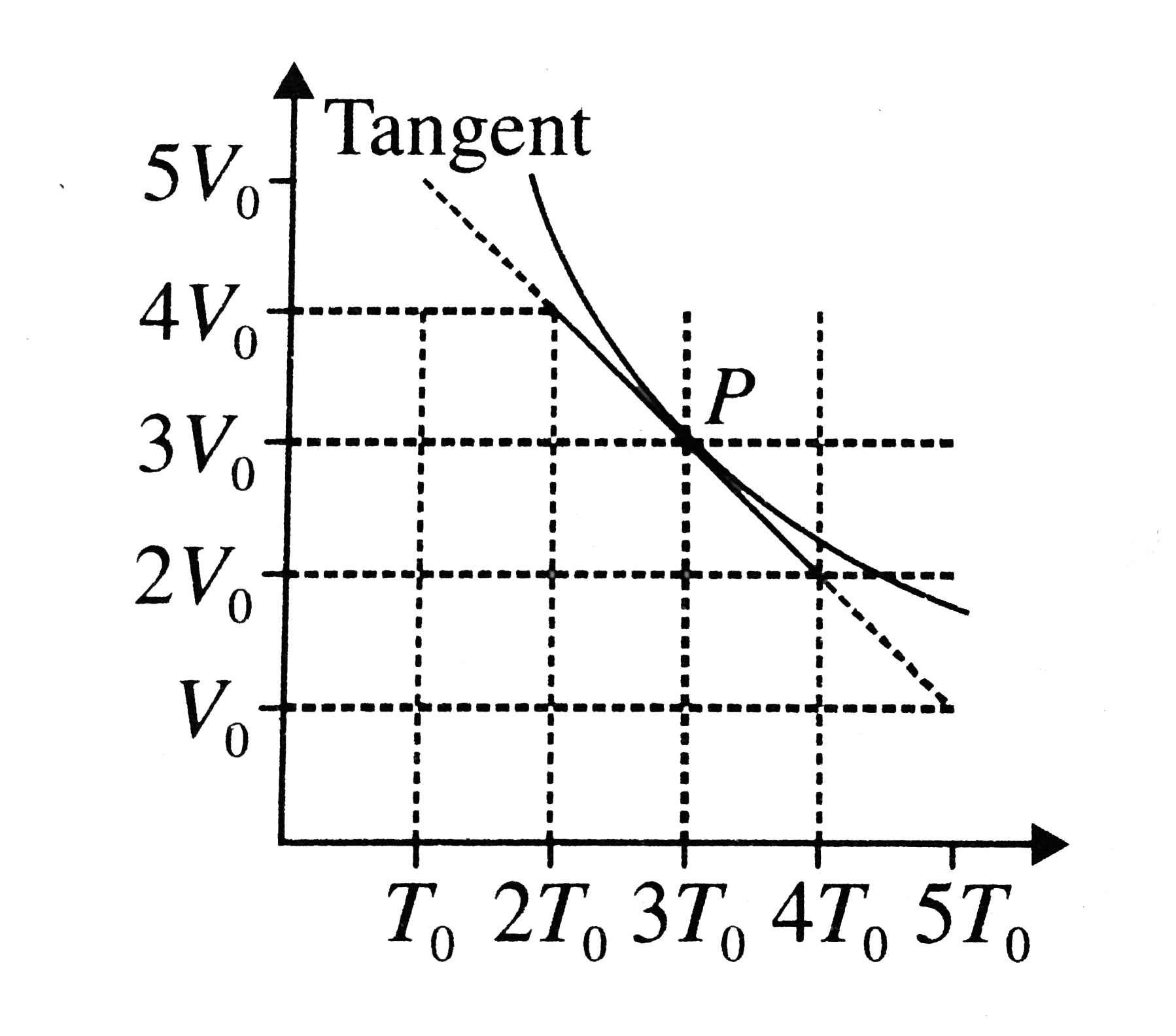 View Solution
View SolutionFigure shows the adiabatic curve for n moles of an ideal gas, the bulk modulus for the gas corresponding to the point P will be
 View Solution
View SolutionA gas undergoes a process in which its pressure P and volume V are related as VP = constant. The bulk modulus of gas for this process
View SolutionIn a process V∝T2, temperature of 2 moles of a gas is increased by 200K. Work done by the gas in this process will be
View Solution

