The kinetic energies of the photoelectrons are E1 and E2 with wavelength of incident light λ1 and λ2. The work function of the metal is :Solution in Kannada
E1λ1−E2λ2λ2−λ1
E1E2λ1−λ2
(E1−E2)λ1λ2λ1−λ2
λ1λ2E1(λ1−λ2)E2
The correct Answer is:A
E=hcλ1−wandE2=hcλ2−w
∴E1+wE2+w=λ2λ1orw=E1λ1−E2λ2λ2−λ1
Topper's Solved these Questions
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - MULTIPLE CHOICE QUESTIONS (LEVEL-III)
9 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- ELECTRONS & PHOTONSBOOK - MODERN PUBLICATIONCHAPTER - ELECTRONS & PHOTONSEXERCISE - RECENT COMPETITIVE QUESTIONS
20 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - RECENT COMPETITIVE QUESTIONS
4 Videos
- ELECTROSTATICSBOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - RECENT COMPETITIVE QUESTIONS
39 Videos
Similar Questions
Energy required for the electron excitation in Li++ from the first to the third Bohr orbit is :
View SolutionAccording to Einstein's photoelectric equation the graph of K.E. of the photoelectron emitted from the metal versus the frequency of the incident radiation gives a straight line graph whose slope
View SolutionAccording to Einstein's photoelectric equation the graph of K.E. of the photoelectron emitted from the metal versus the frequency of the incident radiation gives a straight line graph whose slope
View SolutionThe speed of electromagnetic wave in vacuum depends upon the source of radiation
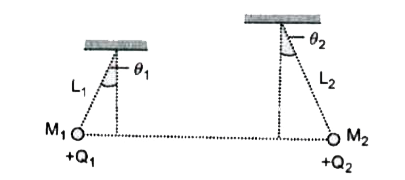
View SolutionTwo small spheres of masses M1andM2 are suspended by weightless insulating threads of lengths L1andL2 The spheres carry charges Q1andq2 respectively . The spheres are suspended such that they are in level with one another and the threads are inclined to the vertical at angles of θ1andθ2 as shown . Which one o fthe following conditions , if θ1=θ2 ?
View SolutionIf light of wavelength λ1 is allowed to fall on a metal , then kinetic energy of photoelectrons emitted is E1. If wavelength of light changes to λ2 then kinetic energy of electrons changes to E2. Then work function of the metal is
View SolutionCalculate the work function of the metal, if the kinetic energies of the photoelectrons are E_(1) and E_(2) , with wavelengths of incident light lambda_(1) and lambda_(2)
View Solutionआइंस्टीन का प्रकाश - वैधुत समीकरण लिखिए । किसी धातु पृष्ठ पर आपतित प्रकाश की तरंगदैर्ध्य λ1 से λ2 परिवर्तित की जाती हैं तब उत्सर्जित प्रकाश - इलेक्ट्रॉन की गतिज ऊर्जा दोगुनी हो जाती हैं । तरंगदैर्ध्य λ0 के सूत्र की स्थापना कीजिए ।
View SolutionThe energines E_(1) and E_(2) of two radiations are 25 eV and 50 eV respectively. The relation between their wavelengths, i.e., lamda_(1) and lamda_(2) will be
View SolutionWhen radiations of wavelength lamda_(1) and lamda_(2) are incident on certain photosensitive material, the energies of electron ejected ar E_(1) and E_(2) respectively, such that E_(1)gt E_(2), Then Planck's constant 'h' is (C = velocity of light)
View SolutionThe energy of a photon is equal to the kinetic energy of a proton. If λ1 is the de-Broglie wavelength of a proton, λ2 the wavelength associated with the proton and if the energy of the photon is E, then (λ1/λ2) is proportional to
View SolutionThe energies E_(1) and E_(2) of two radiations are 25eV and 50eV respectively. The relation between their wavelengths i.e., lamda_(1) and lamda_(2) will be-
View Solutionकिसी परमाणु के ऊर्जा स्तर A,B व C की ऊर्जायें EA,EBतथाEC है । यदि C से B में B से A में तथा C से A में इलेक्ट्रॉनों के संक्रमण से प्राप्त विकिरणों की तरंगदैर्ध्य क्रमशः λ1,λ2,वλ3 है तो सिध्द करो कि -
λ3=λ1λ2λ1+λ2
View SolutionThe energies E_(1) and E_(2) of two radiations are 25 eV and 50 eV respectively. The relation beween their wavelength i.e. lamda_(1) and lamda_(2) will be
View Solutionदो विकिरणों की ऊर्जाएं E1 तथा E2 क्रमशः 25 eV तथा 50 eV है λ1 तथा λ2 के बीच संबंध होगा:
View Solution
