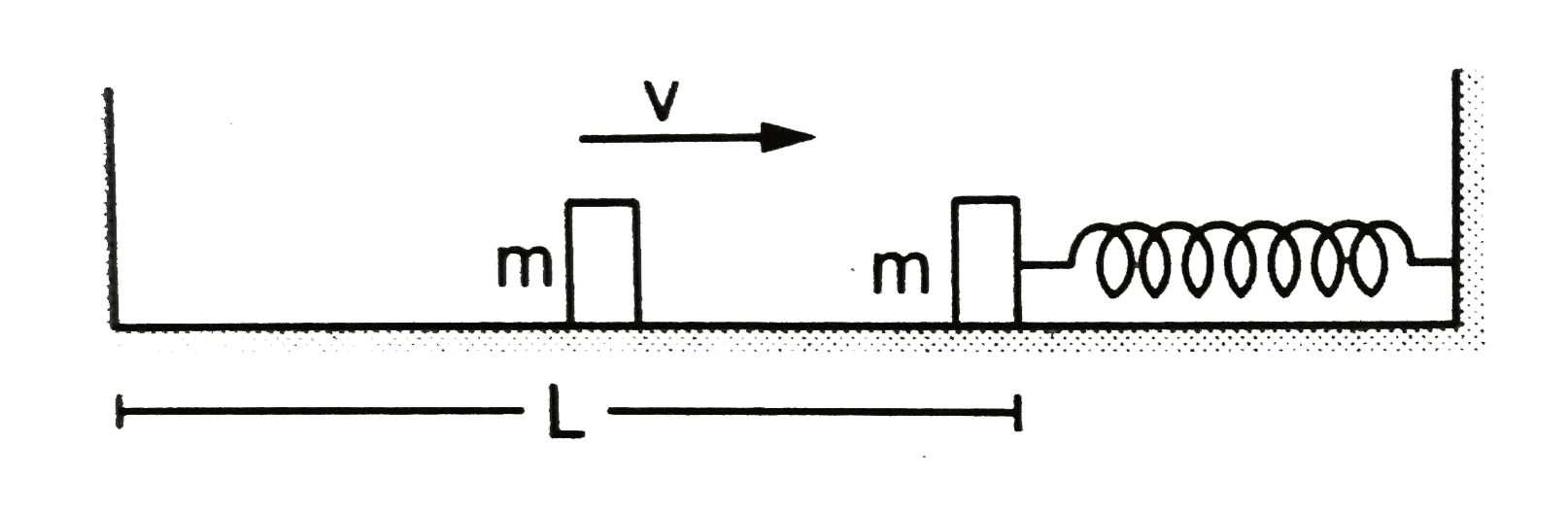
The left block in figure moves at a speed v towards the right block placed in equilibrium. All collisions to take place are elastic and the surfaces are frictionless. Show that motion of the two blocks are periodic. Find the time period of these periodic motions. Neglect the widths of the blocks.
 Solution in Tamil
Solution in Tamil
The correct Answer is:B
When the block A moves with velocity V and collides with the block B, it transfers all energy to the block B. (because it is an elastic collision). The block A will move a distance x against the spring, again the spring, again the block b will return to the origiN/Al point and completes half of the oscillation.
So, the time period of b is
2π√mk2=mk
The block B collides with the block A and comes o rest at that point. The block AS again moves a further distance L to return to its origiN/Al position. The time taken is
LV+LV=2(LV)
So the period of the periodic motion is
2LV+π√(mk)
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Objective-2
16 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Exercises
86 Videos
- SOME MECHANICAL PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - SOME MECHANICAL PROPERTIES OF MATTEREXERCISE - Exercises
32 Videos
Similar Questions
Select the correct statements
View SolutionA wheel is making revolutions about its axis with uniform angular acceleration. Starting from rest, till it reaches 100 rev/sec in 4 seconds. Find the angular acceleration.
View SolutionA load of 10 kg is suspended by a metal wire 3 m long and having a cross sectional area 4mm2. Find a. the stress b. the strain and c. the elongation. Young modulus of the metal is2.0×1011Nm−2
View SolutionFigure shows a small block of mass m which is started with a speed v on the horizontal part of the bigger block of mas m placed on a horizontal floor. The curved part of the surface shown is semicircular. All the surfaces are frictionless. Find the speed of the bigger block when the smaller block reaches the point A of the surface.

View SolutionFind the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.

View Solution(a) The right block collides with left block and sticks to it. Find amplitude of the resulting SHM.
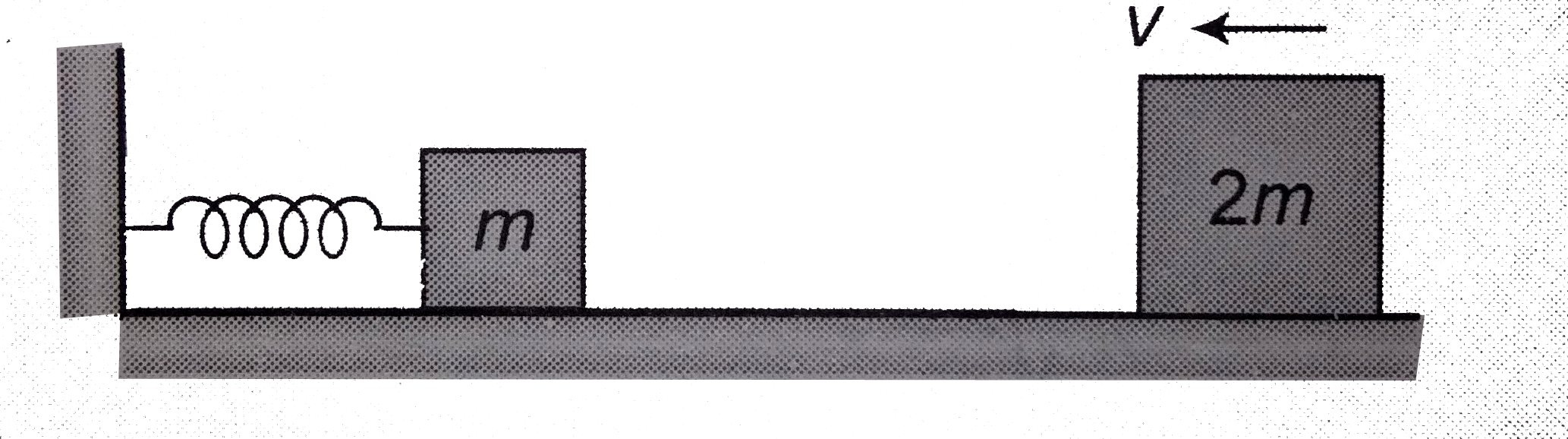
(b) The block starts from left wall and moves with constant speed v. All collisions are elastic. Find time period of periodic motion.

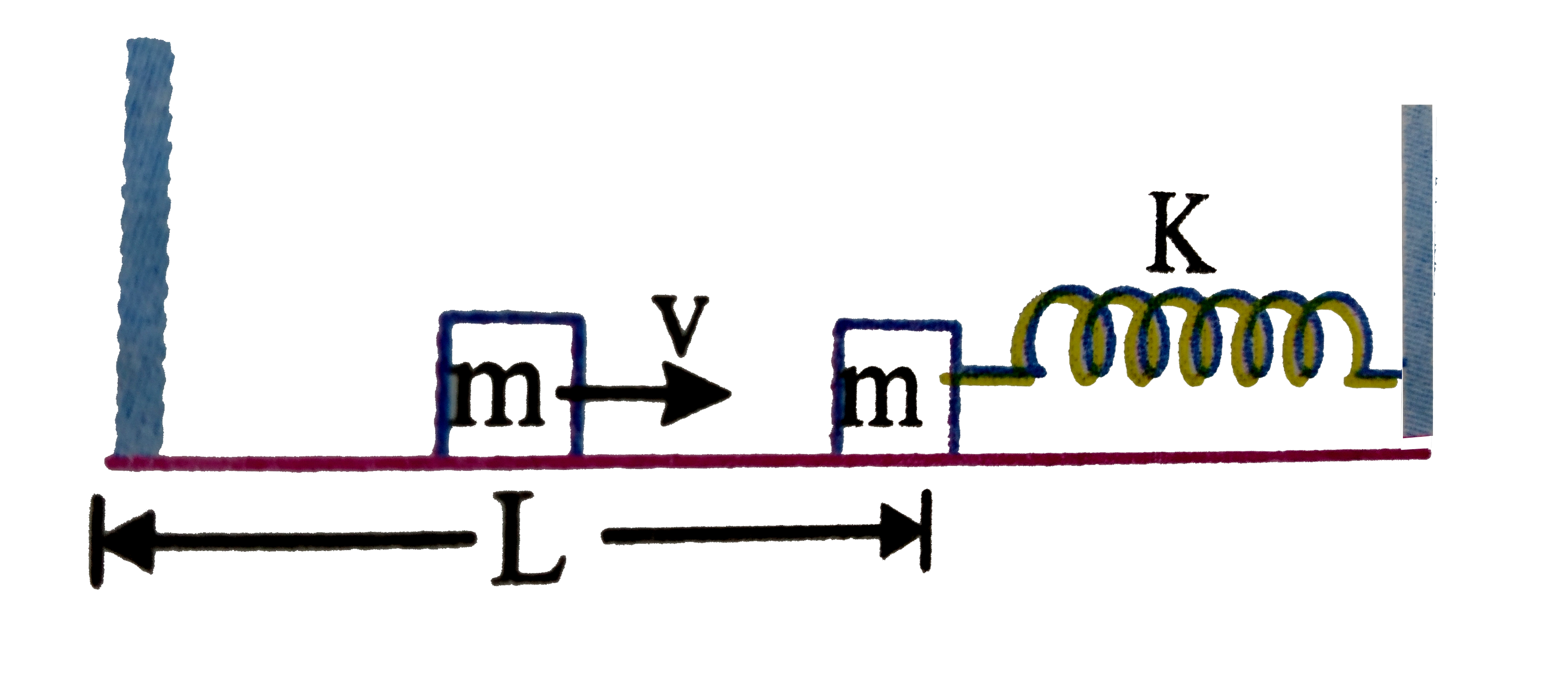
View SolutionA block of mass m moves with a speed v towards the right block which is in equilibrium with a spring attached to rigid wall. If the surface is frictionless and collisions are elastic, the frequency of collisions between the masses will be:
View SolutionA block is attached to a spring and is placed on a horizontal smooth surface as shown in which spring is unstretched. Now the spring is given an initial compression 2x0 an block is released from rest. Collision with the wall PQ are elastic.
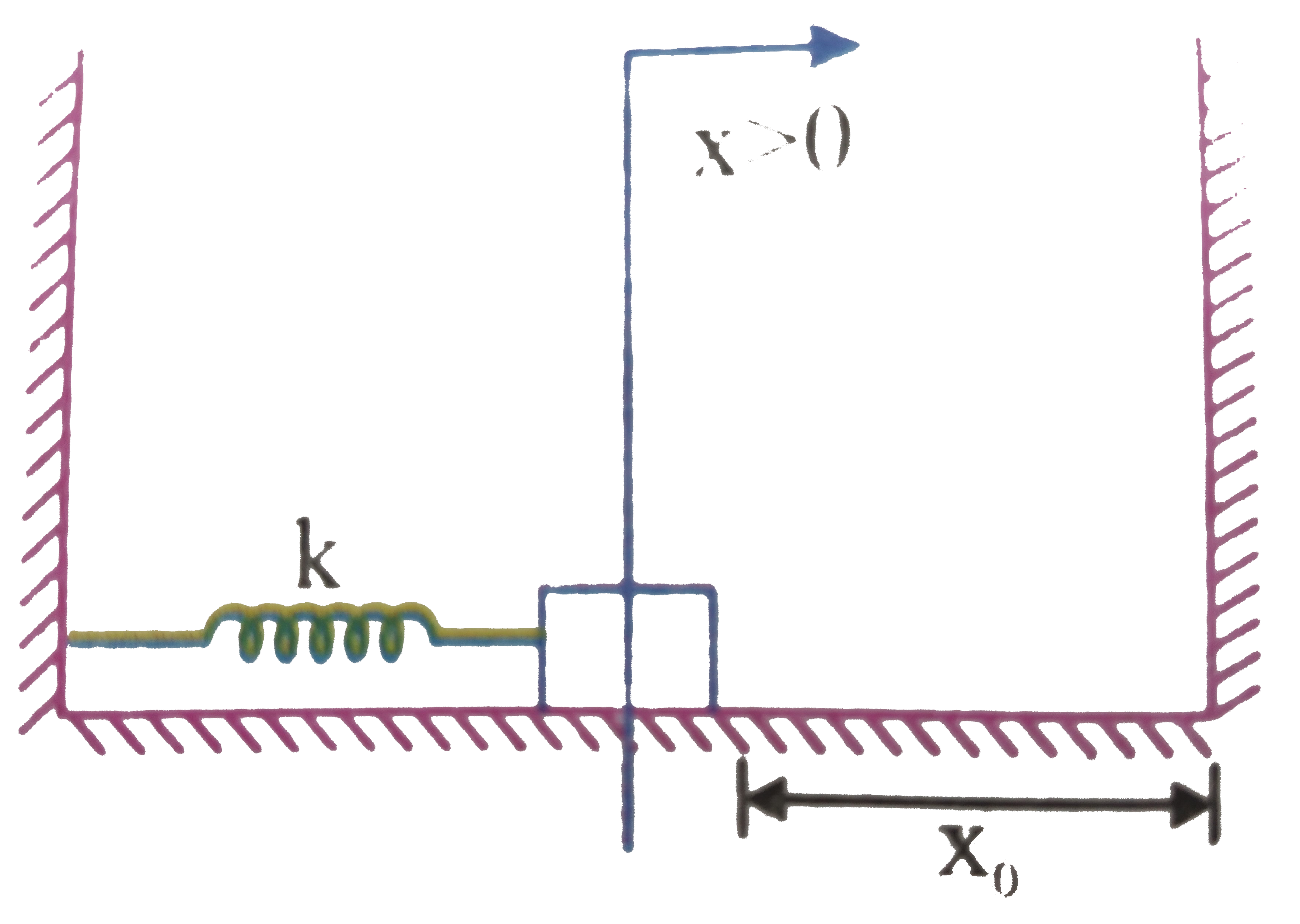
Find the time period of motion of the block:
View SolutionThe right block in figure moces at a speed V towards the left block placed in equilibrium. All the surfaces are smooth and all the collisions are elastic. Find the time period motion. Neglect the width of the blocks.
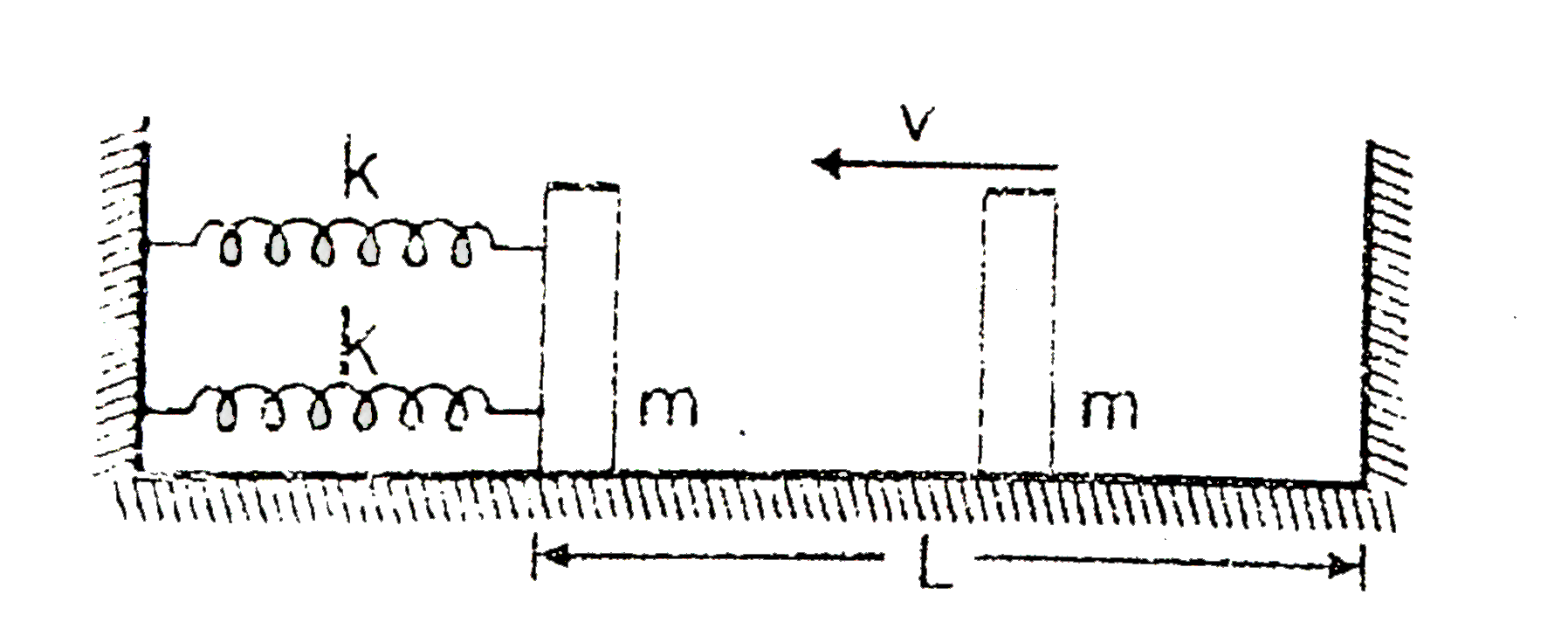
View SolutionThe right block in figure moces at a speed V towards the left block placed in equilibrium. All the surfaces are smooth and all the collisions are elastic. Find the time period motion. Neglect the width of the blocks.
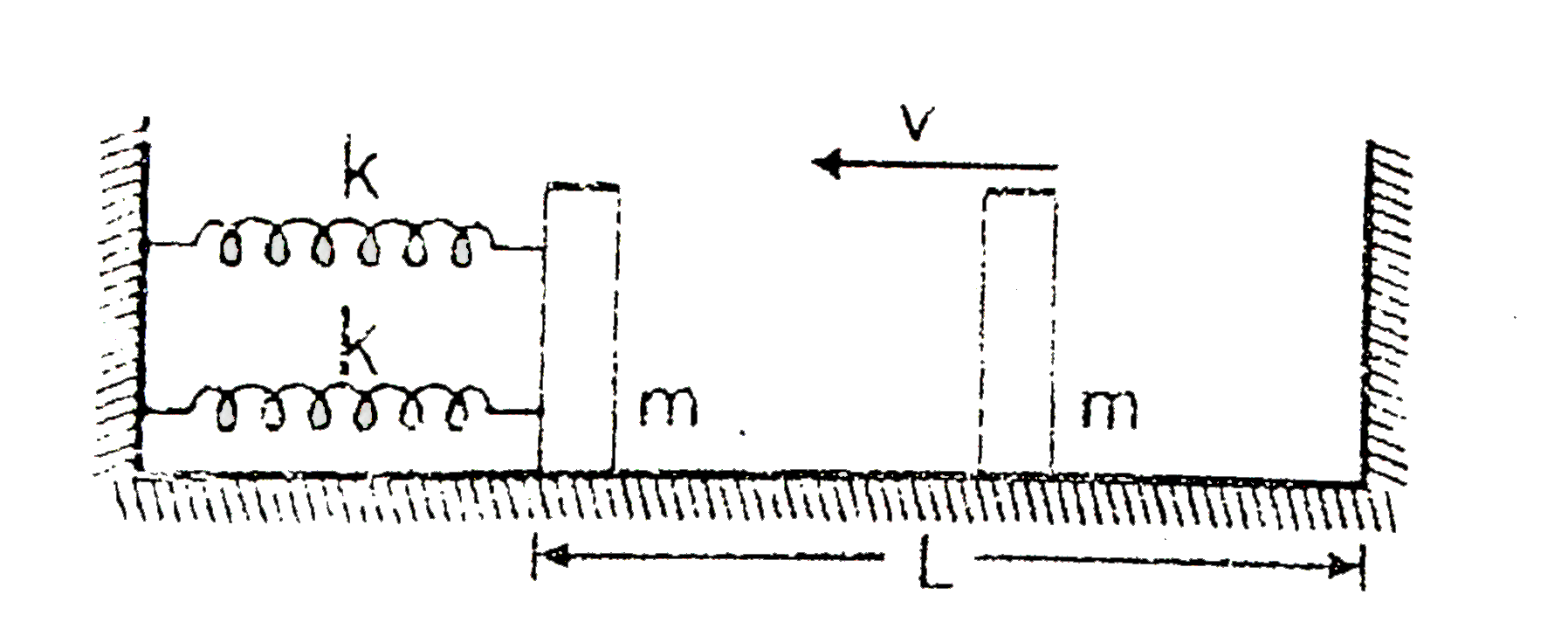
View SolutionA block having mass m and charge Q is resting on a frictionless plane at a distance d from fixed large non-conducting infinite sheet of uniform charge density −σ as shown in Figure. Assuming that collision of the block with the sheet is perfectly elastic, find the time period of oscillatory motion of the block. Is it SHM ?
View Solutionचित्र में एक M द्रव्यमान का बड़ा गुटका एक क्षैतिज सतह पर रखा हुआ दिखाया गया है। इसके क्षैतिज भाग पर द्रव्यमान का एक छोटा गुटका चाल से गति करना प्रारंभ करता है। चित्र में प्रदर्शित सतह का वक्राकार भाग अर्द्धवृत्ताकार है। सभी सतहों को घर्षणरहित मानें। जब छोटा गुटका सतह के बिंदु A पर पहुँचता है उस समय बड़े गुटके की चाल ज्ञात करें।

View Solutionचित्र 21.E10 में दिखाई गई परिस्थिति पर विचार करें। दिखाएँ कि यदि दोनों ब्लॉकों को विपरीत दिशाओं में खीचकर छोड़ दिया जाए, तो वे सरल आवर्त गति करेंगे। प्रत्येक ब्लॉक की सरस आवर्त गति का आवर्तकल निकाले
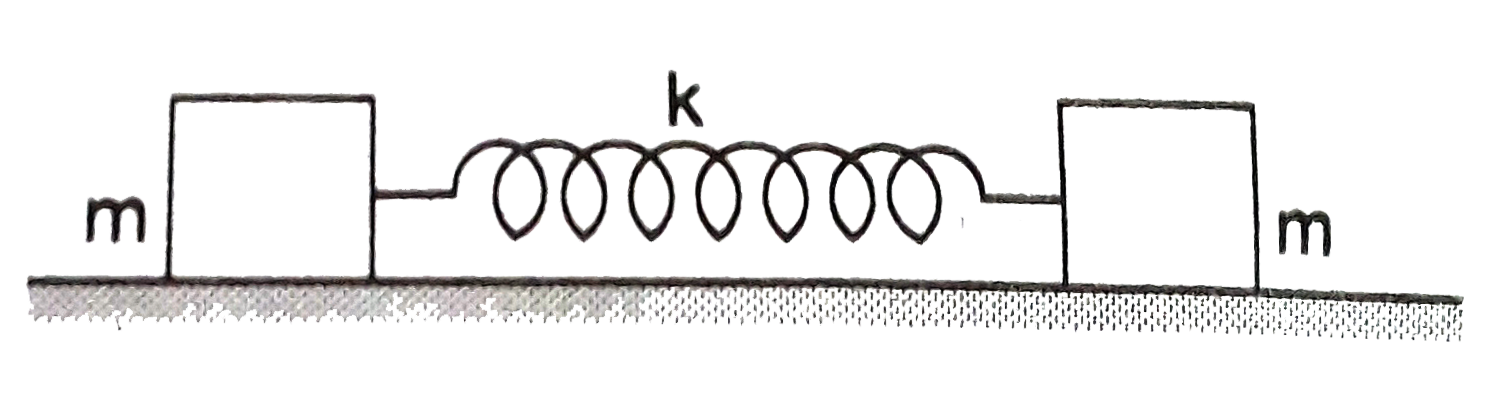
View SolutionFigure shows a small block of mass m which is started with a speed v on the horizontal part of the bigger block of mas m placed on a horizontal floor. The curved part of the surface shown is semicircular. All the surfaces are frictionless. Find the speed of the bigger block when the smaller block reaches the point A of the surface.

View SolutionFind the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.

View Solutionसभी s-ब्लॉक तत्वों को आवर्त सारणी में रखा गया है
View Solution
