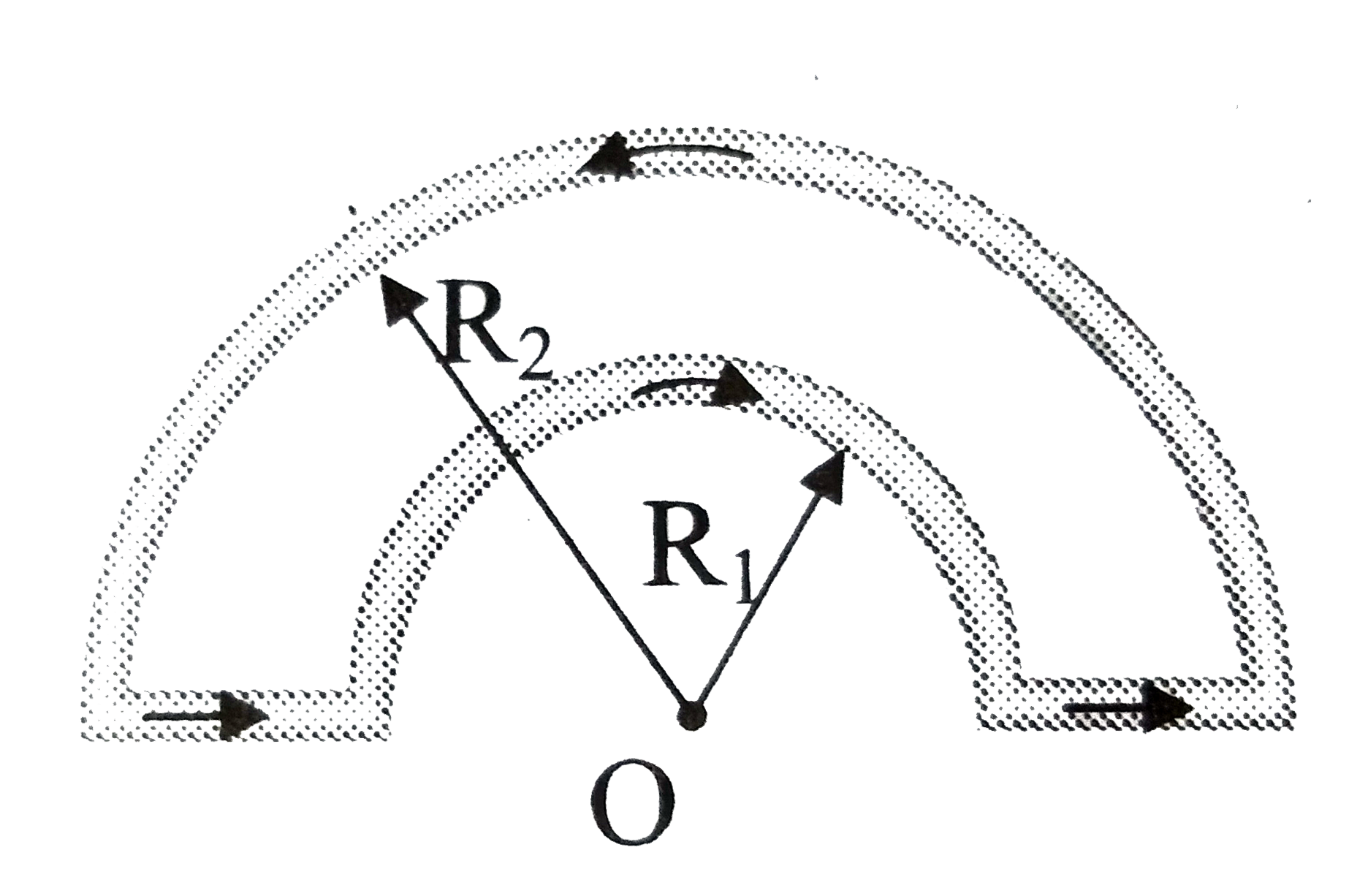
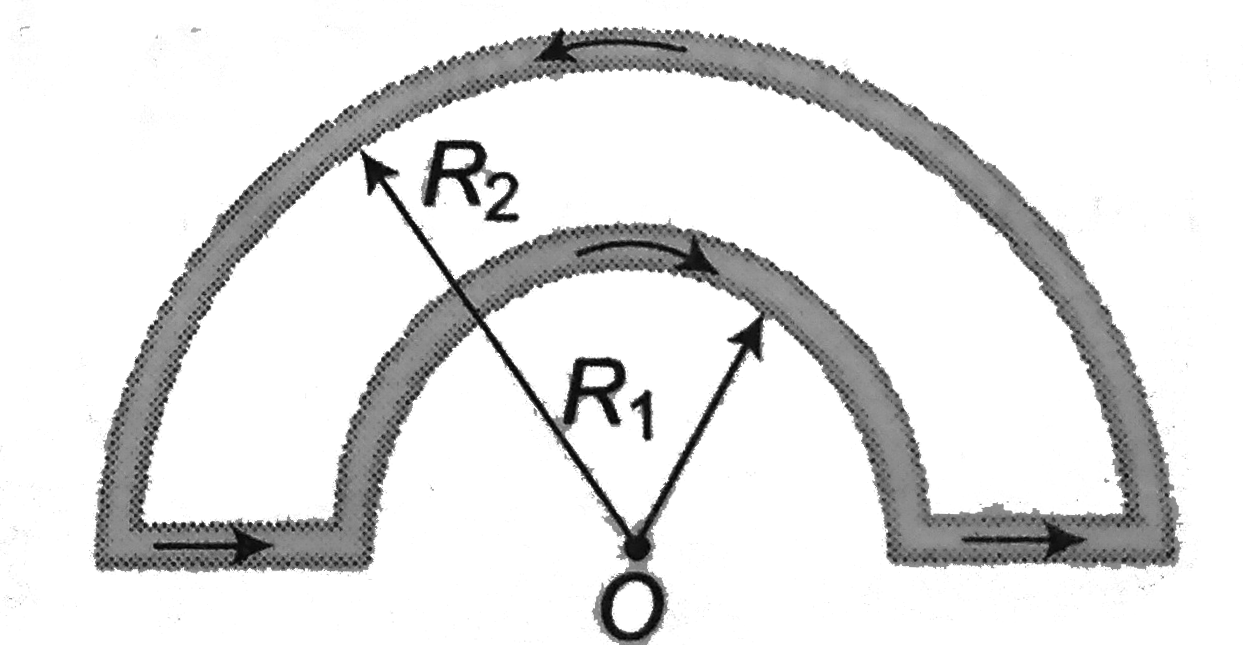
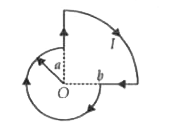
The magnetic induction at centre O Fig.
μ0I2a+μ0I2b⊗
3μ0I8a+μ0I8b⊗
3μ0I8a−μ0I8b⊗
3μ0I8a+μ0I8b⊗
The correct Answer is:B
B=34[μ0I2a]+14[μ0I2b]
B=3μ0I8a+μ0I8b
Topper's Solved these Questions
- SOURCE AND EFFECT OF MAGNETIC FIELDBOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Problems Based On Mixed Concepts
32 Videos
- SOURCE AND EFFECT OF MAGNETIC FIELDBOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Section B - Assertion Reasoning
31 Videos
- SOURCE AND EFFECT OF MAGNETIC FIELDBOOK - A2ZCHAPTER - SOURCE AND EFFECT OF MAGNETIC FIELDEXERCISE - Magnetic Field Due To Current
44 Videos
- SEMICONDUCTOR ELECTRONICSBOOK - A2ZCHAPTER - SEMICONDUCTOR ELECTRONICSEXERCISE - EXERCISE
29 Videos
- WAVE OPTICSBOOK - A2ZCHAPTER - WAVE OPTICSEXERCISE - Section D - Chapter End Test
29 Videos
Similar Questions
Knowledge Check
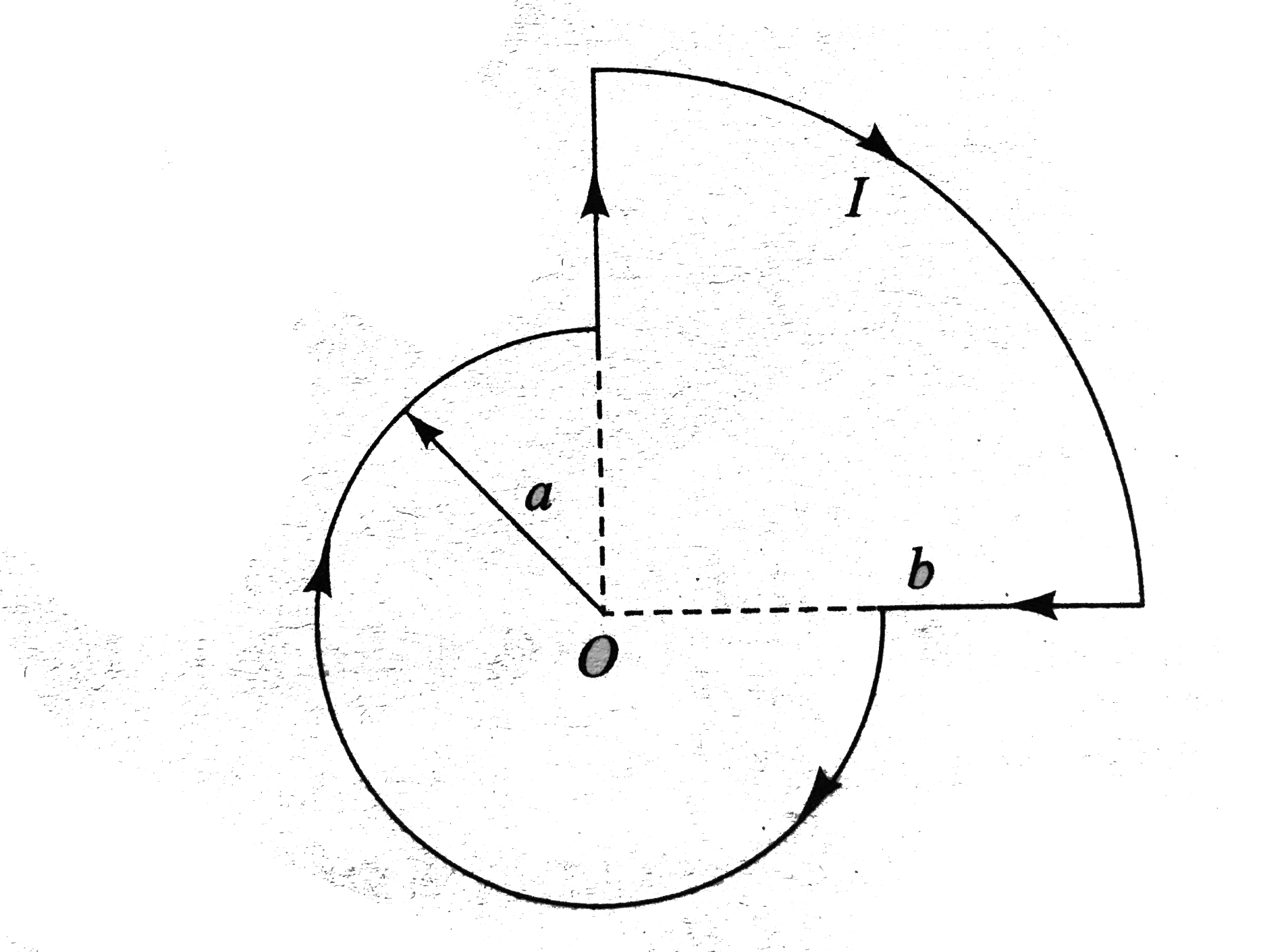
The magnetic induction at centre O in the following figure will be
 Aμ0iα4π(1r1−1r2)⊙Bμ0iα4π(1r1+1r2)⊙Cμ0iα2π[1r1−1r2]⊗Dμ0iα2π[1r1+1r2]⊗
Aμ0iα4π(1r1−1r2)⊙Bμ0iα4π(1r1+1r2)⊙Cμ0iα2π[1r1−1r2]⊗Dμ0iα2π[1r1+1r2]⊗The magnetic induction at the centre O in the figure shown in
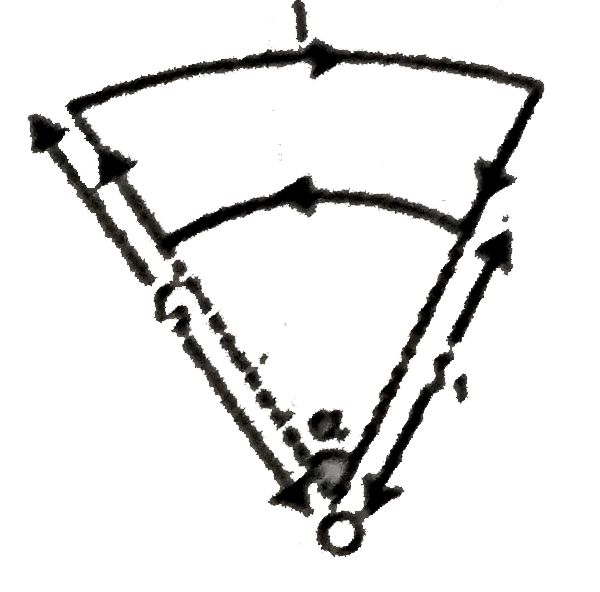
 Aμ0i4(1R1−1R2)Bμ0i4(R1+R2)Cμ0i4(R1+R2)Dμ0i4(R1+R2)
Aμ0i4(1R1−1R2)Bμ0i4(R1+R2)Cμ0i4(R1+R2)Dμ0i4(R1+R2)In the figure shown there are two semicircles of radii r1 and r2 in which a current i is flowing. The magnetic induction at the centre O will be
 Aμ0ir(r1+r2)Bμ0i4(r1−r2)Cμ0i4(r1+r2r1r2)Dμ0i4(r1−r2r1r2)
Aμ0ir(r1+r2)Bμ0i4(r1−r2)Cμ0i4(r1+r2r1r2)Dμ0i4(r1−r2r1r2)
A circular arc of wire of radius of curvature r subtends an angle of p/4 radian at its centre. If i current is flowing in it then the magnetic induction at its centre is -
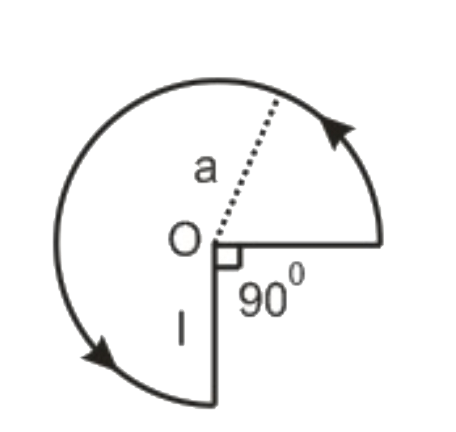
View SolutionThe magnetic induction at center O as shown in the figure is
View SolutionA long wire carrying a steady current is bent into a single coil such that magnetic induction at centre is B. Then same wire is bent to form a coil of smaller radius of 4 turns when magnetic induction at centre is B'. Then
View SolutionThe figure shows a current- carrying loop, some part of which is circular and some part is a line segement. The magnetic induction at the centre is
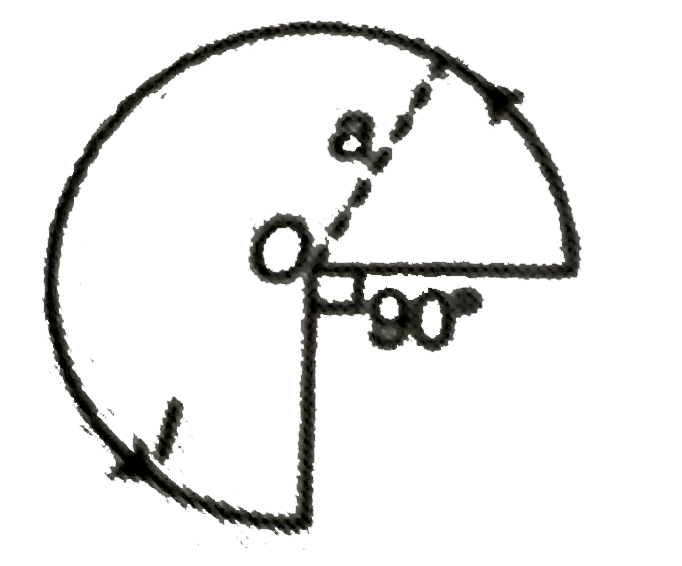
View SolutionFor the arrangment as shown in the figure, the magnetic induction at the centre is
View Solution