The mass M shown in the figure oscillates in simple harmnonic motion and amplitude A. The amplitude of the point P is
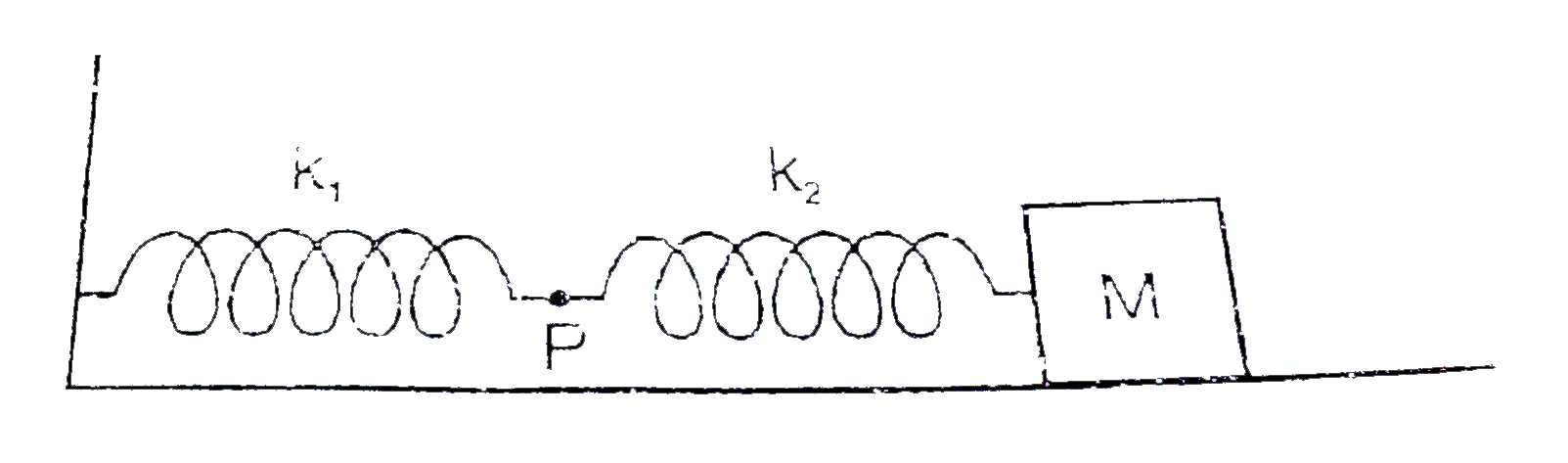
K1AK2
K2AK1
K1AK1+K2
K2AK1+K2
The correct Answer is:D
Let x1 and x2 are maximum elongations produced in the springs when block is at its exteme position then
x1+x2=A …(i)
Here value of x1 is amplitude for point P.
both the springs are connected in series and hence same forces is exerted at the ends of springs.
k1x1=k2x2
⇒x2=k1k2x1
Substituting in equation (i) we get
x1+(k)(1))k2x1=A
⇒x1=k2Ak1+k2
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (Multiple choice question)11videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition File (Multiple choice question) based on a given passage/comprehension9videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Competition file (Multiple choice question) JEE (Main) & Other State Boards for Engineering Entrance25videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - CHAPTER PRACTICE TEST16videos
MOTION IN A STRAIGHT LINE
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
Similar Questions
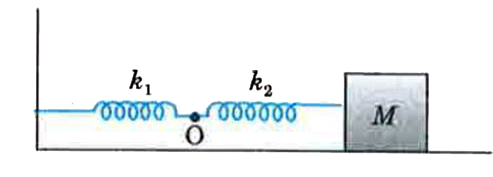
The figure shows a mass M attached to a series arrangement of two springs of spring constants k1andk2 where k2=2k1. If the mass M oscillates in simple harmonic motion with amplitude A, the amplitude of the point O is αAβ. Find β−α.
 View Solution
View SolutionA particle executes simple harmonic motion of amplitude A. At what points is its speed half the maximum speed ?
View Solution
Knowledge Check
- Question 1 - Select One
The mass M shown in figure ocillates in simple harmonic motion with amplitude A.
The amplitude of the point P is
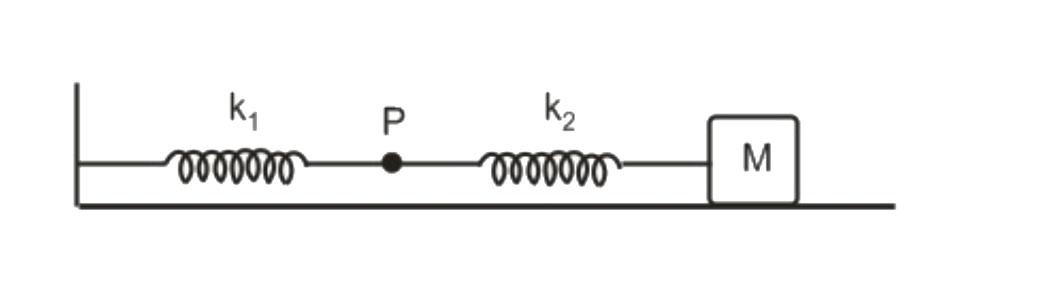 Ak1Ak2Bk2Ak2−k1Ck2Ak1+k2Dk2Ak1
Ak1Ak2Bk2Ak2−k1Ck2Ak1+k2Dk2Ak1 - Question 2 - Select One
The mass M shown in the figure oscillates in simple harmonic motion with amplitude A. The maximum speed of junction P is V0. Assume springs are massless. Select the correct option/s
 AAmplitude of potential energy is springs S1 and S2 is respectivley 19kA2 and kA2BAmplitude of potential energy is S1 and S2 is respectively 29kA2 and 19kA2CV0=A√8k27MDV0=A√8k81M
AAmplitude of potential energy is springs S1 and S2 is respectivley 19kA2 and kA2BAmplitude of potential energy is S1 and S2 is respectively 29kA2 and 19kA2CV0=A√8k27MDV0=A√8k81M - Question 3 - Select One
The total work done by the restoring force in simple harmonic motion of amplitude A and angular velocity ω in one oscillation is
A12mω2A2BmA2ω2CZeroD12mAω
A simple harmonic motion is represented by F(t)=10sin(20t+0.5). The amplitude of the S.H.M. is
View SolutionThe maximum velocity a particle, executing simple harmonic motion with an amplitude 7 mm, 4.4 m//s. The period of oscillation is.
View SolutionA mass m oscillates with simple harmonic motion with frequency f=ω2π and amplitude A on a spring of stiffness constant K. Which of the following is not correct ?
View SolutionThe total work done by a restoring force in simple harmonic motion of amplitude A and angular velocity. ω, in one oscillation is
View SolutionA mass M is attached to a horizontal spring of force constant k fixed one side to a rigid support as shown in figure. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass M is in equilibrium position, another mass m is gently placed on it. When will be the new amplitude of ocillation?
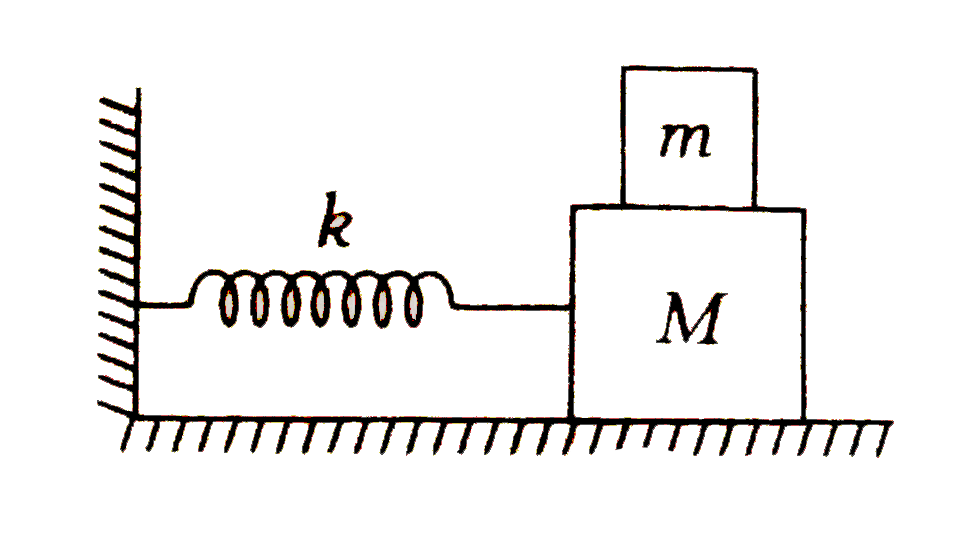 View Solution
View Solution

