The mean kinetic energy of monoatomic gas molecules under standard conditions is . If the gas is compressed adiabatically 8 times to its initial volume, the mean kinetic energy of gas molecules changes to . The ratio is Solution in Telugu
2
4
6
8
The correct Answer is:B
In adiabatic process, constant
In given conditions,
(say), so
and (gas is monoatom)
So,
So, temperature of gas increases by 4 times.
As mean KE of gas molecule is proportional to its temperature, then KE also increases by 4 times.
Topper's Solved these Questions
Similar Questions
If A represents density, B represents velocity, C represents specific heat capacity and D represents wavelength, then the quantity having the dimensions of product of A, B, C and D is
View SolutionMatch the entries in List I with those in List II.

View SolutionEach molecule of nitrogen gas heated in a vessel to a temperature of 5000K has an average energy E1. Some molecule of the gas escape into atmosphere at 300K. Due to collision with air molecules, average kinetic energy of the nitrogen molecule changes to E2. Find the radio E1/E2.
View SolutionTwo bodies of masses m and 2m have same momentum. Their respective kinetic energies E_(1 ) and E_(2) are in the ratio
View SolutionA gas consisting of a rigid diatomic molecules was initially under standard condition.Then,gas was compressed adiabatically to one fifth of its intitial volume What will be the mean kinetic energy of a rotating molecule in the final state?
View SolutionIn SHM , potential energy of a particle at mean position is E_(1) and kinetic enregy is E_(2) , then
View SolutionAt 27^(@)C temperature, the kinetic energy of an ideal gas is E_(1^.) If the temperature is increassed to 327^(@)C, then the kinetic energy will be
View SolutionA molecule may be represented by three structures
having energies E1,E2 and E3 , respectively . The
energies of theses structures follow the order E3<E2<E1 ,
respectively . If the experimental bond energy
of the molecule is E0 , their resonance energy is
View SolutionThe average kinetic energy of the molecules in a gas at T_(1)K and T_(2)K are E_(1) are E_(2)kJ , respectively. If E_(2) gt E_(1) , then which one between T_(1) and T_(2) is higher?
View Solutionकिसी गैस के अणुओं की माध्य गतिज ऊर्जा होती है -
View SolutionThe temperature at which the mean kinetic energy of the molecules of gas is one-third of the mean kinetic energy of its molecules at 180∘C is
View SolutionIf Ek is the average kinetic energy per mole of a gas, then :
View SolutionThe total kinetic energy of a sample of gas which contians N molecules at -123^(@)C has E_(K) joules. Another sample of gas at 27^(@)C has totla kinetic energy of 2 E_(K) joules. The number of molecules in the second sample of gas is :
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
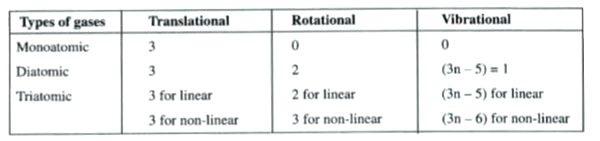
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are E_(K_(x)), E_(K_(y)), E_(K_(z)) Total K.e =E_(K_(x)) + E_(K_(y)) + E_(K_(z)) Since the motion of molecule is equally probable in all the three directions, therefore E_(K_(x)) = E_(K_(y)) = E_(K_(z)) =1/3 E_(K) =1/3 xx 3/2 kT = 1/2 kT , where k =R/N_(A) = Botzman constant. K.E. = 1/2 kT per molecule or =1/2 RT per mole. In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom. Vibration energy =2 xx 1/2kT =2 xx 1/2RT [ therefore two degrees of freedom per mole] If the gas molecules have n_(1) translational degrees of freedom, n_2 rotational degrees of freedom and n_(3) vibrational degrees of freedom, that total energy = n_(1)[(kT)/2] + n_(2) [(kT)/2] + n_(3) [(kT)/2] xx 2 Where 'n' is atomicity of gas. The rotational kinetic energy of H20 molecule is equal to
View Solution
