The optical properties of a medium are governed by the relative permitivity (εr) and relative permeability (μr). The refractive index is defined as √μrεr=n. For ordinary material εr>0 and μr>0 and the positive sign is taken for the square root. In 1964, a Russian scientist V. Veselago postulated the existence of material with εr<0andμr<0. Since then such 'metamaterials' have been produced in the laboratories and their optical properties studied. For such materials n=−√μrεr. As light enters a medium of such refractive index the phases travel away from the direction of propagation.
(i) According to the description above show that if rays of light enter such a medium from air (refractive index = 1) at an angle θ in 2nd quadrant, then the refracted beam is in the 3rd quadrant.
(ii) Prove that Snell's law holds for such a medium.
Suppose the given postulate is true.
In that event, two parallel rays entering such a medium from air (refractive index =1) at an angle θi in 2nd quadrant, will be refracted in 3rd quadrant as shwon in Fig.

Let AB represent the incident wavefront and DE represent the refracted wavefront. All points on a wavefront must be in same phase and in turn, must have the same optical path length.
∴−√∈rμrAE=BC−√∈rμrCDorBC=√∈rμr(CD−AE)
If BC>0, then CD>AE, which is obvious from Fig.
Hence the postulate is resonable.
However, if the light proceeded in the sense it does for ordinary material, (going from 2nd quadrant to 4th quadrant) as shown in Fig. then proceeding as above,
−√∈rμrAE=BC−√∈rμrCDorBC=√∈rμr(CD−AE)
As AE>CD, therfore, BC<0 which is not possible. Hence the given postulate is correct.
(ii) In Fig. BC=ACsinθiandCD−AE=ACsinθr
As BC=√∈rμr(CD−AE)
∴ACsinθi=√∈rμrACsinθr
or sinθisinθr=√∈rμr=n, which proves Snell's law.
Topper's Solved these Questions
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Higher order thinking skills
3 Videos
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Value based questions
3 Videos
- OPTICSBOOK - PRADEEPCHAPTER - OPTICSEXERCISE - NCERT Exercise (10 chapter)
3 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)
2 Videos
Similar Questions
The optical properties of a medium are governed by the relative permittivity (εr) and relative permeability (μr). The refractive index is defined as √μrεr=n. For ordinary material, εr>0 and μr>0 and the positive sign is taken for the squre root.
In 1964, a Russian scientist V. Veselago postualted the existance of material with εr<0 and μr<0 . Since, then such metamaterial have been produced in the laboratories and their optical properties studied. For such materials n=−√μrεr. As light enters a medium of such refractive index the phases travel away from the direction of propagation.
(i) According to the description above show that if rays of light enter such a medium from air ( refractive index =1) at an angle theta in 2nd quadrant, then the refracted beam is in the 3rd quadrant.
(ii) Prove that Snell's law holds for such a medium.
View SolutionThe optical properties of a medium are governed by the relative permitivity (εr) and relative permeability (μr). The refractive index is defined as √μrεr=n. For ordinary material εr>0 and μr>0 and the positive sign is taken for the square root. In 1964, a Russian scientist V. Veselago postulated the existence of material with εr<0andμr<0. Since then such 'metamaterials' have been produced in the laboratories and their optical properties studied. For such materials n=−√μrεr. As light enters a medium of such refractive index the phases travel away from the direction of propagation.
(i) According to the description above show that if rays of light enter such a medium from air (refractive index = 1) at an angle θ in 2nd quadrant, then the refracted beam is in the 3rd quadrant.
(ii) Prove that Snell's law holds for such a medium.
View Solution
Knowledge Check
The optical properties of a medium are governed by the relative permitivity (εr) and relative permeability (μr). The refractive index is defined as √μ1εr=n . For ordinary sing is taken for the square root. In 1964, a Russian scientist V. Veselago postulated the existence of material with εr<0 and μr<0 . Since then such 'meta materials' have been produced in the laboratorised and their optical properties studied. For such materials n=−√μrεr. As light entes a medium of such refractive index the phases traval away front the direction ofpropagation.
For light incident from air on the meta materials, then appropriate ray diagram isA B
B C
C D
D
The optical properties of a medium are governed by the relative permitivity (εr) and relative permeability (μr). The refractive index is defined as √μ1εr=n . For ordinary sing is taken for the square root. In 1964, a Russian scientist V. Veselago postulated the existence of material with εr<0 and μr<0 . Since then such 'meta materials' have been produced in the laboratorised and their optical properties studied. For such materials n=−√μrεr. As light entes a medium of such refractive index the phases traval away front the direction ofpropagation.
Consider following statements
i) Snall's slaw valid for these mater ials
ii) Snell's slaw is not valid for these mater ials
iii) Speed of light in these mater ial is v=c|n|
iv) Speed of light in these material is v=cAI, iii are correctBI, iv are correctCii, iv are correctDii, iii are correctThe relative permeability (μr) of a substance is related to its susceptibility (χ) as
Aμr=I−χBμr=I+χCμr=I−χ2Dμr=I+χ2
Write the formula for the velocity of light in
a material medium of relative permitivity ∈r and relative magnetic permeability μr.
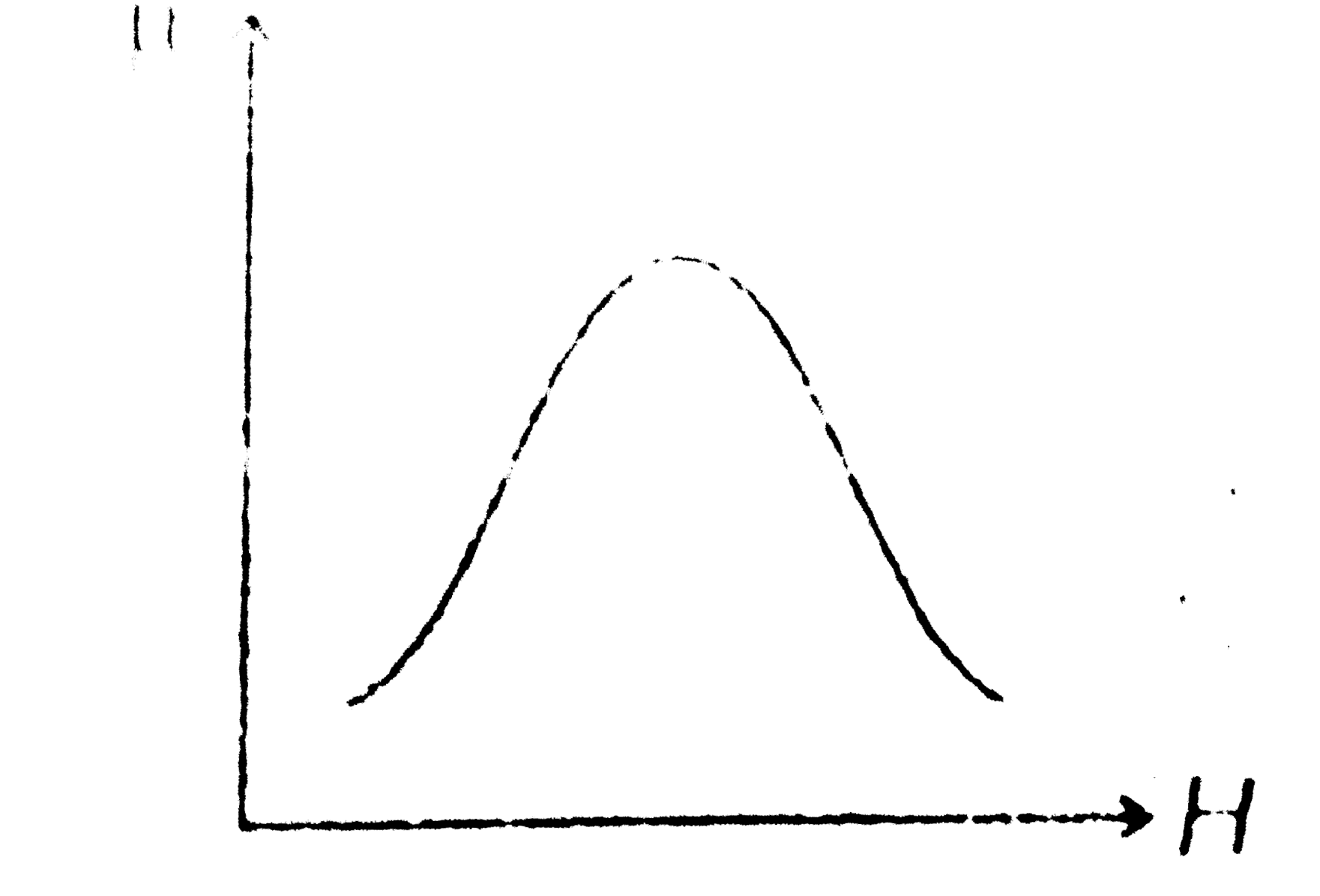
View SolutionVariation of μr (relative permeability) with magnetizing field as shown in figure is for
View SolutionWhich of the following is not correct about relative magnetic permeability (μr)?
View SolutionFor ferromagnetic material, the relative permeability (mu_(r)), versus magnetic intensity (H) has the following shape
View SolutionThe dimensional formula μ0ε0 is
View Solution
