The power of a biconvex lens is 10 dioptre and the radius of curvature of each surface is 10 cm. Then the refractive index of the material of the lens is
The correct Answer is:A
Power of lens, P (in diopter)
According to lens maker.s formula
For biconvex lens,
Topper's Solved these Questions
- BOOK - MTG GUIDECHAPTER - OPTICSEXERCISE - Check your NEET vitals25videos
OPTICS
- BOOK - MTG GUIDECHAPTER - OPTICSEXERCISE - AIPMT/NEET55videos
OPTICS
- BOOK - MTG GUIDECHAPTER - OPTICSEXERCISE - AIPMT/NEET55videos
OPTICS
- BOOK - MTG GUIDECHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - AIPMT/NEET (MCQs)48videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
Similar Questions
The focal length of an equiconvex lens is equal to radius of curvature of either surface. What is the refractive index of the material of the prism ?
View SolutionA biconvex lens has a focal length half the radius of curvature of either surface. What is the refractive index of lens material ?
View Solution
Knowledge Check
- Question 1 - Select One
The power of a biconvex lens is 10 dioptre and the radius of curvature of each surface is 10 cm. Then the refractive index of the material of the lens is
A3/2B4/3C9/8D5/3 - Question 2 - Select One
Power of biconvex lens is 10 diopters and radius of curvature of each surface is 10cm.Then refractive index of material of lens is
A43924B44082C43954D43892 - Question 3 - Select One
For a plano convex lens, the radius of curvature of convex surface is 10 cm and the focal length is 30 cm. The refractive index of the material of the lens is
A1.5B1.66C1.33D2.5
The focal length of an equiconcave lens is 34 times of radius of curvature of its surfaces. find the refractive index of the material of lens. under what condition will this lens behave as a converging lens?
View SolutionA converging bundle of rays is intercepted by a biconcave lens. The radii of curvature of both surfaces are 20cm and the refractive index of the material of the lens is 1.5. If the rays originially converged to a point 10cm in front of the lens, where will they now converge after passing through the lens?
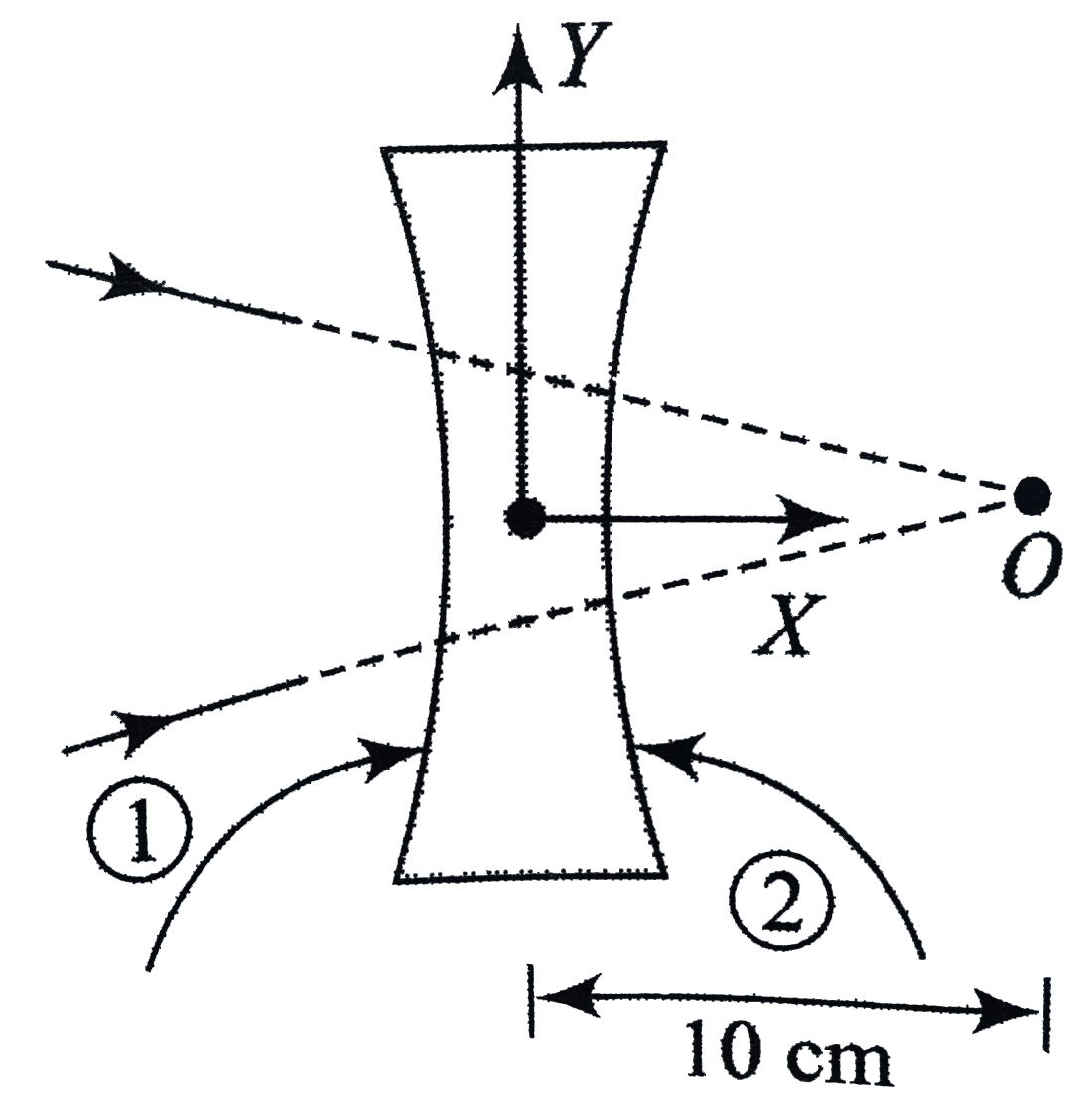 View Solution
View SolutionIf in a planoconvex lens, the radius of curvature of the convex surface is 10cm and the focal length is 30cm, the refractive index of the material of the lens will be
View SolutionThe radius of curvature of each surface of a lens is 40 cm. Its refractive index is 1.5. The focal length of lens will be
View SolutionThe focal length of a double convex lens is equal to radius of curvature of either surface. The refractive index of its material is
View Solution

